by: Doris Santarone
Final Assignment #1: Investigating 
Consider the rational equation of the form:
Part 1: I want to explore the effects of changing a, b, c, and d.
VARY a: I am going to set b = 3, c = 1, and d = 1, and I will vary a. I will use a = -2, -1, 1, 4, and 5.
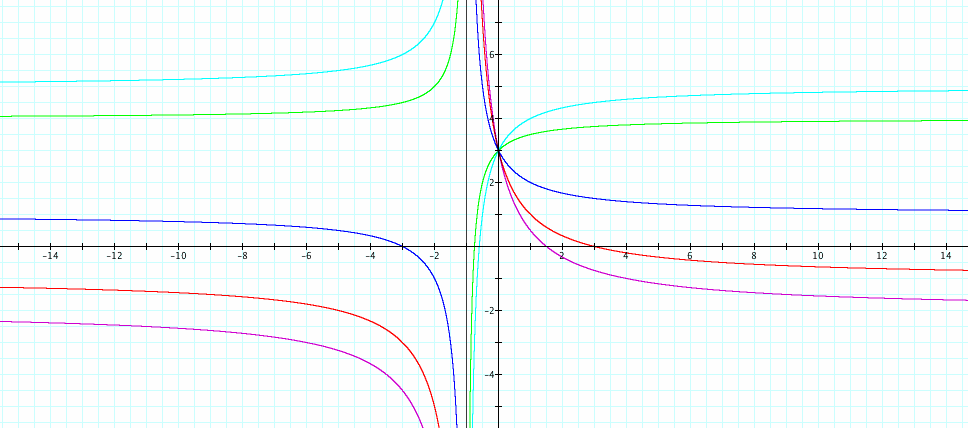
Observations:
1) The vertical asymptote is the same for all functions at x = -1. This shows a restriction of the domain. Since our function is a rational expression, we know the denominator cannot equal 0. So, for this function x + 1 cannot equal zero, or x cannot equal -1. This is where the vertical asymptote is found.
2) The y-intercept is the same for all functions at y=3. This can be found by setting x = 0.
3) All of the function have a horizontal asymptote, but it is NOT the same. The horizontal asymptote for each function is y = a/c.
4) The functions have different x-intercepts. The x-intercept can be found by setting the numerator equal to zero because if the numerator is equal to zero, then the entire fraction is equal to zero (or y = 0).
4) The green and light blue functions are increasing functions, while the purple, red, and dark blue functions are decreasing. When does it change from being a decreasing function to an increasing function? Look at the video below, where a varyies from -10 to 10:
The function changes from a decreasing function to an increasing function at a = 3. When a = 3, the function is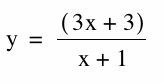 
, which can factor and simplify to y = 3, which is a horizontal line.
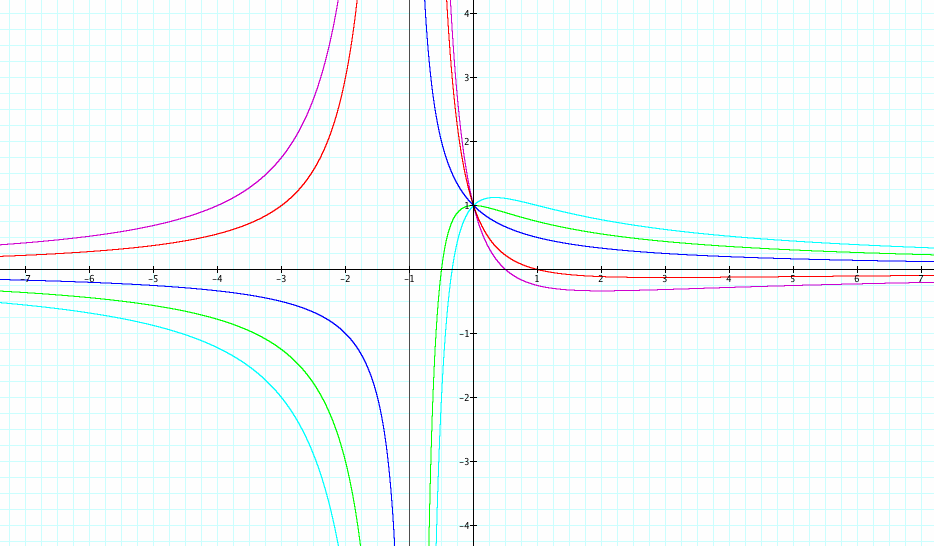
VARY b: I am going to set a = 1, c = 1, and d = 1. I will vary b, letting b = -3, -1, 1, 3, and 5.
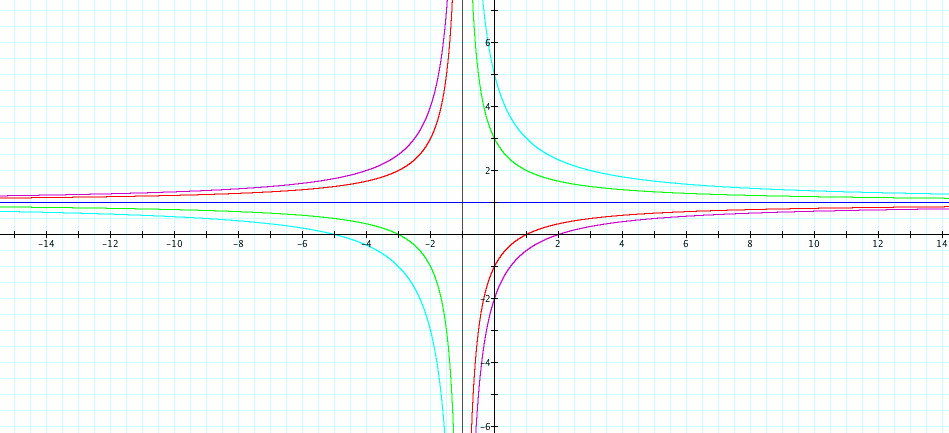
Observations:
1) All of the functions have the same vertical asymptote at x = -1. Again, this is found by the restriction on the denominator.
2) All of the functions have the same horizontal asymptote at y = 1. This is found by y = a/c. In this case, y = 1/1 = 1.
3) The functions have different x-intercepts. As stated before, the x-intercepts are found by setting the numerator equal to zero because if the numerator is equal to zero, then the entire fraction is equal to zero.
4) The functions have different y-intercepts. As stated before, the y-intercepts are found by setting x = 0.
5) The purple and red functions are increasing; the dark blue function is constant, and the green and light blue functions are decreasing. Like before, I can see that the function changes from a decreasing function to an increasing function when the numerator is divisible by the denominator, making it simplify into a constant. I wanted to take this a step further to determine when exactly the function will be increasing and when it will be decreasing. I know that it will be constant when ax+b is divisible by cx+d. This is only true when a/c = b/d, or when ad = bc. From this, we can see that when ad > bc, the function is increasing, and when ad < bc, the function is decreasing.
**At this point, I feel like I know how each variable affects the graph. But, I will continue to vary c and d to verify my findings.
VARY c: I will set a = 2, b = 1, and d = 1. Then, I will vary c, letting c = -2, -1, 1, 3, and 5.
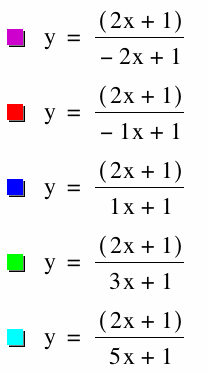
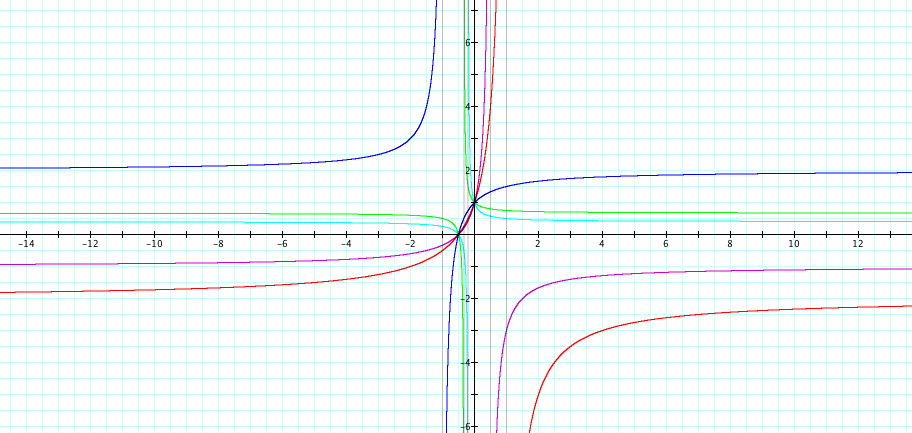
Observations:
1) Vertical asymptote is found by setting the denominator equal to 0.
2) Horizontal asymptote is found by y = a/c.
3) X-intercepts are found by setting the numerator equal to 0. In this case, the numerator is the same for all of the functions, so the x-intercept is -1/2 for all of the functions.
4) Y-intercepts are found by setting x = 0. In this case, the y-intercept is 1 for all functions because when we plug 0 in for x, we get c(0) which will eliminate c from affecting the y-intercept.
5) The purple, red, and dark blue functions are increasing because ad > bc for each of them (purple --> 2(1) > 1(-2), red --> 2(1) > 1(-1), dark blue --> 2(1) > 1(1)). The green and light blue functions are decreasing because ad < bc (green --> 2(1) < 1(3) and light blue --> 2(1) < 1(5)).
VARY d: I will set a = 2, b = 1, and c = 1. Then I will vary d, setting d = -2, -1, 1, 3, and 5.
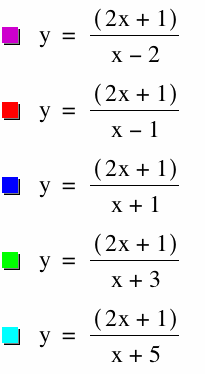
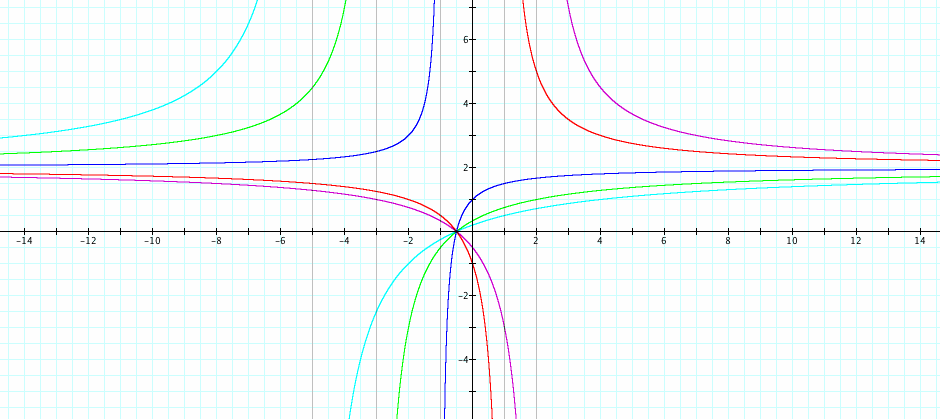
Observations:
1) Vertical asymptote is found by setting the denominator equal to 0.
2) Horizontal asymptote is found by y = a/c.
3) X-intercepts are found by setting the numerator equal to 0. In this case, the numerator is the same for all of the functions, so the x-intercept is -1/2 for all of the functions.
4) Y-intercepts are found by setting x = 0.
5) The purple and red functions are decreasing because ad < bc for each of them (purple --> 2(-2) < 1(1), red --> 2(-1) < 1(1)). The dark blue, green, and light blue functions are increasing because ad > bc (dark blue --> 2(1) > 1(1), green --> 2(3) > 1(1) and light blue --> 2(5) < 1(1)).
CONLUSIONS:
1) Vertical asymptote is found by setting the denominator equal to 0.
2) Horizontal asymptote is found by y = a/c.
3) X-intercepts are found by setting the numerator equal to 0.
4) Y-intercepts are found by setting x = 0.
5) If ad > bc, the function is increasing. If ad < bc, the function is decreasing. If ad = bc, the function is constant.
NOTE: You can also determine whether a function is increasing or decreasing by finding the value of the first derivative. If f'(x) is positive, then the slope of the function is positive, or the function is increasing. If f'(x) is negative, then the slope of the function is negative, or the function is decreasing.
Part 2: Generalize for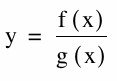 
where f(x) and g(x) are polynomials.
How would the conclusions that I made in Part 1 change if:
1) the degree of f(x) were greater than the degree of g(x)?
2) the degree of f(x) were less than the degree of g(x)?
3) the degree of f(x) were equal to the degree of g(x), but the degrees are greater than 1 (like in Part 1)?
1) the degree of f(x) were greater than the degree of g(x)?
I will set f(x) equal to the quadratic function (degree =2)
and g(x) will be a linear function (degree = 1) and I will vary the linear function.
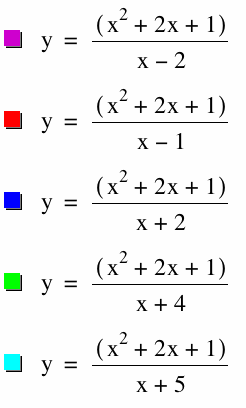
Observations:
1) Vertical asymptote is still found by setting the denominator equal to 0.
2) There is NO horizontal asymptote. This time, there is a slant asymptote. The slant asymptote can be found by dividing f(x)/g(x) and disregarding the remainder.
3) X-intercepts are still found by setting the numerator equal to 0.
4) Y-intercepts are found by setting x = 0.
5) These functions change direction, unlike the previous example. These functions are all increasing, then decreasing to -infinity at the vertical asymptote. After the asymptote, the graph is decreasing, then increasing.
Here are a few more example where the degree of f(x) is greater than the degree of g(x).
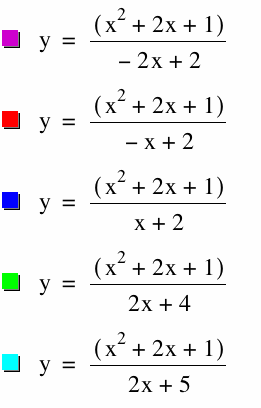
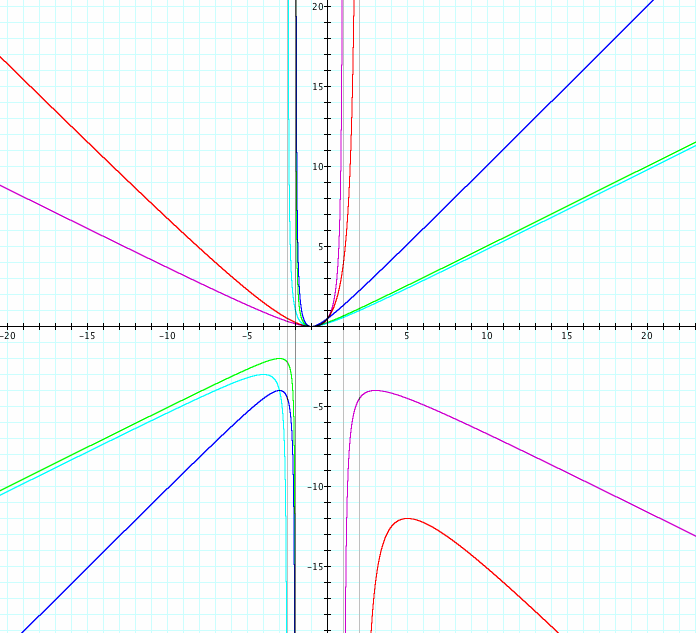
The only change here is that some of the funtions are increasing first (dark blue, green, and light blue) and others are decreasing first (purple and red).
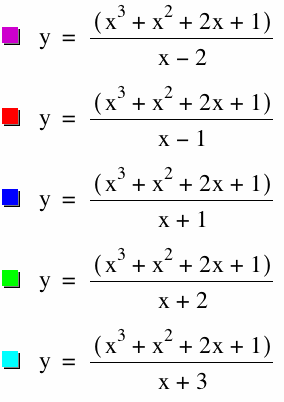
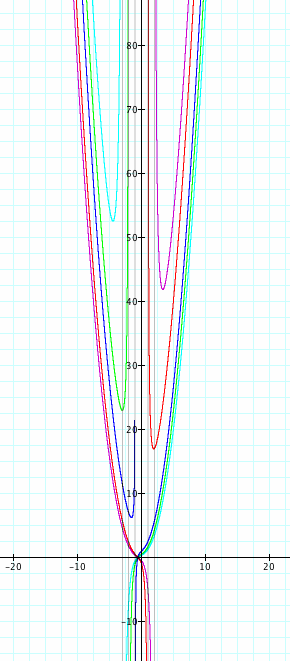
You can see here that the asymptotes are different...they are actually quadratic asymptotes. The reason for this is because when you perform long division, your result will be a division of x^3 by x, which will be an x^2 function.
2) the degree of f(x) were less than the degree of g(x)?
I will set f(x) equal to a linear function and g(x) equal to a quadratic function.
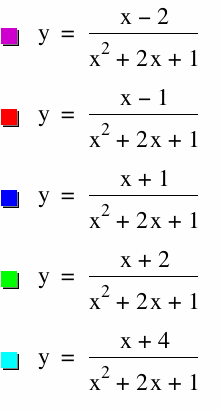
Observations:
1) Vertical asymptote is still found by setting the denominator equal to 0.
2) The horizontal asymptote is y = 0 for all of the functions.
3) X-intercepts are still found by setting the numerator equal to 0.
4) Y-intercepts are found by setting x = 0.
5) The purple and red functions are decreasing to the vertical asymptote, then increasing after the asymptote. The green and light blue functions are increasing to the vertical asymptote, then decreasing after the asymptote. The blue function is always decreasing (this is because the numerator divides into the denominator, simplifying this to a constant over a linear function).
Here are a few more examples:
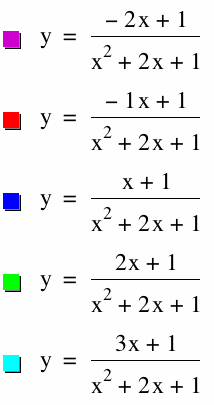
Still have horizontal asymptotes at y = 0!!
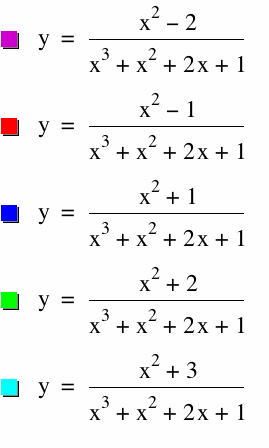
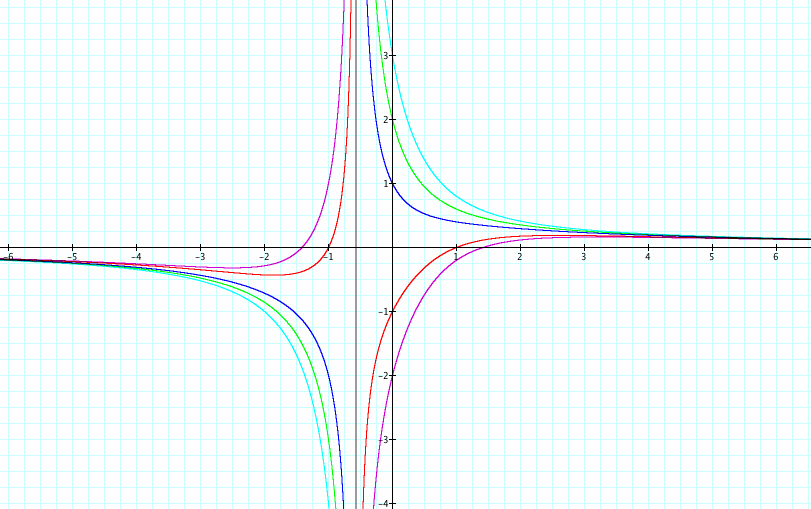
Even when the functions are changed to larger degrees, the horizontal asymptote is still always at y = 0!!!
3) the degree of f(x) were equal to the degree of g(x), but the degrees are greater than 1 (like in Part 1)?
I will make f(x) and g(x) a quadratic, then both of them a cubic, then both a quartic, then both a fifth degree function:
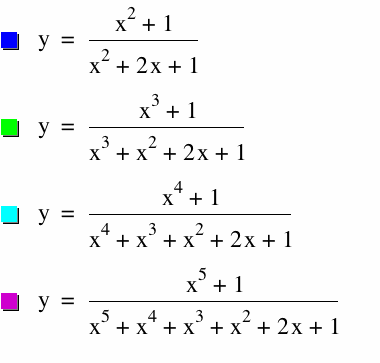
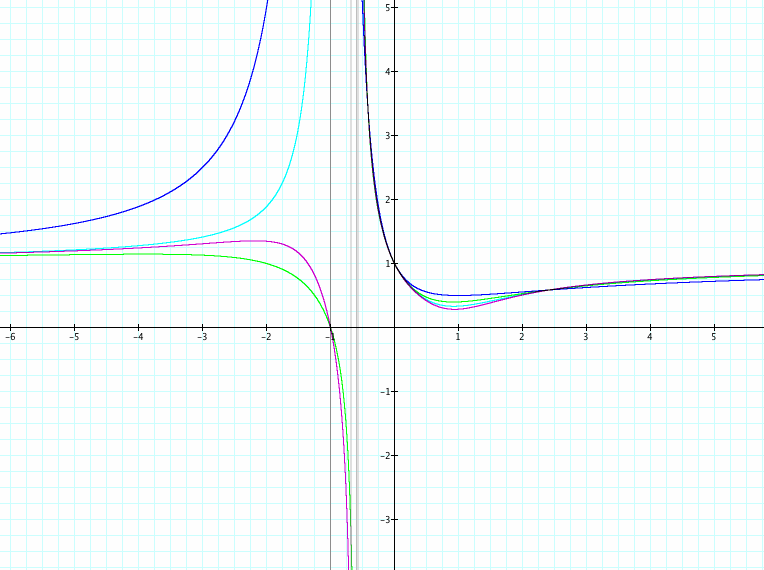
CONCLUSIONS:
1) Vertical asymptote is found by setting the denominator equal to 0.
2) Horizontal asymptote is found by:
If the degree of f(x) is the same as the degree of g(x), then the horizontal asymptote is y = a/c.
If the degree of f(x) is larger than the degree of g(x), then there is no horizontal asymptote. There is a slant asymptote (or even a curved asymptote). This can be found by calculating long division
If the degree of f(x) is smaller than the degree of g(x), then the horzontal asymptote is y = 0.
3) X-intercepts are found by setting the numerator equal to 0.
4) Y-intercepts are found by setting x = 0.
5) You can determine whether a function is increasing or decreasing at a specific x on the curve by finding the value of the first derivative. If f'(x) is positive, then the slope of the function is positive, or the function is increasing. If f'(x) is negative, then the slope of the function is negative, or the function is decreasing.