
This is clearly an investigation on parametric equations and how they can save the day
By
Ryan Shannon
Let's start with a very basic parametric equation and it's graph:
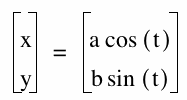
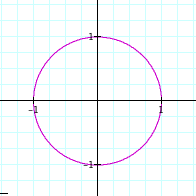
Here we can notice a few things, starting with a radius of one. This is a perfect circle, so perfect we call it the unit circle. This is what some teachers would tend to say is the bases of trigonometry. This circle is the basic, lets continue to explore more by changing B and A.
Let's try and see what happens as we change A,and B from -3,3 where A = B and 0< t < 2pi
Clicking the link above we can see that if A, and B are equal and either positive or negative value, the radius of the circle is going to coordinate with that value.
Here is a great way to show students that the values of sin and cos can be defined as
![]()
by using the values of the intercepts and squaring them we get one ever time.
I'm beginning to think that A and B could be the values that coincide with the X-axis and Y-axis respectively.
n cos which is represented by the red graph, n sin which is represented by the blue graph.
When clicking the link above we can notice that the leading coefficient does correlate with the radius of that axis.
We can conclude that the radius will correspond to the
. Now we also notice from the graphic above that when a>b that we will be stretched more on the y-axis and too that b>a we will be stretched more on the x-axis. Can we start to conclude that cosine correlates to the x-axis and sine correlates to the y-axis?
Let's go further and investigate the square of the parametric equations
What a change, we now are changed into a negative sloping line, that is only contained in the first quadrant. This could be easily seen as when we square a value we are going to only be positive, thus we can exclude the values in the negative x, y-axis, unless maybe both values were negative. As shown above. Let us go further into changing out coffins. I would image that our guess would be that we could have a very similar relationship with the x-axis and cosine and the y-axis and sine.
First we investigate when a=b, and a,b > 0 or a,b<0
Here we notice that as n increases so does the graph in the positive direction along the x,y-axis respectively. The values of n are a positive correlation with the intercepts of the axis increases. The same is true as both a and b are negative. The line is unchanged but in the third quadrant and the distance from zero increases as the value for a,b increases.
What if we allow only a to change, Where b will stay constant, or if we allow a to be constant and b to change?
purple represents n sin graph and red represents n cos graph
We notice that the when the lines move we have a stationary point of one. This is the domain of the sin which is [-infinity,infinity) is the range of the function cos. The domain of cos is [0,1] which is the range of sin.
As we watch n change as related to cos, we notice that the values are only changing on the x-axis. Thus we can start to strengthen our assumption that cosine is connected to the x-axis. This too we notice that sine is changing along the y-axis and connected to that axis. Are these the points at which the circles and ellipses from above had a radius?
When looking at the graph we notice that sin and cos do not cross each, nor do they enter the third quadrant.
since cos is squared it's value will always remain positive and thus always stay in positive x-values.
Are the circles from the first equations
going to meet the x,y-axis as the lines in the second equation
If you click the photo below you can see how all the graphs listed above will interact with one another.
The yellow graph is the first equations
The light blue and light green graphs are the second equations
the blue, green and red lines are the last equations.
When looking at the above interactive graph please notice how each react.
Note how the square of sin and the square of cos line are constantly touching the ellipses that are created from sin and cos functions.
Also that there are times when the two circles are equal, the that square lines over lap and when the circles have points of intersection. These points are the special angles or radian measure that are taught in pre calc.