

Problem:
A parabola is the set of points equidistant from a line, called the directrix, and a fixed point,called the focus. Assume the focus is not on the line. Construct a parabola given a fixed point for the focus and a line (segment) for the directrix.
Write up:
The parabola is something that is given a lot of time in the High School Curriculum as a consequence of quadratic functions and is basically treated as the "shape" that results when plotting points of a quadratic function. However, not many students (at least I didn't) learn the true definition of what a parabola is. In fact, I am confident that if you asked 100 school children to define a parabola precisely, most would not come close to the definition above. This write up is a short introduction to how GSP can help one understand what a parabola truly is.
First: Use an Action Button to generate the parabola from an animation and trace of a constructed point.
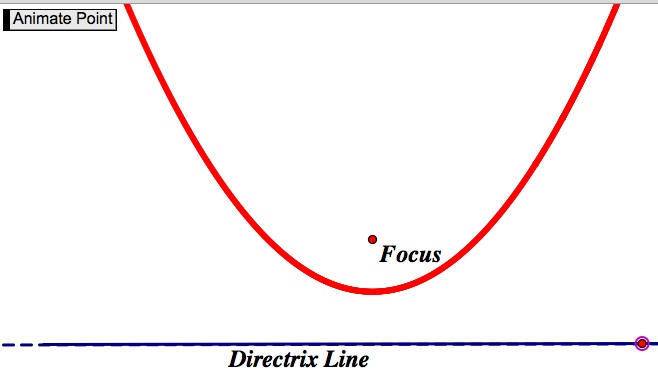
See the GSP file attached here to animate. The below image shows what the trace will look like after it is animated. Just seeing the focal point and the directrix line would give children a good hands on feel to what the parabola is actually representing.
Second: Repeat with a trace of the tangent line at the constructed point.
See the GSP File attached here to animate. The below image shows what the trace of the tangent line will look like after it is animated. Once again all you will have to do is click animate point. You will see the tangent line before you start the animation.
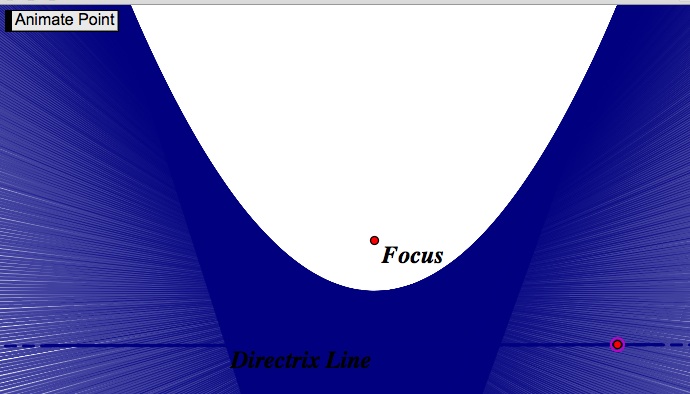
You can clearly see the envelope of lines that is traced out by the tangent line of the parabola at each given point. As would be expected, the envelope traces out the parabola, leaving the inside clear.
Third: Use the locus command to generate the parabola from a constructed point or the tangent line at that point.
This can actually be done using the GSP file linked in the second part, but I will link it again here. To do this do the following:
1. Click on the tangent line
2. Click on "construct" in the menu bar.
3. Click locus, which should be the very last thing in the drop down box.
4. The result should be something like this:
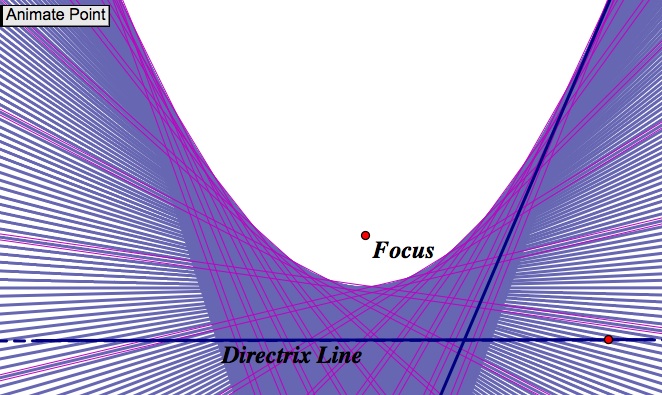
Once again, we see here an envelope of lines "spread out" by the tangent lines to the parabola, the intersections all equidistant from both the focus and the directrix line.
Take aways from this type of exploration:
1. One can more easily see dynamic concepts like the tracing of the tangent line on a program like this than on regular pen and paper. Imagine just trying to draw all of those lines?
2. Like I said at the outset, I wonder if many students know what a "focus" actually is in terms of a parabola, and I am almost certain most do not know what a directrix line is.
3. Finally, it brings out the concept of a locus, which can be immediately done using an easy command in the GSP menu, but can very easily lead to explaining to students what a locus means.