

Throughout this write-up, we will be looking at the roots of quadratics in alternative planes, namely the X-B and X-C planes. We will be working with the standard form of quadratics.
X-B plane
We will work with the standard equation with a=1. So, we are looking at
. We are examining the roots, the solutions, so that means we need to let y=0.
For this first part, I will focus on the roots with c=1. So we have
which becomes
when we let c=1. We can use a graphing utility such as Graphing Calculator to get a better understanding of what this looks like and also what it means in relation to the roots of our quadratic.
Remember that this is the X-B plane. So the x-axis is the horizontal axis as usual. However, the vertical axis is not the B-axis.
The solutions to our equation, or our roots, can be represented by intersections of a horizontal line with our graph. The number of solutions is exactly the number of times that the horizontal line crosses the graph. Realize that horizontal lines are values of B. So obviously as B changes, the solutions themselves as well as the number of solutions change. You can see this and explore it for yourself below before I discuss it.
From the graph, we can see that there are 2 solutions when |B|>2. There is 1 solution when |B|=2. And there is 0 solutions when |B|<2. Again, I determined this by looking at when and how many times our horizontal line crosses our curve.
We can also make sense of this algebraically. The ever-popular quadratic formula can also give us this information. All we need to focus on from the quadratic formula is the discriminant, that is
. With a=1 and c=1, this can be simplified to
. Now we can use this simplified form to find the number of solutions. We know that when the discriminant is positive, there are 2 real solutions. When the discriminant is negative there are no real solutions. And when it is 0, there is 1 real solution. Here we are only concerned with real solutions. That is, complex solutions are not being considered here.
First, we can easily find when
. The solutions to this equation here are b=2 and b=-2. This corresponds to what we learned from our graph. The horizontal line only crosses our graph once at b= 2 and -2.
From there we can logically gather the remaining solutions. Or we could set up two inequalities and solve those to get our number of solutions. So, there would be 2 solutions when
and 0 solutions when
. Solving these inequalities results in the same analysis as the graph
Now I will consider the roots when c=-1. So our new function is
. The graph is below on the left. Now, if we do the same thing as above but this time imagining the horizontal line, we can see that this equation always has 2 solutions since any horizontal line would cross the graph twice.
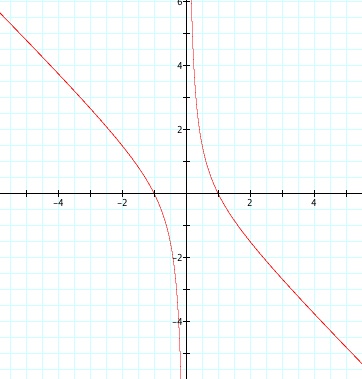
Again, this makes sense algebraically by looking at the discriminant. With a=1 and c=-1 this time, the discriminant becomes
. Since the square of any number is positive, adding 4 to it will keep it positive. Therefore, since the discriminant is always positive, there is always 2 real solutions.
I only examined the equations with c=1 and c=-1. However, the case is similar to c=1 for and c>0 and similar to c=-1 for any c<0. By similar I mean that the curves look similar. There will always be 2 solutions for any equation with c<0. And there will always be 0, 1, and 2 solutions for an equation with c>0. When c=1, we have a different scenario. Now instead of having curves, we have a line. There is an animation below that might make more sense of all of this.
X-C Plane
Now we can look at the X-C plane. We will let a=1 and b=1 to begin with. So, that gives us
to work with. The graph is below.
Again, horizontal lines represent values of c where the equation has a solution and the number of intersections of the horizontal line with the curve is the number of the solutions. So we need to find the vertex to analyze the solutions. But right now, we know that there will be one solution when c is the vertical component of the vertex. There will be zero solutions when c is greater than that value and 2 solutions when c is less than that value.
The vertex is (-.5, .25). Therefore when c=.25 there is only one solution. When c>.25, there are 0 solutions and when c<.25, there are 2 solutions.
Now, we can let b=-1. So, we have
. The graph of that is below.
If we find the vertex of this parabola, we get (.5, .25). Although the x-coordinate of the vertex changed, the conditions of the solutions are the same because the c-coordinate of the vertex did not change.
We can make sense of this algebraically by again referencing the quadratic formula and the discriminant. The discriminant does not change when b changed from negative to positive. This is because b is being squared. Therefore, the sign of b is relatively insignificant when determining the number of solutions. Now, if we consider the entire quadratic formula, we can make sense of how the vertex changed. I would like to break up the quadratic into two parts. So we have
and
. We already deduced that the right half of the formula does not change with b. However, we see that the left half does. This left half represents the axis of symmetry, which is also the x-coordinate of the vertex. Therefore, the x-coordinate switches signs but that is the only part that changes.