Ratios in a Triangle Using the Orthocenter

Alex Szatkowski

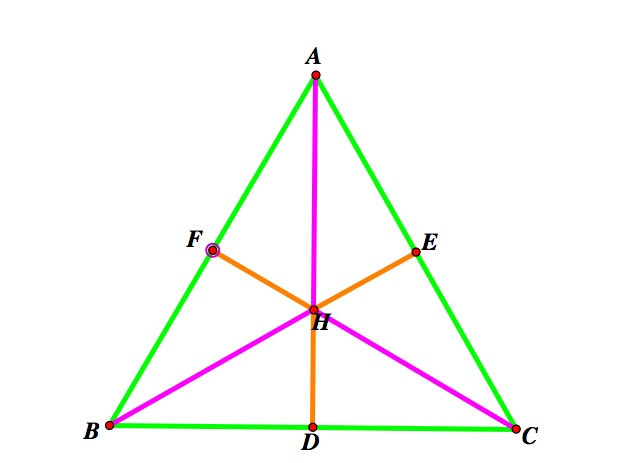
Given acute triangle ABC. Construct the Orthocenter H. Let points D, E, and F be the feet of the perpendiculars from A, B, and C respectfully.

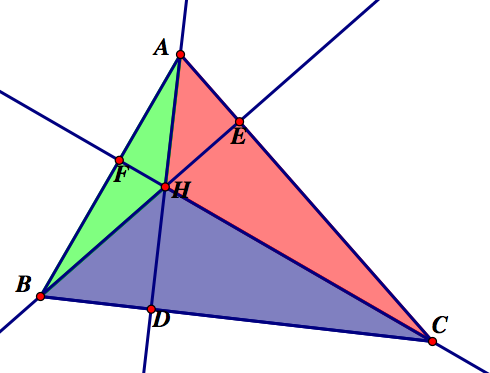
We must prove: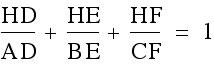

as well as: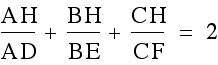

There are several different ways to prove these ratios, but lets take a look at the areas of these triangles.

In order to look at areas, I have divided up our original triangle into three smaller ones. Triangle BAH, triangle AHC and triangle BHC.

Therefore:

Dividing through by ABC: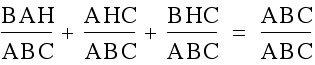

and furthermore: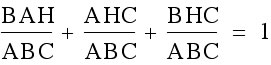

The area of a triangle can be found using the formula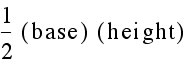

So, the area of triangle BAH is 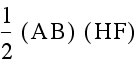in addition, (AB) (HF) = 2 times the area of triangle BAH.

So:

1) 2 times the Area of BAH = (AB) (HF)

2) 2 times the Area of AHC = (AC) (HE)

3) 2 times the Area of BHC = (BC) (HD)

4) 2 times theAarea of ABC = (BC) (AD) (which we will call "D")

We also know that (AB) (HF) is one third the area of the total triangle, as well as (AC) (HE) and (BC) (HD)

So, using these two conclusions, we can re-write our equation again.

then,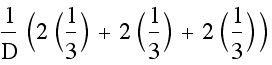  

Furthermore, D is 2 times the Area of ABC, which in relation to the other three triangles in 1, so:

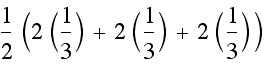
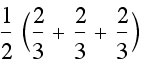
And finally: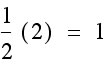 

So: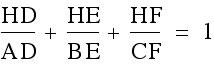

So we have proven our first task, lets take a look at the second.

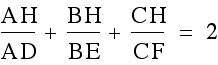
Lets consider: 1. AH= AD - HD 2. FH = FC - HC 3. CH = CF - HF

So: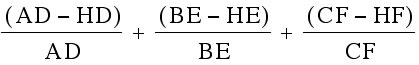

After re-writing each fraction:

and grouping the ratios of a segment to itself: 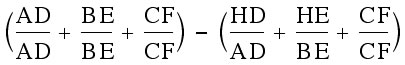I now see two sets of ratios for which I know the value.

We can now see that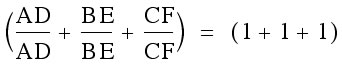and from our previous proof;

So:

Here we have just proven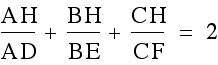

One thing that is very interesting to remember is that the proofs above, will work for triangles that are not neccessarily equilateral triangles. There is a very simple proof of this relationship when dealing with equaliateral triangles. We must first remember that in an equalitaeral triangle, the centers (orthocenter, incenter, centroid and circumcenter) are coincident. Using this knowledge, we can conclude that the orthocenter and the centroid are the same point in an equilateral triangle. Therefore, the orthocenter will be exactly two tirds the distance from the angle to the opposite leg of the triangle. Furthermore, the lenghts of the altitude can be represented by a 2:1 ratio. Lets take a look at a picture

So 2 (HD) + (AH) = AD

and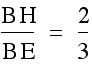  

Using the same property, 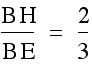and

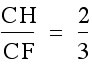
Therefore: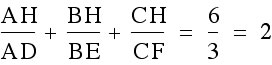

In addition: 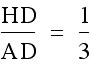and and

Therefore: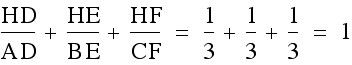

These proofs were very easy to see, but are limited to only equilateral triangles. The proofs found on the top of the page hold true for non-equilateral triangles.

.

Return