Investigation 1:
Consider the equation
.
Let's consider graphing this equation in the xb plane for c values +1, -1, +3, -3, +7, -7. The following graphs are produced:
Conclusion: If the value of c is positive, then the graph produces hyperbolas. If the value of c is negative, the graph produces a hyperbola with a horizontal transverse axis.
If we take any particular value of b, say b = 5, and overlay this equation on the graph, we add a line parallel to the x-axis. If it intersects the curve in the xb plane the intersection points correspond to the roots of the original equation for that value of b. We have the following graph.
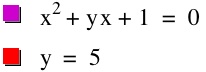
For each value of b we select, we get a horizontal line. It is clear on a single graph that we get two negative real roots of the original equation when b > 2, one negative real root when b = 2, no real roots for -2 < b < 2, One positive real root when b = -2, and two positive real roots when b < -2.
Now, consider the case when c = -1 rather than c = +1.
The following graph is the function we are investigating with different values of c all on the same graph.
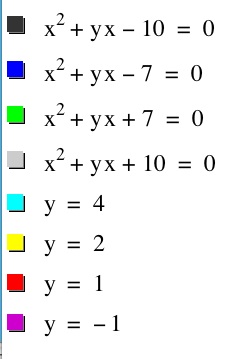
So, we have shown graphs of translations of our function
based on various values of b and c. Now, how do we determine the number and type of zeros or roots of the equation
Below are examples correlated to graphs above.
Investigation 2.
Add the graph of 2x + b = 0 to the picture and discuss its relation to the quadratic formula. The intersection of any horizontal line with 2x + b will occur at a point midway between the two intersections with each of the hyperbolas.
Letting b = y, we see that 2x + b = 0 provides this graph.
The intersection of any horizontal line with 2x + b = 0 will occur at a point midway between the two intersections with each of the hyperbolas.
