Assignment 6
Optimum Kicking Angle
Drew Wilson

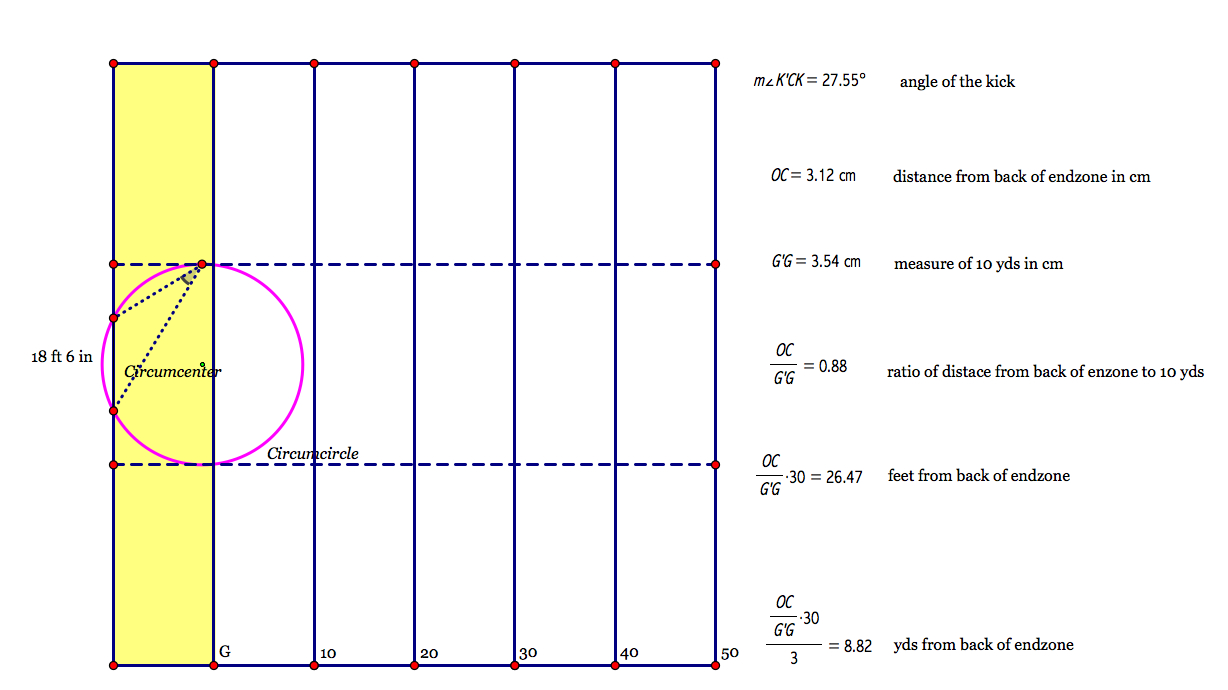
I have heard many times and have also experienced this phenomena myself as a kicker in middle school. I personally felt it was easier to kick from the hash marks the further I was from the goal post, merely because in our eyes the goal post looks wider if we are looking from straight in front of the goal post. Based on my findings, I have found that I was wrong to think there is a better angle from the hash marks if you are further away from the goal post. The first thing we have to consider is at point is the angle the greatest and how do we determine this angle. If we think about a circle that contains the two points of the upright and the point of where the ball is kicked, then we can think of the width of the upright as a chord of the circle. If we want to minimize the angle formed between the place of the kick and the uprights, then we have to minimize the size of the circle because the uprights is a fixed chord, so if the circle is larger then the angle is smaller because the chord is smaller. Therefore, in order to optimize the angle to the goal post from the hash marks, the ball must be kicked from the point where the hash mark is tangent to the circle formed by the three points (two uprights and point of the kick). This would make the circle the smallest because the point on the hash where the ball is kicked and the corresponding point of the other hash mark would form a perpendicular line to the hash mark, thus making the diameter of the circle the smallest and therefore minimizing the size of the circle. Now, if we construct the circumcircle of the triangle formed by the three points, then we can drag the point of the kick until the angle is optimized, which is where the hash marks are tangent to the circle. In the figure below I have provided the angle measurement, as well as the distance from the end of the endzone that optimizes the kickers angle. There is no merit to the commentators remarks about the angle of the kick would be greater if they decided to take a penalty. Based on the figure below, we can see that the optimum angle is acquired at a distance of 8.82 yards from the back of the endzone. The endzone is 10 yards long, so the optimum angle of the kick is acquired inside the endzone, which is not in the field of play. Therefore, there is no merit to the commentators argument that taking a penalty would increase the angle of the kick.
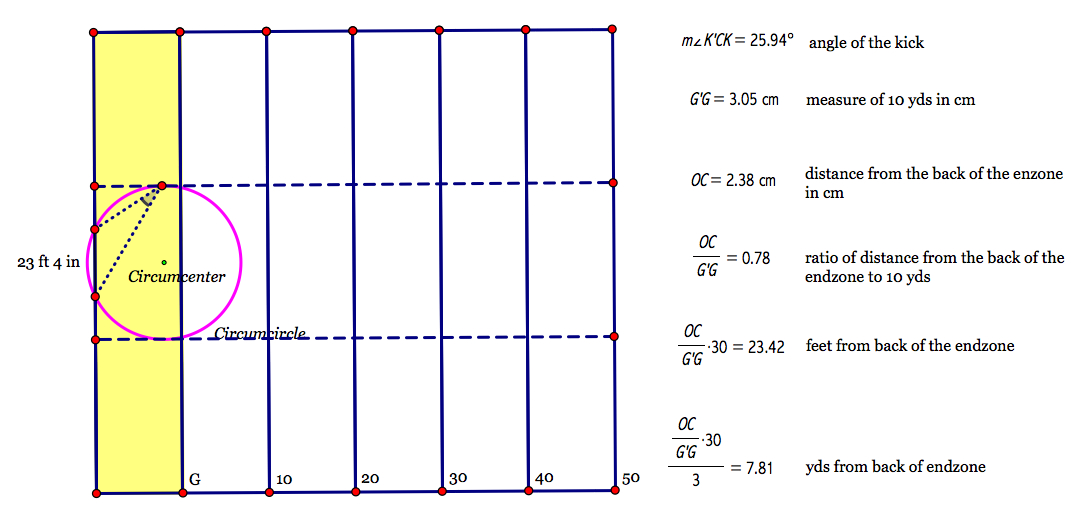
Now, let's see if the optimum angle and distance from the back of the endzone is the same at the high school level. Below is the original figure of the high school problem.

When beginning to work with this problem, my hypothesis was that the optimum angle would not be attained in the field of play because the optimum angle from the hash for a college kicker would be 8.82 yards from the back of the endzone which is not in the field of play because the end zone is 10 yards long. I used the same process to find the optimum angle for a kick from the hash marks as I did for the college problem. I found that a similar phenomena occurs with high school as does with college. The optimum angle for high school, which was 25.94 degrees, is greater than the optimum angle for college. However, the distance from the back of the enzone to the place of the kick was closer for high school than for college. In order to attain the optimum angle in high school you must be 7.79 yards from the back of the endzone, which is 2 yards closer than that of college. Similar to the argument in the college problem, there is not place on the hash mark that would give the kicker a better angle if they decided to take a 5 yard penalty because the optimum angle is attained in the endzone instead of in the field of play. Therefore, the commentators argument would be wrong for high school kickers as well. Below is a picture that includes the optimum angle, as well as the optimum distance from the back of the endzone.