Comparing Standards Forms of a Parabola
by: Maggie Hendricks
There are two standard forms of the graph of a parabola. One is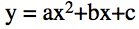 
and the other is +k.jpg)
. If both of these forms represent a parabola, then it can be concluded that the two forms are in some way related to one another. To explore this relationship, let’s look first at the second form ().
If we take and expand the two factors of (x-h), we get: .jpg)
Now we can distribute the factor of a to result in: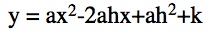 
From here, some grouping of terms will help us establish a relationship between the parameters of the two standards forms.
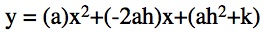
This looks very much like the first standard form of a parabola, where a = a, b = -2ah, and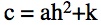 
.
Similarly, we could start with the first equation for the standard form of a parabola and manipulate it to look somewhat like the second.
If we start with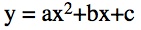 
and divide each term by the factor a, we get:
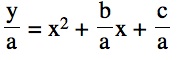
From here, we can use inverse operations to move the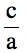 
term from the right side of the equation to the left. This gives us:
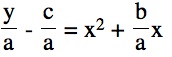
Next, we can complete the square by taking ½ of the 
term and adding the square of this amount to each side of the equation. This gives us:
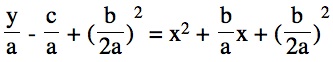
If we factor the right side of the equation, we get:
Now, if we use inverse operations to move all terms except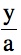 
to the right side of the equation, we get:
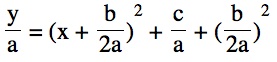
Lastly, if we multiply both sides of the equation by the factor a, we get:
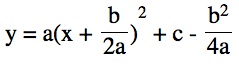
This looks much like our second equation for the standard form of a parabola, if we let a = a,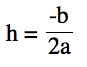 
, and 
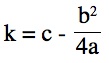. Although, the number and appearance of algebraic terms gets rather complex at some points in this process, both sets of manipulations (the second form to the first and the first form to the second) can help us to see more specifically how the parameters a, b, c, h, and k relate to one another.
Now let’s look at the effect that these parameters have on the shape of a given parabola. Below, we see a variety of different parabolas, with b = c = 0, and a taking on the values of 1, 2, ½, and 3.
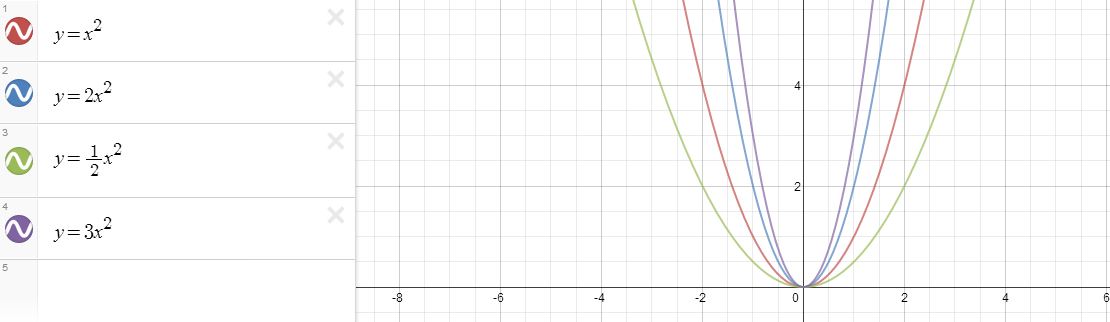
Based on the above graphs, we might conjecture that the parameter a affects the “openness” or wideness of the parabola’s shape. However, it would be more accurate to see that the overall shape of the parabola remains the same in all of the above functions; thus, the parameter a simply dilates what we think of as a standard parabola by the scale factor of a. Another way to think about this is by considering what would happen if we zoomed in or out on the viewing screen of the graph of a basic parabola. The effect would be similar to what we have seen above. Now let’s look at different values of b, with a and c held constant (a = 1 and c = 0).
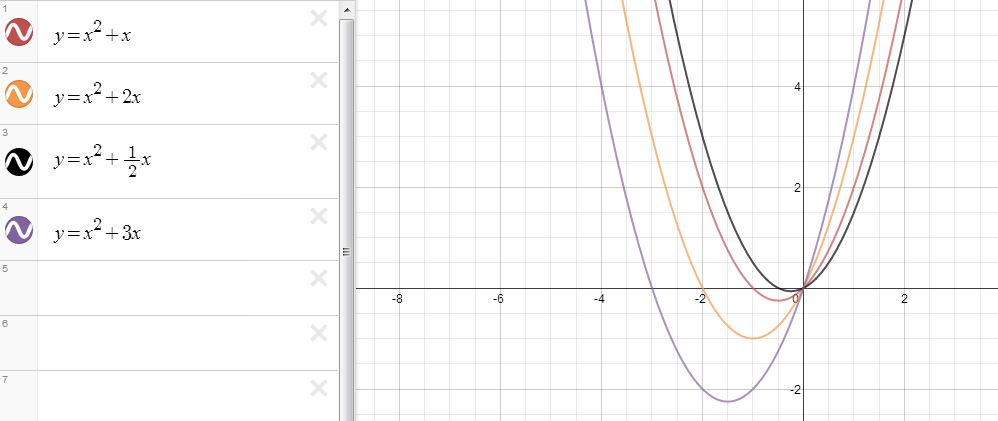
It appears that the parameter b does not affect the overall shape of the parabola, but the placement of the vertex changes for varying values of b. Unlike the parameter a, changes in the parameter b do not change the shape of the parabola at all; rather, the location of the curve is shifted (or translated) along what appears to be a linear path. We might expect this linear path because the parameter b is multiplied by the linear term in the standard form of a parabola's equation (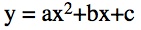). Next, let’s look at varying values of c with a and b held constant at 1.
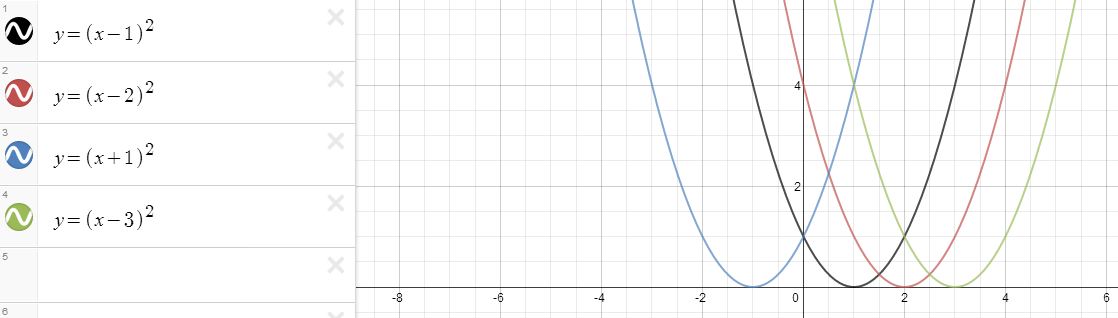
This parameter, like b, seems to affect the placement of the vertex of the parabola without changing the overall shape of the function. What’s unique about the parameter c, however, is that it appears to translate the the vertex vertically for varying values of c. Again, we might expect this simple type of translation from changes in the parameter c because it is the constant term in the standard form of a parabola's equation (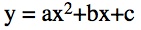). 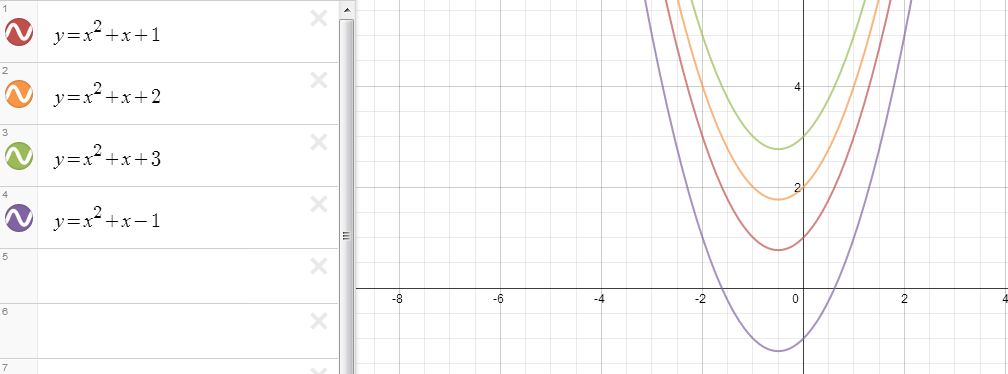Now let’s look at the effect the parameter h has on the shape/placement of a given parabola (we’ll hold a = 1 and k = 0).
Similarly to b and c, we see that h affects the placement rather than shape of a parabola. Changes in the value of h seem to translate the vertex of the parabola horizontally (rather than vertically, as with c). Lastly, let’s look at the effect the parameter k has on the shape/placement of a parabola (we’ll hold a = 1 and h = 0).
Very similarly to the parameter c, k appears to translate the vertex of a parabola vertically. What we have seen in the above graphs ties into the algebraic relationships we looked at earlier. We showed that b and c are closely related to h and k, so it makes sense for us to see these parameters affecting the shape/placement of a parabola in some similar, yet not identical ways.