
Parabola Probs….
By Natalie Streiner
Graph y=2x2+3x-4
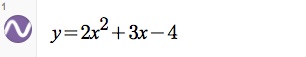
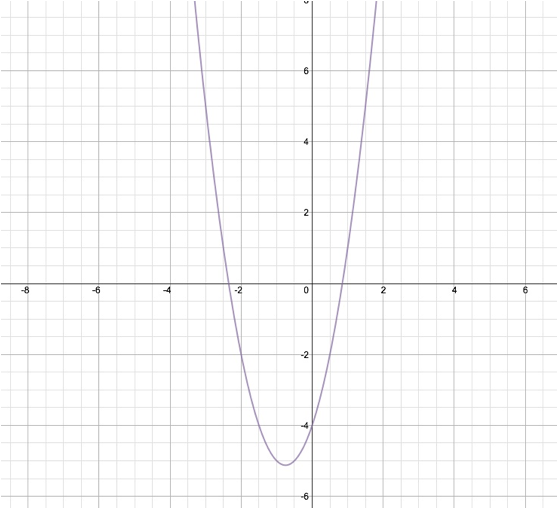
Overlay a new graph replacing each x by (x-4)…
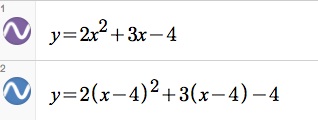
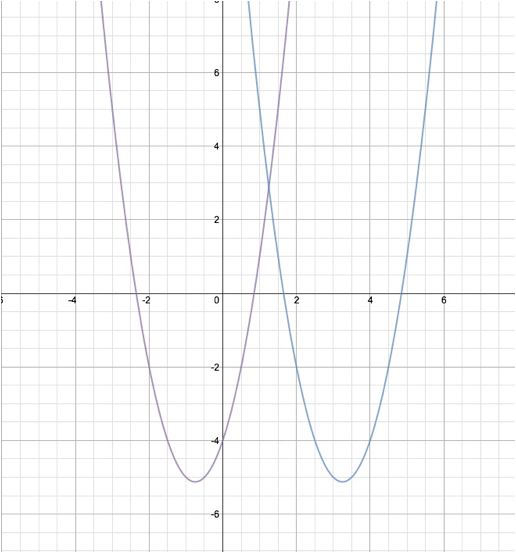
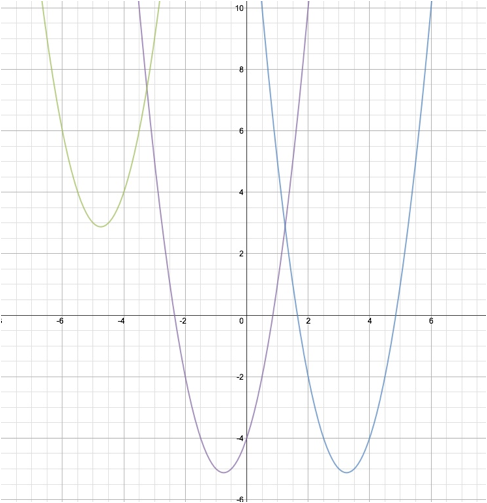
By replacing x with (x-4) we see the entire parabola
shifted to the right 4.
All other properties of the parabola stayed the same.
Change the equation to move the vertex of the graph
into the second quadrant…
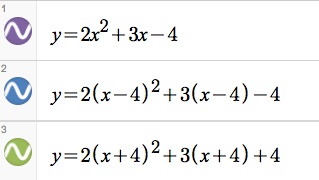
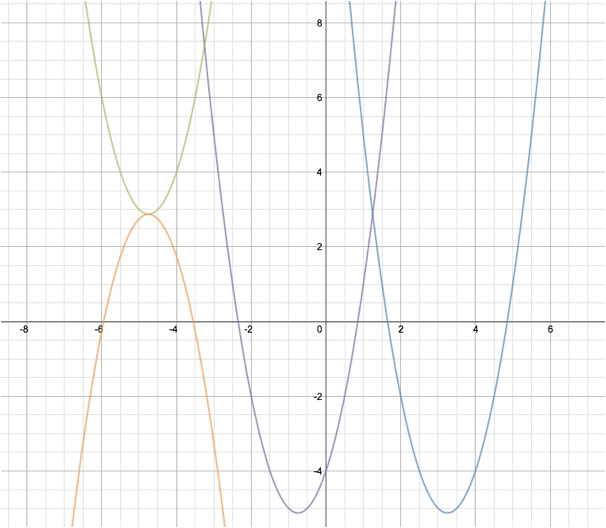
Prior to changing the equation, the vertex of the
graph was in the fourth quadrant. This means both the x and y values of the
vertex were negative. In order to move the vertex to the desired quadrant I
changed all the subtraction signs in the equation to addition signs. This
shifted my graph to the left 4 and up 4.
Change the equation to produce a graph concave down
that shares the same vertex…

This change was a little harder to figure out. It was
simple enough to add a negative sign in front of the equation in order to make
it concave. Finding a value to add in order to have the same vertex as the
graph before was a little harder to figure out. I finally figured out that this
is calculated by using the equation
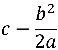
We see that this looks similar to something related to
the quadratic equation or completing the square. By using the
a, b, and c of the equation I was able to find that when (23/4) was
added to the equation it shared the vertex of the prior graph.
Generalize….
We see in order to shift a graph vertically we add or
subtract some constant at the end of the equation.
In order to shift horizontally we add or subtract some
value to x only. Be aware that when a value is added the graph shifts to the
left, and when a value is subtracted the graph shifts to the right. For
example, to shift a graph to the left 2 we would change x to (x+2).
To negate the function, or flip it upside down, we
simply multiply the entire function by negative one.
We also learned that we could use the formula above in
order to match up the vertex with that of another function.