

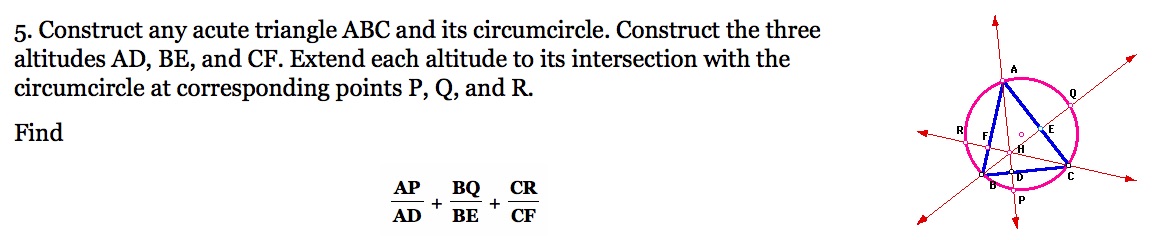
***Before reading this write up, it will be helpful to open this GSP file!!***
If you open the linked GSP file, you will see that I have already constructed the 6 segments seen above. The segments in the numerators are the longer ones seen in solid green while the segments in the denominators are overlayed on top of those longer segments in dotted black. They've been measured and the ratios off the segments added together as seen above is calculated. We've found that these ratios add up to 4. Try dragging any point in the file and you will see that no matter what your acute triangle is, this ratio will always be equal to 4. Now, let's discuss why this is so, because using the measurement tools on GSP isn't enough.
Well we can rewrite each of the numerators as the two segments they are broken up into since the numerators are the longer segments. You will notice that one of these smaller segments is the corresponding denominator. So rewritten they are as follows:
AP = AD + DP CR = CF + FR BQ = BE + EQ
So now we substitute these into our three ratio sum given at the beginning, break apart or separate the numerators, and then simplify to get:
Since we already have a value of 3 as one term of our expression, which we know is always equal to 4, all we have left to do is prove that
is equal to 1.
Well we know that DP = DH, FR = FH, and EQ = EH since H is our orthocenter. These are also our altitudes, or heights, of our 3 smaller triangles. Also note that the areas of the 3 smaller triangles add up to the area of the big triangle. So, we want the proportions of the altitudes of the three small triangles over the altitudes of triangle ABC to sum to 1. So we will set up three different area formulas of triangle ABC with a corresponding small triangle area formula. Using the area formula A = 1/2bh we define the following formulas:
Added together, these three formulas give us the area of triangle ABC. Now, the area of triangle ABC can be given as three different formulas, which be numbered corresponding to the three above as follows:
We can say that the sum of the first set of three area formulas will equal any three of area formulas in our second set, so those can be used interchangably for the area of triangle ABC. So adding them together we get the following equation:
And since we want the sum of these three smaller areas to sum to 1, then 1 should be on the RHS of this equation, so let's divide by the area of triangle ABC, which remember, can be any of the three formulas from our second set of area formulas. Well in doing this, we get a lot of cancellations with 1/2, (BC), (AB), and (AC). If you go back and look towards the beginning of this write up, we stated that DP = DH, FR = FH, and EQ = EH, so these can be substituted back in and we end up with
Well remember, that through this last part of the proof process, we had set our RHS equal to one, then used substitution to get back to the three ratios above for our LHS of the equation. Therefore, we have shown that this expression of three ratios is equal to 1. So finally going back to
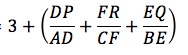
we substitute to get 3 + 1 =4. And we have finally shown why the sum of the original three ratios is equal to 4!
Return to Home Page