
by Courrey J. Alexander
The CENTROID of a triangle is the common intersection
of the three medians. A median of a triangle is the segment from a vertex to
the midpoint of the opposite side.
Given
a triangle, we construct the medians of the triangle.
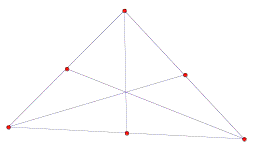
Here
we have the three medians of the triangle. The three medians of the triangle are concurrent. The point of concurrency is, of course,
the centroid of the triangle.
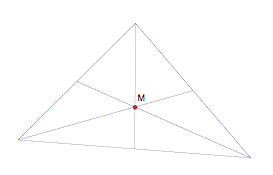
Therefore,
by definition, point M must be the centroid of the triangle.
I
conducted an investigation, assuming that M is the centroid of the triangle. The investigation involved finding the
ratio of the distance from each vertex to the centroid to the measure of its
corresponding median.

I
found that all three ratios are the same.
I went farther and did a GSP animation
on the points to see if this would change.
Although
the distance from each vertex to the centroid and the measure of its
corresponding median changed, the ratios remained the same. Therefore, it is my conjecture that the
medians of a triangle intersect at a point that is two thirds of the distance
from each vertex to the midpoint of the opposite side.
According
to this special concurrency property for medians of a triangle, if M is the
centroid of DABC,
then AM=2/3 AD, BM=2/3 BF, and CM=2/3
CE.