by Courrey J. Alexander

Construct a triangle and explore the altitudes and orthocenters of the triangle.

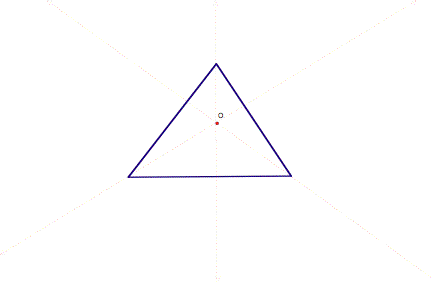
Here, O is the orthocenter of triangle ABC. The orthocenter is inside the triangle. But, is the orthocenter of a triangle always on the inside? Manipulate any of the points (other than point O) in this GSP construction to find the answer to this question.

Let’s look at the circumcircle for triangle ABC.

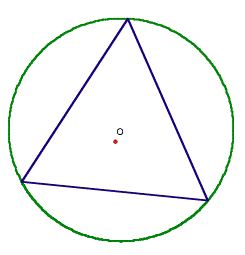
What happens when we construct the orthocenters and circumcircles of the other triangles in the original construction? Let’s find out!

First, let’s find the orthocenter for triangle OAB.

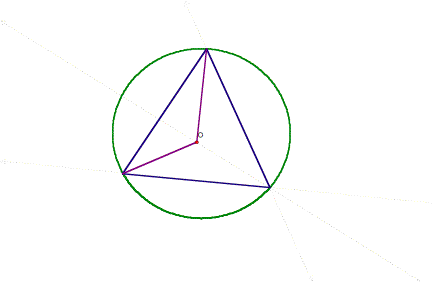
Here we see that point C is the orthocenter of triangle OAB. Let’s look at its circumcircle.

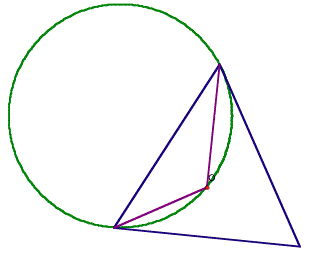
Next, let’s find the orthocenter for triangle OBC.

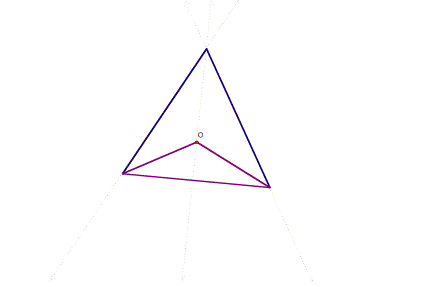
Here we see that point A is the orthocenter of triangle OBC. Let’s look at its circumcircle.

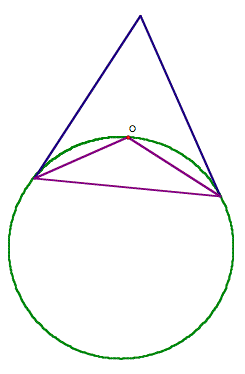
Finally, let’s find the orthocenter for triangle OAC.

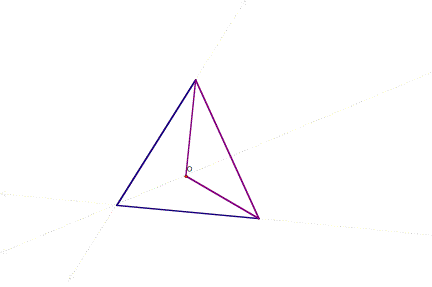
Here we see that point C is the orthocenter of triangle OAC. Let’s look at its circumcircle.

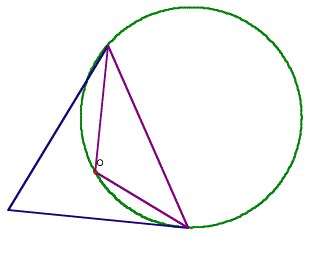
Let’s look at the entire construction.

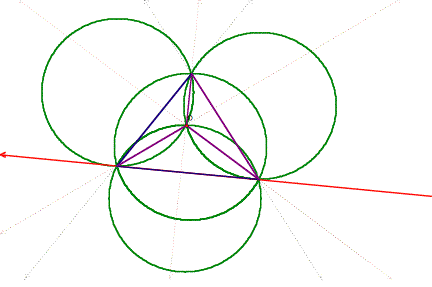
We have observed that the orthocenters of the triangles formed by the orthocenter and the vertices of the original triangle are actually the vertices of the original triangle.

The radii for all 4 circles seem to be the same.

What happens to the construction when points A, B, and C are collinear? Open the GSP file to investigate.

Return to Homepage