The Circumcenter

Damarrio Holloway

July 5, 2006

The Circumcenter (C) of a triangle is the point in the plane equidistant from the three vertices of the triangle. (C) is a point in the plane where all of the perpendicular bisectors of the segments are concurrent. We will explore the various locations of the circumcenter with different types of triangles, assuming that C is not within the interior of the triangle.

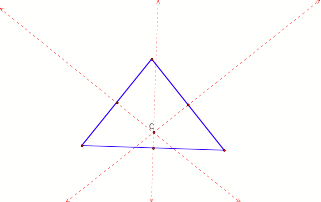
In this example, the Circumcenter (C) is located within the interior of the triangle. The perpendicular bisectors are drawn in red, where their intersection point is the circumcenter.

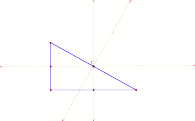
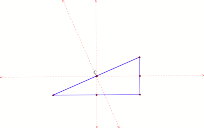
We see on a right triangle, that the concurrent point is located on the triangle. The circumcenter will always lie on the side opposite the right angle of a triangle, specifically on the midpoint of hypotenuse.

Suppose we try to find the circumcenter of an obtuse triangle.

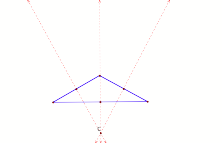
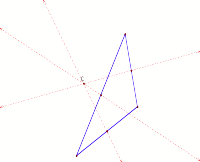
With the perpendicular bisectors drawn in red, their intersection in graph 3, is outside the triangle. Therefore, with obtuse triangles, the concurrent point of perpendicular lines drawn to an obtuse triangle is an exterior point within the plane.

After discovering that the circumcenter is not necessarily located inside the triangle, lets discover the location of C when the triangle is inscribed within a circle.

Going back to graph 3, we see that the circumcenter of the triangle still holds true as being constructed outside of the triangle. (C) is also the center of the circle. By using the circumcenter and a vertex of the triangle, we were able to circumscribe a circle about the triangle. With the help of the red dashes indicating the radii, we have constructed a circumcirle where (C) performs two functions.

Return