During this assignment I explored different types of tangent circles and discovered that if you trace the center of a circle that is tangent to 2 other circles, it produces an ellipse around the centers of the 2 given circles. For example here are two given circles (blue) and a tangent circle (red) where when the given point on the bigger blue circle is rotated, the trace of the center of the tangent circle creates an ellipse.

This is also true when the given point is on the smaller circle and is rotated around.

It is also interesting to note that if the tangent circle is outside the smaller circle but inside the bigger circle, then the ellipse is still formed by tracing the center point, whether it be constructed from a given point on the bigger or smaller circle.

The construction of the tangent circle is quite different if the 2 given circles intersect. Below are constructions for cases when the 2 given circles intersect at 1 point and when they intersect at 2 points.

As you can see the loci for the tangent circle center (ellipse) always passes through the points of intersection, whether it is 1 point or 2. In either case, the construction of the tangent circle is still the same. The interesting but rational concept is that the tangent circle passes through the intersections and still remains between the 2 given circles. Hence the ellipse stays inside the 2 given circles. Therefore my conjecture is that when the 2 given circles intersect, the tangent circle passes through the intersections and henceforth the loci of the center point of that circle remains an ellipse and also is constructed where if you were to construct a line from the center points of the 2 given circles, the intersection of the line and ellipse are equal distant from each center. By the sketch below, points A and B are equal distant from both centers of the 2 given circles.

Now lets explore the construction of a tangent circle of 2 disjoint circles. As you can tell from the sketch below that the construction is the same as previous problems where you construct the perpendicular bisector of the triangle to get the tangent circle.

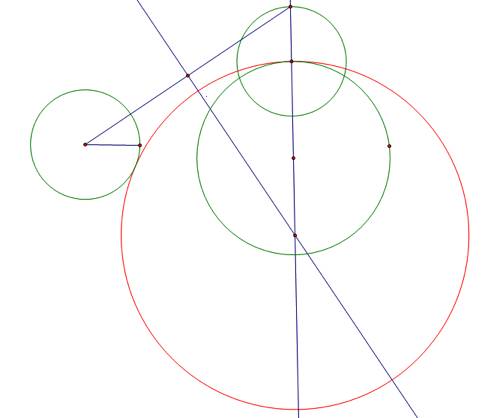
Inscribed given circles with point on bigger circle

 

 

Tangent circle with point on smaller given circle

 

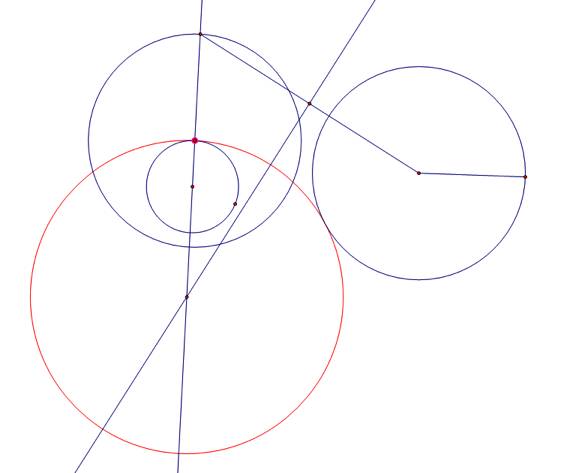
Inscribed given circles with point on smaller circle