Christa Marie Nathe
Faith In Construction
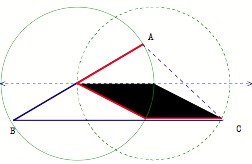
The objective in this investigation is to locate a
point x on line AB such that when
connected with point y on line BC
there will be three equal line segments.
The following construction shows the relationship described above, where
we desire for the line segments of Ax, xy, and yC to be equal.
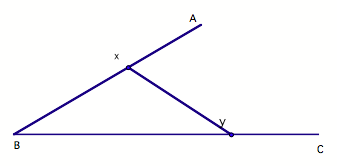
The subsequent steps and related figures will show how
to find points x and y such that there position will yield three equal line
segments.
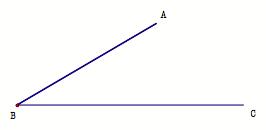
Start by creating an angle and labeling it ABC
Now create a line from A to C, to make the angle into
a triangle and plot a random point P on line BC.
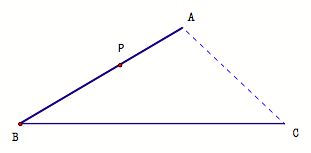
The line AC was created to give us a familiar shape to
work with, of which it might help to influence our ability to find the desired
points x and y. Point P is not a fixed position, it is there in
order to give us something to manipulate in our figure.
Constructing a circle with the point P as the center
with radius PA we can develop more geometric relationships within the
figure. In addition, we can use
draw a line parallel to BC through point P which gives us yet another geometric
association.

The previous figure tell us that PQ=PA.
By creating another circle with its center at Q, with
the same radius of PQ, we can take full advantage of the length of the radius
to help determine where point x
ought to be placed. Currently, we cannot manipulate the radius or the circle to
do anything useful.

This ensemble is rich with associations as we can see.
The two circles with equal radii allow us to form a rhombus. By connecting PQR
to form a triangle and reflecting it over the line PR we obtain a rhombus.
Lucky for us, a rhombus has two characteristics that work in our favor; its
sides are equal in length (in our case, the radius of the circles) and its
opposite sides are parallel. As you remember, the light blue dashed line was
created parallel to BC. If segment PQ is parallel to BC, then that means that
SR is parallel to both BC and PQ that aids in our quest to find the three equal
line segments. We have thus far established some helpful equivalent relations.
By dragging point P, remember it is not fixed, down
the line AB segment, we are increasing the size of the circle therefore its
radius, which directly affects the sides of the rhombus. We can position P such that the radius
PA=PS=SC of the rhombus.