

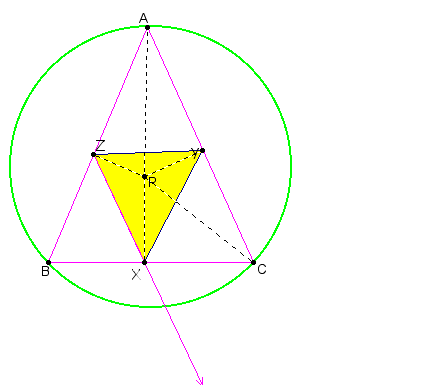
So first, let's look at a the Pedal triangle. We know that if point P is any point in the plane, then the triangle formed by constructing perpendiculars to the sides of ABC form the points Z, Y, Z, which are the intersections. below is a basic construction of the pedal triangle.

Now, as the point P approaches any of the vertices on triangle ABC, the pedal triangles points Z, Y, and X form a straight line which means that they are collinear.
SEE GRAPH HERE !(play with the pedal point P and see if a line is formed when it is on top of the vertices of the triangle ABC)
Now what happens if the pedal point is outside the graph? Below, I have constructed a circumcircle with the pedal triangle.
Now if we let p be a point on the circumcircle, we have the following:
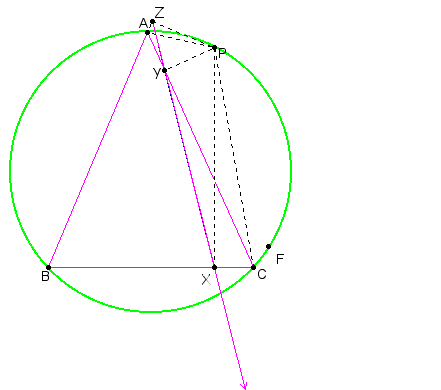
So wlog, let PABC be a cyclic quadrilateral. From the right angles, PYXC, PZAY and PXBZ are cyclic like PABC.
With angle APC = 180 - angle ABC = angle XPZ and by subtracting angle APX, we have angle XPC = angle ZPA = angle ZYC = angle ZYA.
Therefore, angle XYC = angle ZYA, so X, Y,and Z are collinear and the pedal triangle is degenerate. Thus, all conditions have been shown where x, y, and z are collinear.