
EMAT 6600
Problem Solving
Given an isosceles
triangle ABC with AB = AC and the measure of angle BAC = 100 degrees. Extend AB
to point D such that AD = BC. Now draw segment CD. What is the size of angle
BCD?
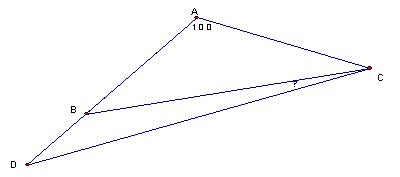
Solution: By properties of an isosceles triangle, we know that angles ABC and ACB are congruent (40 degrees each). From there, we know DBC is 140 degrees. Well, that does not help. Let us build some auxiliary construction, as suggested by Dr. Wilson. First, rotate A 60 degrees clockwise of D and let that point be E. Thus, triangle DAE is an equilateral triangle.
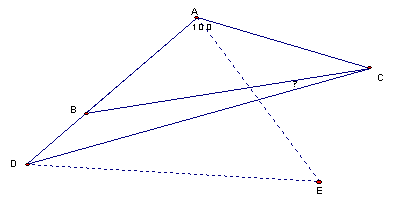
Then construct segment EC and label every angle than you know up to this point.
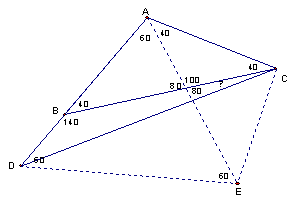
Take notice that DC bisects angle ADE. If not construct the angle bisector for ADE and observe the following.
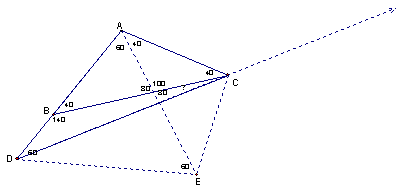
So, we can state that DC ┴ AE. Thus, a 90 degrees angle. From this point, one can do the operation to find the measure of angle BCD. The angle measures 10 degrees.