

EMAT 6600
Problem Solving
An Exploration of
Polya’s Problem
For a certain given triangle ABC, from any vertex construct an angle bisector, the median, and the altitude. Given that the vertex angle is dived into four equal angles, find the size of the angle at the vertex, as depicted below.
fig 1

Let us name the new points.
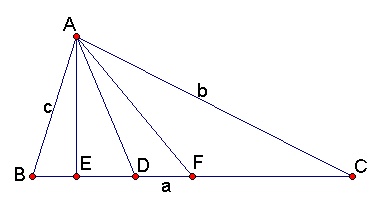
Since all the angles from the vertex are congruent, AE is the bisector of ÐBAD, AD is the bisector of ÐEAF, and AF is of ÐDAC. So, DABE ≈ DEAD by ASA. Thus, we can state that DBAD is an isosceles triangle.
With this note in mind, for both triangles, angles ÐEBA and ÐBAE sum up to 90 and angles ÐADE and ÐEAD sum up to 90. However, angles ÐBAD, ÐADB and ÐDBA sum up to 180. Also, 4(ÐBAE) < 180, which implies that ÐBAE < 60.
Now, since we know the sum of the angles of a triangle equal 180, we can use that to set up some equations/inequalities. This is very elementary.
Suppose the following: x + y = 90
x + 4y £ 180
where x is the measure of the angles ÐABE and ÐADE and y is the measure of the angles ÐBAE and EAD. From there, we obtain that y £ 30 and x ³ 60.
With a bit of reasoning, we see that 30 and 60 would not work, for 60 + 4(30) =180 and not less.
So the angle ÐA can measure from 4 to 116
or ÐA = {x| 4 < x < 116}.