
An Exploration of Parametric Curves
by
Larousse Charlot
We have seen parametric curves many times in the form of functions. For instance, y = f(x). We did not point them out because maybe that was not relevant at the time. Parametric curves often happens when an object moves, and when tracking the motion of the object, it is useful, or easy to think of the objectÕs position as a function of time. In a two dimension plane, the functions of a parametric curve are given by x(t) and y(t). For example,
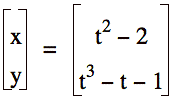
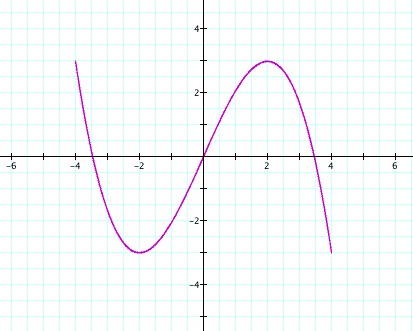
Although a polynomial seems simple, it might produce complicated parametric graph, such as the following:
-2 £ t £ 2
fig. 1
Investigation of Lissajous Curve
Lissajous curve is also known as Bowditch curve due to the fact that Nathaniel Bowditch first studied the curves in 1815. However, Jules-Antoine Lissajous was the one who studied it in detail 1857.
Consider the parametric equations:
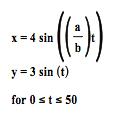
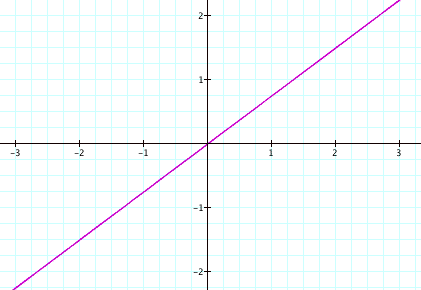
a = b = 1
For a = b, we have a straight line.
The parameter t, here, represents an angle that defines the motion and the position of the graph (from the ray (x,y) and the y-axis).
If we were to change the value of (a/b) to ½, we have the following:
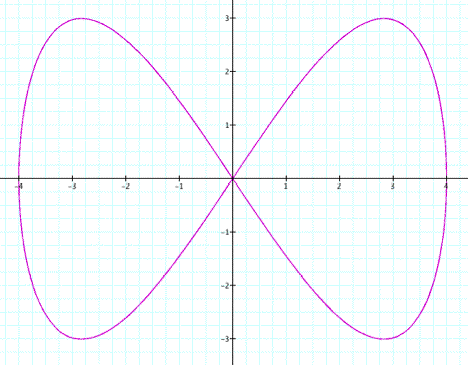
a ¹ b a = 1 b = 2
what we have here is the sine function oscillating with amplitude of 3 along the y-axis and amplitude of 4 on the x-axis. Observe what happens as the value of a/b = ¼.
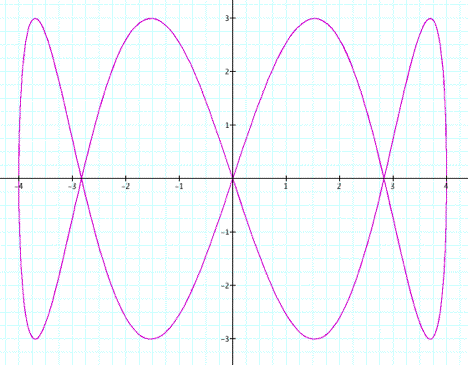
a = 1 b = 4
We see here that the period of the graph remains the same, yet its frequency changed. The period of graph is determined by t, which is the angle upon which the graph is built. Remember that t remains in the following set
![]()
For a/b = 2/3, we have
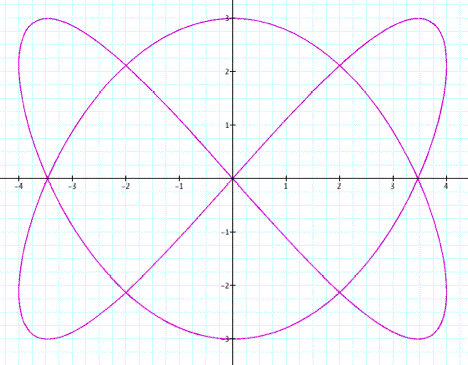
a = 2 b = 3
and a/b = 12/13 we have

a = 12 b = 13 for -70 £ t £ 70
with our observation, we can conclude that the graph of the parametric equation

is highly sensitive to the value of a/b.
To get a general understanding of what has happened to the graphs weÕve just seen, let us compare it this parametric equations:
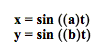
keeping the value of t in the set of 0 £ t £ 50.
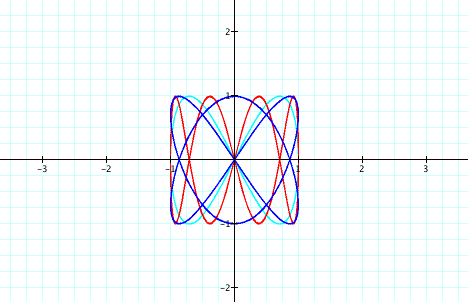
Light blue: a/b = ½ red: a/b = ¼ blue: a/b = 2/3
Notice that the graphs are exactly the same, except the fact of the amplitudes of the sine functions are 1.
What we have observed in the graphs are the combinations of several simple harmonic motions, it repeats itself at a standard interval, and it oscillates with constant amplitude, as previously mentioned. That is a complex harmonic motion, which is described by the class of Lissajous curve.
-70 £ t £ 70
If you were to observe the graph with its ratio as 1/3, you will notice that we obtain a simple harmonic curve as sin x with a frequency of 1/3 as display above.
Click here to try different ratio of a/b.