The data set below is based on the first class letter postage for the US Mail from 1933 to 1996. Plot the data and develop a prediction function. When will the cost of a first class postage stamp reach $1.00? When will the cost be 64 cents? How soon should we expect the next 3 cent increase?
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Now let's graph our points to see what our data looks like.
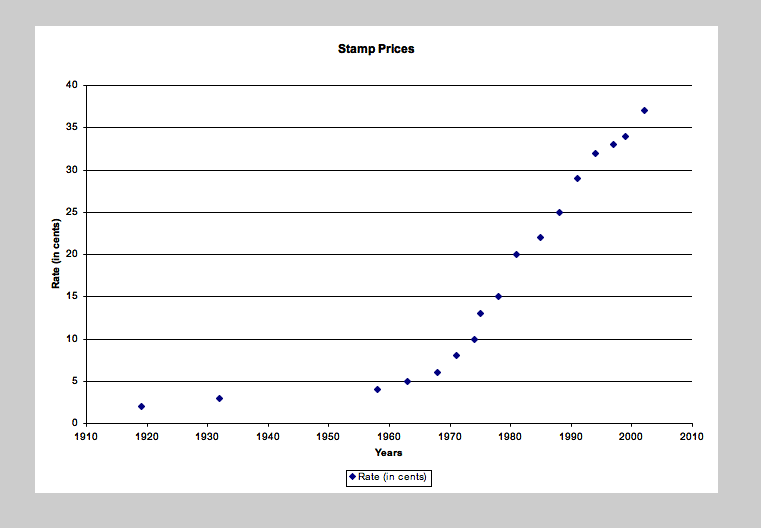
Now let's try plotting a linear regression to see if we can come up with some sort of equation for our line.
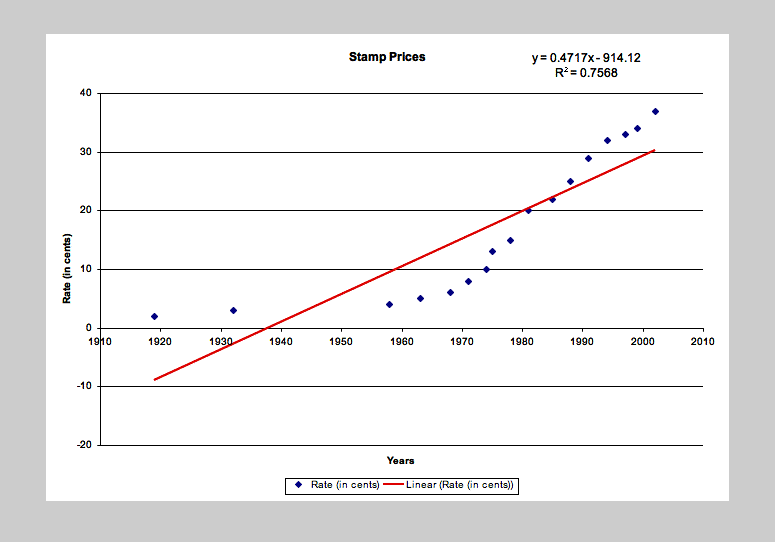
We can see that the line doesn't
work so well with our points. And our correlation for the linear
regression is only .76, which is okay, but it could be better.
What does the number 
mean? 
is
the correlation for our equation and our points. 
refers to the fraction of variance explained by a model. The closer
our correlation gets to 1, the better that equation will fit the
pattern of the data that we have been given. Let's try a different
trendline and see if we get a better correlation.
Here is a trend line using an power equation.
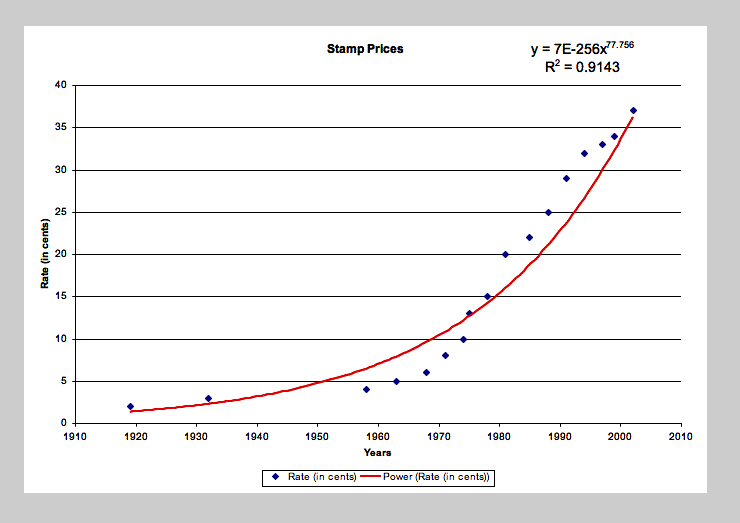
Now our correlation is .91, which is definitley better than .76. This means that we have found a better equation to help predict future stamp prices than the previous linear regression. However, we should check out a few more before we settle on this one.
Let's look at an exponential curve to see if its correlation is any higher.
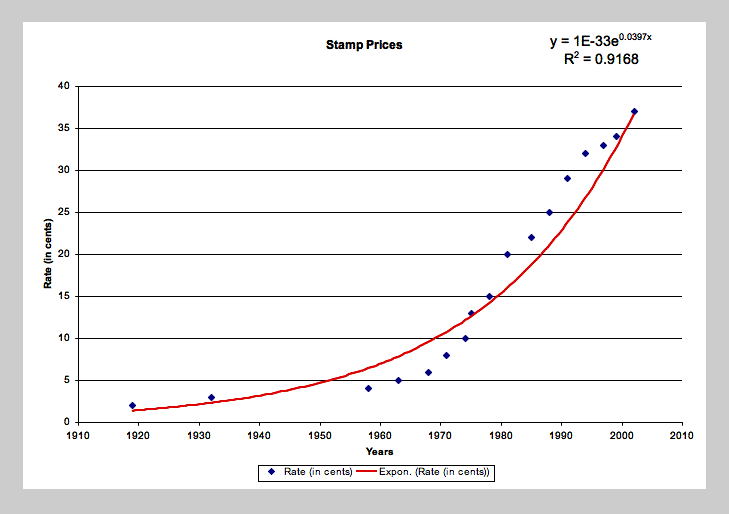
Our correlation number is a little bit higher now, going from .91 to .92 so this equation will predict future stamp prices better than our previous power equation.
Let's try one more using a polynomial.
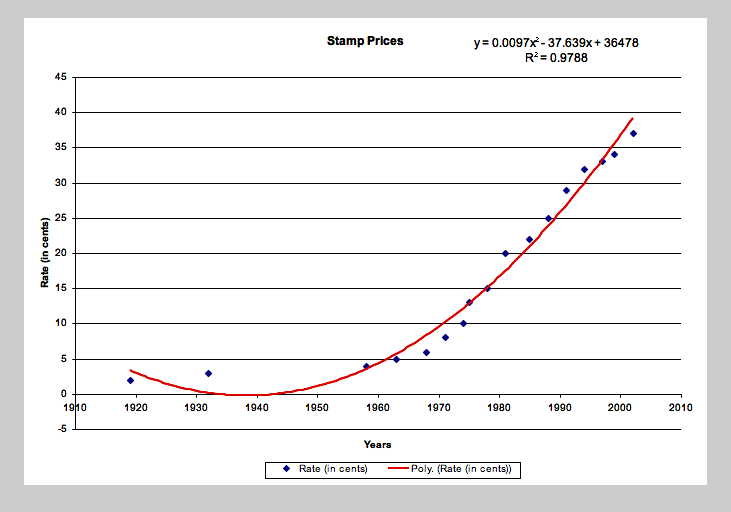
Wow, our correlation has jumped all the way to .98. That is really close to 1, which is our ideal correlation. However, the gap of data between the years 1919 and 1932 and 1958 are bothersome. Let's try replotting the data starting with the year 1958 and see what we get.
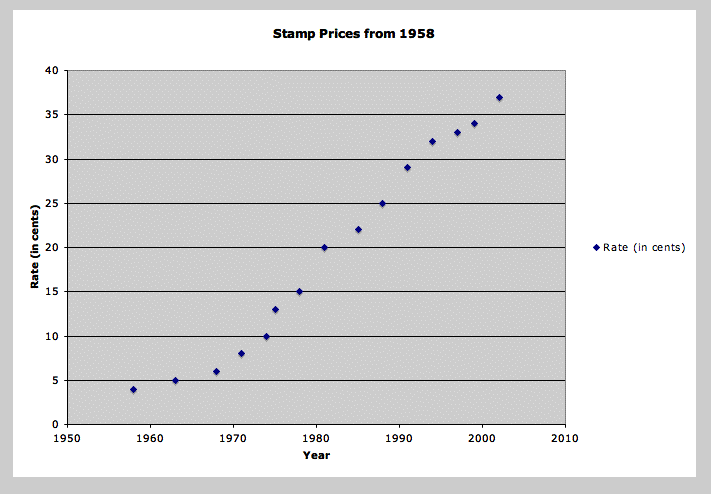
Now let's plot another linear regression using our new chart and see what we get.
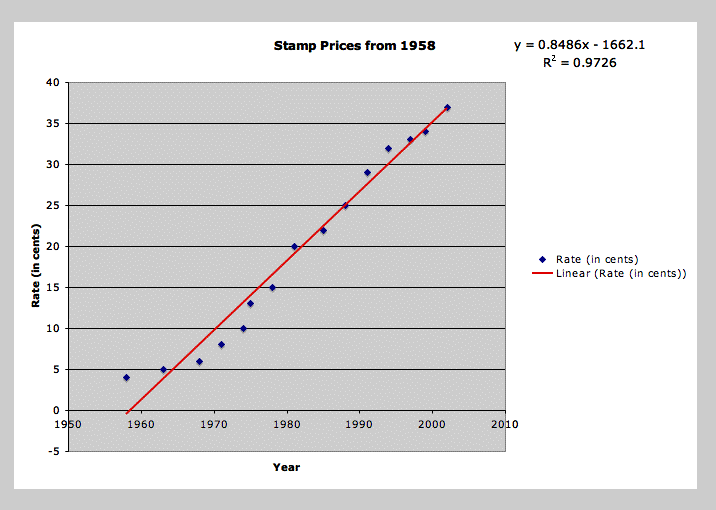
If we use the new data, then our correlation is .97 which is really close to the correlation we got using the polynomial above. Now let's use just the linear regression line to figure out what the future will bring as far as stamp prices go. We could try using the polynomial, but it would be extremely hard to factor it and try to figure it out.
Predictions using linear regression:
When stamps will cost $1.00:
100 = .8486x - 1662.1
x = 2076
When stamps will cost 64 cents?
64 = .8486x - 1662.1
x = 2034
When will the next 3 cent increase happen? A 3 cent increase would bring the cost of stamps to 40 cents, so
40 = .8486x - 1662.1
x = 2005
So it has already happened.
By Carolyn Amos