

Let's explore the graph:
What happens when you plug in different values for d? Does changing d alter the shape of the graph? Does changing d alter the position of the graph?
First lets look at the graph wih no value plugged in, where d = 1.
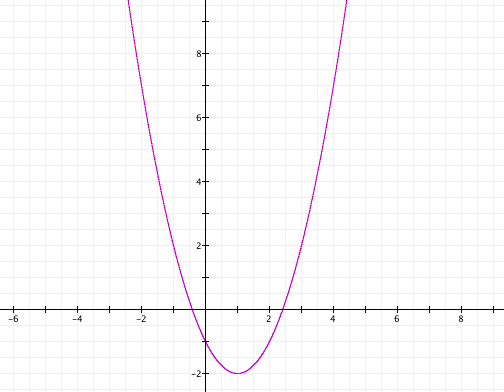
What do we notice about the graph? First, we see that it is a parabola that is opening up. When d = 1, the graph's line of symmetry occurs at x = 1. Also, that the lowest point is at -2, which means that -2 is the functions minimum value.
Now let's plug in some other values for d and see what happens to the graph.
First lets plug in some positive values for d.
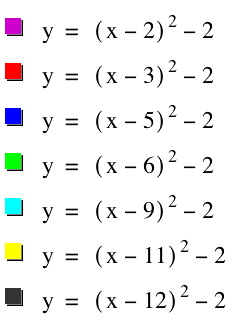
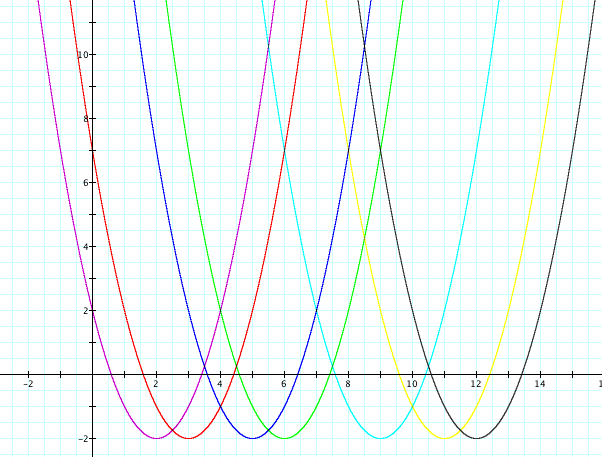
Now lets look at the some negative values for d.
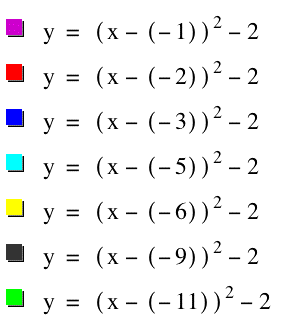
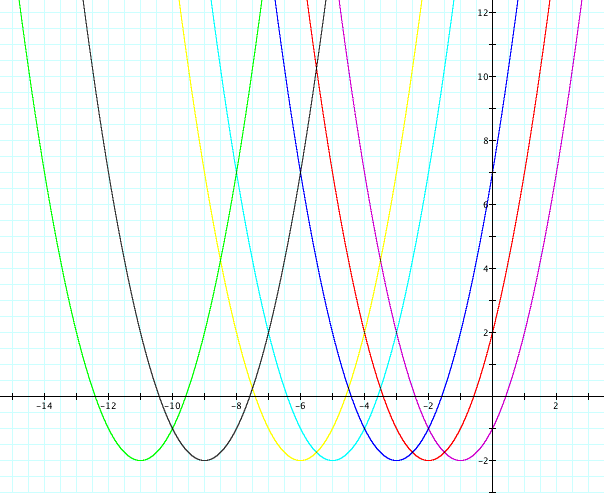
The shape of the graph does not change no matter what the value of d. The parabola always opens upward, no matter the value of d. The lowest y-value of -2 does not change no matter what value of d is plugged in. For whatever value of d that is plugged in, that is where the line of symmetry occurs for that parabola. If d = -5, then the line of symmetry occurs at -5. d is also the x-value which when plugged into the equation gives the minimum value of the function, which in this case is always -2. The postion of the graph depends upon the value of d. The line of symmetry shifts to whatever value d is. So the graph only changes position and not shape.
What will happen if you remove the -2 from the equation? Let's graph it and find out.
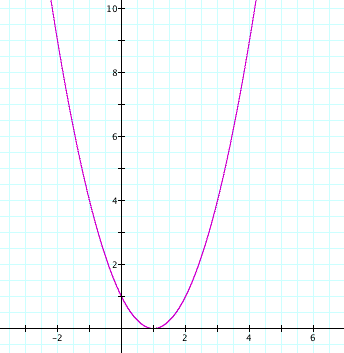
When the -2 is removed, the lowest point on the graph shifts back to the x-axis from where it was at -2. So we can assume that the number in that place tells us where along the y-axis the minimum value of the function lies. Once again, the shape of the function does not change, but the position does.
By Carolyn Amos