

Investigate:
Note:
- When a and b are equal, and k is an integer,
this is one textbook version of the "n-leaf rose."
- Compare with
for various k. What if . . . cos( ) is replaced with sin( )?
![]()
First let's take a look at our graph of:
with a and b equal and k as an integer (in this case we will vary k, and in our graph we call it n).......
We can see from our first bit of exploration that our "rose" when a=b that for k=0, we have a circle with radius a+b, in this case 1+1=2, but when k>0 we have a rose with k number of petals.
Now let's take a look what happens to our graph when a>b. We will start with a=2 and b=1, but after take a look at that graph let a=3 and b=1, then a=4 and b=1.
Click here to explore these values
What we see again, is at k=0 we have circle of radius a+b, but when k>0 is our "rose" now does not meet in the center, in fact we have a circle in the middle of radius a-b. If we apply this to our findings above where a=b=1, it works there as well, because in that case 1-1=0, and it appeared as if we did not have a circle in the middle of our rose (but in fact we did have a circle but of radius 0). Let's confirm our findings...........click here
So our next exploration will be to take a look at:
Let's start with b =1 and again let our k=n (where we vary the value of n).......Click here
What we find is that when k is even we have 2k petals and when k is odd we have k petals. Now let's explore what happens if we change the value of b.....Click here for demonstration
What we find here is similar to our findings above, that when k=0 our graph of :
![]()
is a circle with radius b, and that when k is even we have 2k number of petals, which are of length b, and if k is odd we have k number of petals of length b.
Now let's take a look at our graph if we change our function to sine.....
![]()
What we find is that when k=0 we do not have a graph, this makes sense because Sin(0) = 0, and if K>0 then we have our two cases again, if k is even we have 2k number of petals with length b, and if k is odd we have k number of petals with length b.
Other things you might want to explore:
what happens when our k value in the equation is not an integer......
Do we still get a rose? what does it look like?
Well, let's take a quick look at a couple of examples and then I will let you explore it deeper if you wish to.
Let's let K= 3/2 and then K = 5/2 (notice that when we graph these functions that our Theta value is 0 to 14, (instead of 0-6.28) to get 2 full rotations)...........
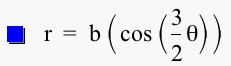
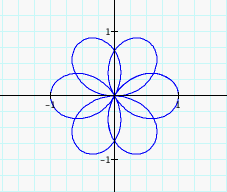
and......

What we find is that in our first diagram for the equation with 3/2 we get 2 six-leaf roses, and for our second diagram for the equation 5/2 we get 2 ten-leaf roses
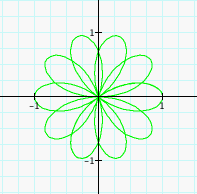
now let's look at equations with other fractions and see if what I think is our trend is holds true....
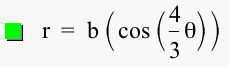
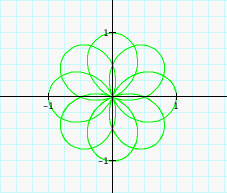

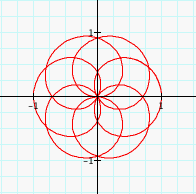
My first thought did hold up in these cases as well, it seems that when we have fractions as our coefficient of Theta, that our resulting rose is as follows:
our number of roses = our denominator
and,
our number of petals = our numerator*2
(keep in mind that your parameters for Theta must go from 0 to 6.28*your denominator to get the full rotation, and make your roses complete)