

In this investigation, we are going to explore pedal triangles and the different characteristics depending on where the pedal point P is located. First, let's define what a pedal triangle is:
Let triangle ABC be any triangle. Then if P is any point in the plane, then the triangle formed by constructing perpendiculars to the sides of ABC (extended if necessary) create three points R, S, and T that are the intersections. Triangle RST is the Pedal Triangle for Pedal Point P.
So, let's look at an example of a pedal triangle for triangle ABC where pedal point P is inside triangle ABC.
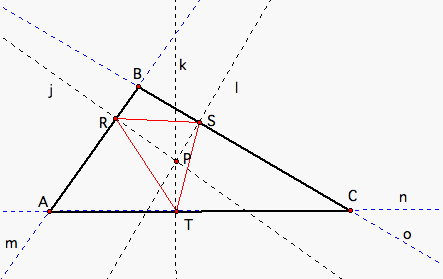
From pedal point P, we constructed three lines (j, k, and l) that are perpendicular to the three sides of the triangle (AB, BC, and AC). The intersection of AB and j created point R. The intersection of BC and l created point T. The intersection of AC and k created point S. Triangle RST is then created and this is the pedal triangle of triangle ABC. Notice that in this case, the pedal point is inside triangle ABC and thus, the pedal triangle RST is also inside triangle ABC. If we move point P so that it is outside triangle ABC, the perpendicular lines j, k, and l will intersect the sides of triangle ABC when the sides are extended by the lines m, n, and o. Here is an example of pedal triangle RST where P is outside of triangle ABC.

Click HERE to move vertices of triangle ABC and the pedal point P and observe what happens to pedal triangle RST.
Now we will look at special cases where the pedal point P is the centroid, circumcenter, incenter, orthocenter, and center of the nine-point circle of triangle ABC.
First, we will define the centroid, circumcenter, incenter, and orthocenter. The centroid is the common intersection of the three medians. The circumcenter is the common intersection of the three perpendicular bisectors. The incenter is the common intersection of the three angle bisectors. The orthocenter is the common intersection of the three altitudes.
When the pedal point P is the centroid of triangle ABC, pedal triangle RST will always be inside triangle ABC since the centroid of triangle ABC is always inside triangle ABC. Let's look at an example of pedal triangle RST where P is the centroid of triangle ABC.
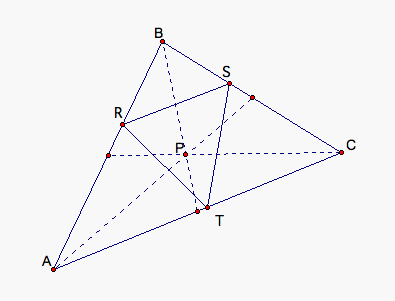
Notice that pedal point P is the common intersection of the three medians. Click HERE to move the vertices of triangle ABC and observe the changes in pedal triangle RST.
When the pedal point P is the circumcenter of triangle ABC, pedal triangle RST will also always be inside triangle ABC even though the circumcenter can be outside of triangle ABC. Pedal triangle RST is also the medial triangle. Let's look at an example of pedal triangle RST where P is the circumcenter of triangle ABC.
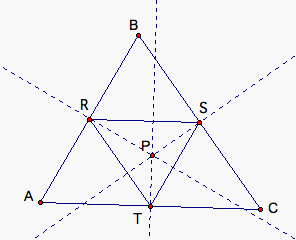
Notice that pedal point P is the common intersection of the three perpendicular bisectors. Click HERE to move the vertices of triangle ABC and observe the changes in pedal triangle RST.
When the pedal point P is the incenter of triangle ABC, pedal triangle RST will also always be inside triangle ABC since the incenter is always inside triangle ABC. Let's look at an example of pedal triangle RST where P is the incenter of triangle ABC.
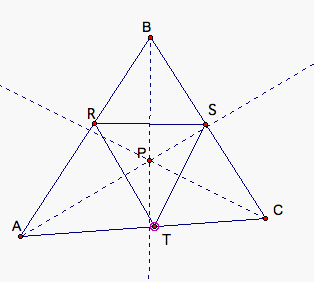
Notice that pedal point P is the common intersection of the three angle bisectors. Click HERE to move the vertices of triangle ABC and observe the changes in pedal triangle RST.
When the pedal point P is the orthocenter of triangle ABC, pedal triangle RST will either be inside triangle ABC or it will be partly outside triangle ABC. Pedal triangle RST will also be the orthic triangle of triangle ABC. Let's look at two examples: the first is where pedal triangle RST is inside triangle ABC and the second is where pedal triangle RST is partly outside triangle ABC.
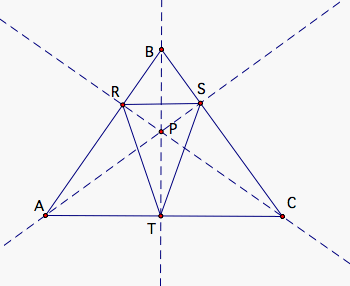
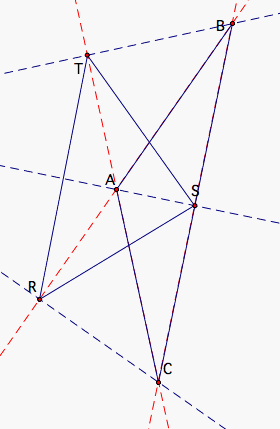
Notice in both examples that pedal point P is the common intersection of the three altitudes of triangle ABC. We can see that in the second example, the sides of the triangles must be extended to see the intersections. Click HERE to move the vertices of triangle ABC and observe the changes in pedal triangle RST.
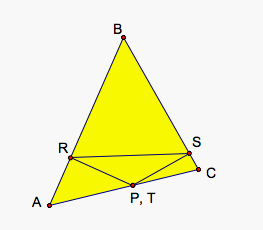
Now let's look at pedal triangle RST when pedal point P is on a side of triangle ABC and when pedal point P is one of the vertices of triangle ABC. When pedal point P is on a side of triangle ABC, pedal triangle RST is inside triangle ABC (point T is the same as point P). Here is an example.
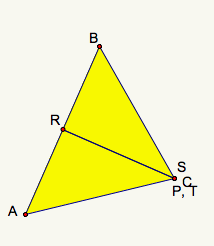
When pedal point P is one of the vertices of triangle ABC, we get a degenerate triangle since the three vertices of pedal triangle RST are collinear. This line segment is called the Simson Line. This is one condition in which the Simson Line can be created. Here is an example.
Click HERE to move pedal point P or the vertices of triangle ABC and observe the changes of pedal triangle RST.
Click HERE for a script tool for the general construction of a pedal triangle to triangle ABC where P is any point in the plane of ABC. Then you can move the vertices and/or pedal point P and observe the changes of pedal triangle RST. Can you find other conditions that will generate the Simson Line?
Click HERE to see that the pedal triangle of the pedal triangle of the pedal triangle of a point is similar to the original triangle. That is, show that the pedal triangle A'B'C' of pedal triangle RST of pedal triangle XYZ of pedal point P is similar to triangle ABC. Here is an example.

Notice that the ratio of corresponding sides are equal, so triangle A'B'C' is similar to triangle ABC.