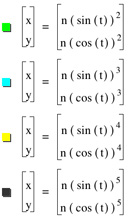In this investigation, we are going to explore parametric equations where x = nsin(t)a and y = ncos(t)b . In these cases, a and b are integers and n varies between -10 and 10. As well, t ranges from -5 to 5. In the initial instances of the investigation, I looked at the following: a,b=1, a=1 and b=2, a=2 and b=1, a,b=2, a,b=3, a,b=4, and a,b=5. Here are the matrices and what the graphs looked like. Remember that n varies.
After the first exploration, we can make some observations about the graphs. The blue, light blue, and black graphs seem to be complete. Where as the purple, red, green and yellow are not complete. Are there any conclusions we can make about these observations? Maybe not yet, but we can notice that the powers or exponents of the blue, light blue and black parametric equations are both odd. In the other cases, the powers or exponents are either even or one odd and one even. We may also notice that the larger the exponent, the less circular-shaped the graph becomes. As well, the larger the exponent, the more the graphs seem to flatten out and the "center" moves toward (0, 0). Let us now look at how we can complete the "incomplete" graphs. First we will look at the purple, where x = nsin(t) and y = ncos(t)2 . We need to add the part of the graph that is negative. We get the following.
Now we want to look at the red graph from the parametric equations x = nsin(t)2 and y = ncos(t). We want to complete the graph again, so we need to add the negative, such as in the first case. Here is what we got.
Now we will look at the case with the green graph where the parametric equations are x = nsin(t)2 and y = ncos(t)2 . In both equations, the exponent is even. We will need to add some other sets of equations to complete the graph. The following is what we got.
In the last case we will take the yellow graph where the parametric equations are x = nsin(t)4 and y = ncos(t)4 . Again, the powers of these equations are even numbers. We will then add more sets of equations to complete the graph. Here is what we got.
Lastly, we had said that the larger the exponent, the less circular the graph seemed to be. As well, the larger the exponent, the more the graph seemd to flatten out. Let's look at the case where the exponent is 21 for both parametric equations, so we have x = nsin(t)21 and y = ncos(t)21 . What do you think the graph with look like? For this specific case, I have zoomed in on the x- and y-axis to get a better view. You can imagine what you might see if the axes set as in the previous examples--probably not a whole lot. Here is the extreme case.
Return to main