Jernita Randolph

Pedal Triangles

Here we will construct a pedal triangle where the pedal point P is any point in the plane. Then we will explore the case when the pedal point P is the centroid of a given triangle.

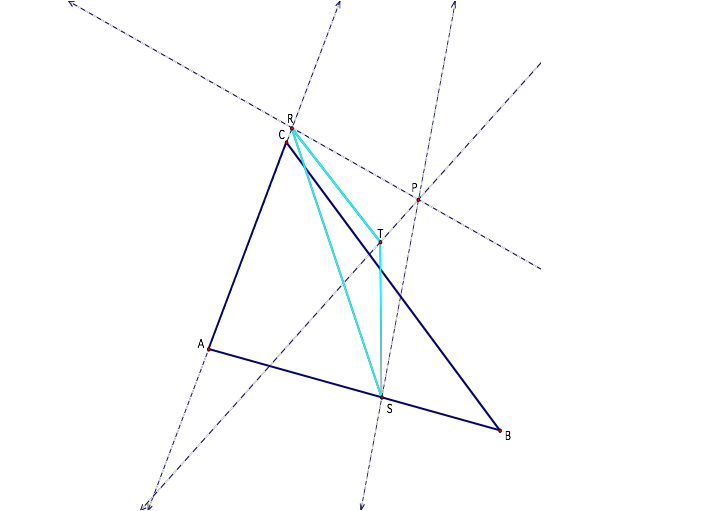
Suppose we have an arbitrary triangle ABC and let P be any point on the plane. By constructing the perpendicular lines from each side of triangle ABC to point P and calling the intersections of these lines points R, S, and T, we have constructed a pedal triangle. Namely, the triangle formed by points R, S, and T is the pedal triangle and P is the pedal point. In this case, we have P lying outside of triangle ABC.

Click here or on the figure to move the pedal point P and observe how the pedal triangle RST changes as these points are moved.

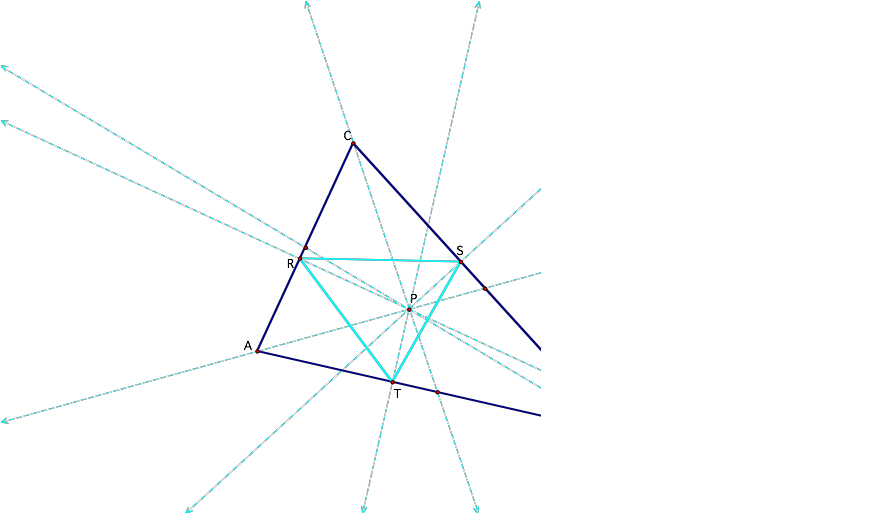
Now we will investigate the case when the pedal point P is the centroid of triangle ABC. The centroid is the common intersection of the three medians. By constructing the centroid of triangle ABC and letting it be the pedal point P, we can construct the pedal triangle about P.

Upon observation, we see that when the pedal point P is the centroid of triangle ABC, the pedal triangle RST lies inside triangle ABC. This is logical because the centroid is always located inside the triangle.

Click here or on the figure to move the pedal point P and observe how the pedal triangle RST changes as these points are moved when P is the centroid.

Return