
Quadratics in the xb plane
by Michael Walliser
Consider the equation x2 + bx + 1 = 0 (of the standard quadratic form ax2 + bx + c = 0). When we graph this relation in the xb plane, we get

Now if we take an arbitrary value of b, for instance b = 3, and overlay this equation on the graph, we get a line parallel to the x-axis, as shown below. Anywhere this line intersects our original curve corresponds to the roots of the original equation for that value of b.
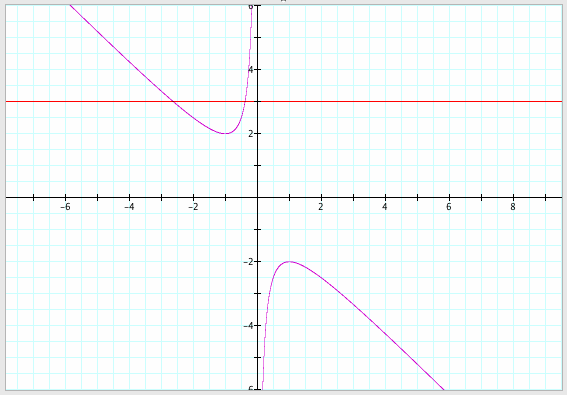
By observing the graph, it is easy to see that for all values b > 2, the line intersects the curve at two points to the left of the b-axis. Thus, the equation has two negative real roots. At b = 2, the line is tangent to the curve at a point to the left of the b-axis, so there is one negative real root. For values of b such that -2 < b < 2, the line does not intersect the curve, hence there are no real roots. When b = -2, the line is tangent to the curve at a point to the right of the b-axis, so there is one positive real root. For all values b < -2, the line intersects the curve at two points to the right of the b-axis. Thus, the original equation has two positive real roots.
Let's now consider the case for different values of c. The graph below shows x2 + bx + c = 0 in the xb plane for c = 1, c = 16, c = -1, c = -25, and c = 0.

Notice that for each c > 0, there is a unique positive value, let's call it u, which we can use to define the key characteristics of the curve. When b > u, there are two negative real roots. When b = u, there is one negative root. When -u < b < u, there are no real roots. When b = -u, there is one positive root. Finally, when b < -u, there are two positive roots. From observation, it looks like u is equal to two times the square root of c. Let's see if this is indeed the case.
If b is greater than twice the square root of c, then b2 is greater than 4c, and since a = 1, that means b2 - 4ac > 0. From the quadratic formula, we know that this means there are two real roots. We also know, since c is positive, then 4ac is positive, so b2 - 4ac is less than b2. Thus, the square root of b2 - 4ac is less than the square root of b2, or just simply b. It follows that -b + sqrt(b2 - 4ac) < 0, and obviously the converse is true as well, -b - sqrt(b2 - 4ac) < 0. Hence, if b is greater than two times the square root of c, then there are two negative real roots.
If b is equal to twice the square root of c, then x2 + bx + c can be factored into (x + sqrt(c))2. Therefore, x2 + bx + c = 0 has one real root, at x = -sqrt(c).
If b falls in the range -2sqrt(c) < b < 2sqrt(c), then b2 < 4ac, so from the quadratic formula, there are no real roots.
If b equals -2 times the square root of c, then x2 + bx + c can be factored into (x - sqrt(c))2. Therefore, x2 + bx + c = 0 has one real root, at x = sqrt(c).
If b is less than -2 times the square root of c, then b2 is greater than 4c, and since a = 1, that means b2 - 4ac > 0. Again using the quadratic formula, we know that this means there are two real roots. Like in the first case, since c is positive, then 4ac is positive, so b2 - 4ac is less than b2. Thus, the square root of b2 - 4ac is less than the positive square root of b2, which is -b, since b < 0. It follows that -b - sqrt(b2 - 4ac) > 0, and obviously the converse is true, -b + sqrt(b2 - 4ac) > 0.
So our postulate holds for all five cases when c > 0.
Now look at the cases where c < 0. We see that for every value of b, there is one positive real root and one negative real root. It looks also as though when b = 0, the roots are +/- sqrt(-c). Is this true?
Well, when b = 0, then x2 + bx + c = 0 becomes simply x2 + c = 0. We know that for any value t, x2 - t2 = (x + t)(x - t). Since c < 0, we conclude that yes, x2 + c = 0 can be factored into (x + sqrt(-c))(x - sqrt(-c)) = 0.
The final case to look at is c = 0. It would appear as though the roots are along the line b = -x. In other words, x2 + bx is equal to zero when b is equal to -x. If we factor out x, our equation becomes x(x + b) = 0. Our observation is correct, and it is also true that x = 0 is a root for all values of b.