
Tangent Circles
by Michael Walliser
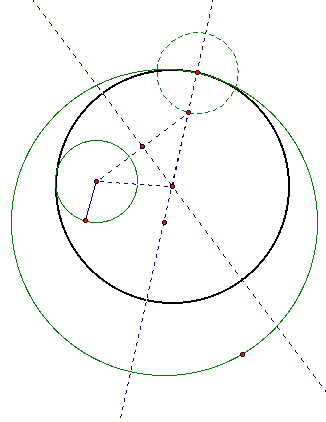
This lesson stems from the following problem: Given two circles and a point on one of the circles, construct a third circle that is tangent to both circles, and the tangency is at the given point on the circle on which it is defined. There are two solutions for a given pair of circles. One solution has the second circle outside the constructed tangent circle, and one solution has it inside. The constructions of these two tangent circles begin the same way. Our starting circles and given tangent point are shown below.

We know that to say Circle A is tangent to Circle B at a given point is equivalent to saying that Circle A is tangent to the tangent line of Circle B at that point. We also know that for a circle to be tangent to a line at a given point, the center must lie along the perpendicular through that point. Then, in order for two circles to be tangent at the same point, their centers must lie along the same line. Therefore, without drawing the tangent line, we can say that the center of our desired circle lies along the line defined by the center of the first circle and the point of tangency, as shown below.
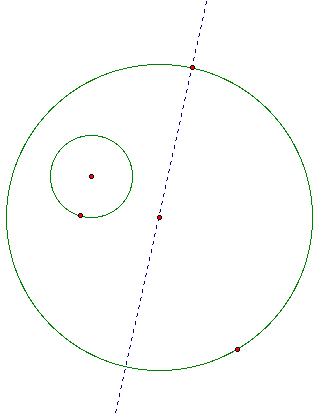
Here's where the constructions of the two solutions differ. First, we will consider the desired circle that is outside the second given circle. Think about the segment that runs from the center of our second circle to the center of our desired circle:
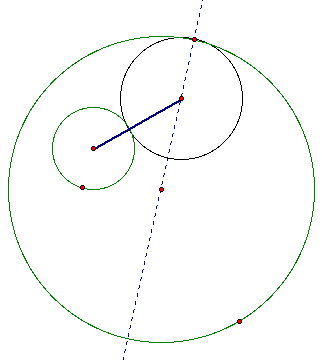
This segment intersects the two circles at their point of tangency, and its length equals the sum of the two radii. However, we don't know where the center of our desired circle is yet, other than the fact that it lies along the line defined by the center of the first given circle and the point of tangency. BUT, we know some stuff about isosceles triangles. We know that the center of our desired circle is the vertex of an isosceles triangle, and the other two vertices are the points of tangency on the two given circles. Therefore, one leg of the triangle is coincident with the segment described above, and the other leg is coincident with the line on which the center lies. We can't create this triangle yet, but we can create a triangle similar to it, with the same vertex. We must start by creating the base. In order for these triangles to be similar, we need to find a point along the dashed line above, which is beyond the tangent point by a distance equal to the radius of circle 2. We do this by making a circle whose center is at the point of tangency, with radius equal to that of circle 2, and marking off its exterior intersection with the line on which our center lies. The segment between this intersection and the center of circle 2 is our base.
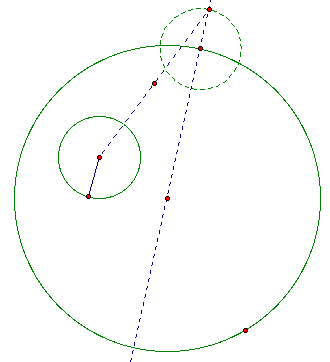
If we construct the perpendicular bisector to this segment, its intersection with the line on which the center lies creates the vertex of an isosceles triangle.
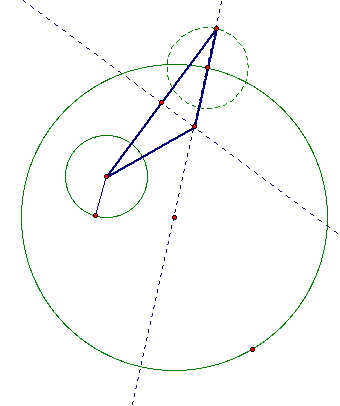
This triangle is similar to the one that defines our desired circle. Therefore, if we construct a circle whose center is at this intersection and passes through our desired point of tangency, we see that it is also tangent to the second given circle. Thus, we have our desired tangent circle.
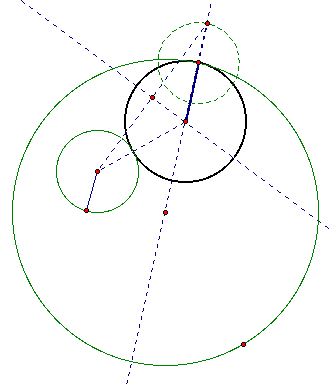
Now let's go back and construct the circle that is tangent to both given circles, but contains the second given circle in its interior. Start by thinking again about the segment that runs from the center of the second circle to the center of the desired circle.
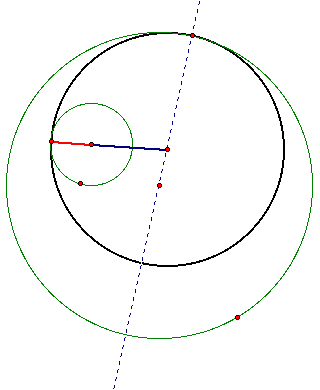
The length of this segment is the difference between the radius of the desired circle and the radius of the second given circle. Therefore, to create the base of our isosceles triangle, we will move along the same line, but since we are using subtraction, we will move toward the center of the first given circle.
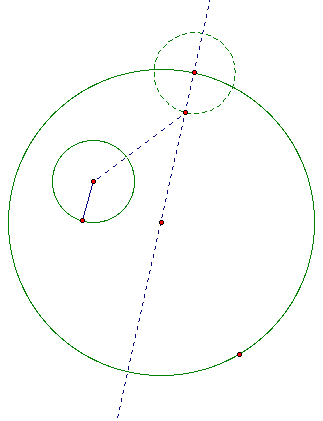
In the same manner as before, we construct an isosceles triangle using the base we just constructed, and the vertex is the intersection of the line on which our center lies and the perpendicular bisector of the base.
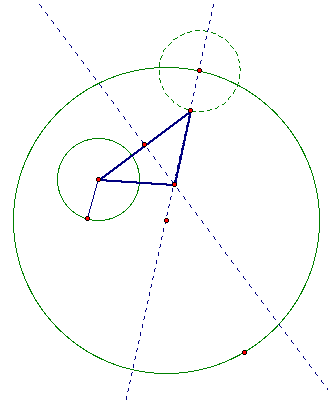
Just as before, the vertex of this triangle is the center of our desired circle. We can construct this circle with the knowledge that it passes through the given point of tangency.
Click here to open a GSP file with script tools that will construct each type of tangent circle given two circles and a point of tangency on the first circle.
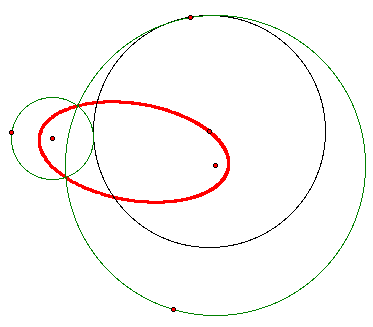
Let's examine the locus of the center of the tangent circle as we move the point of tangency around the first given circle. The shape of the locus depends on the relative positions of the given circles, so we will look at various cases. First, we'll consider the case as it was when we constructed the tangent circle, which is that one circle was inside the other circle.

We see that the locus is an ellipse, and that the foci are the centers of the given circle. We can also calculate that the radius of the ellipse is the sum of the radii of the two given circles. If we let d be the distance between the centers, r be the radius of the small circle, and R be the radius of the big circle, then consider the point on the locus where the tangent circle is smallest. We know that this point lies along the line defined by the circle centers, halfway between the circle edges. Therefore, we can calculate its distance from each circle as
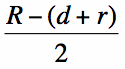
![]()
![]()
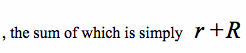
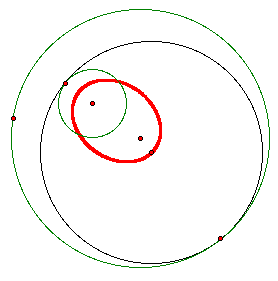
When we move the second circle out so that it intersects with the first circle, we see that nothing drastic happens. The locus now extends outside the first circle, but it is still an ellipse with the same foci and radius. We do see, however, that when the point of tangency moves inside the second given circle (as shown in the picture on the right), the tangent circle becomes externally tangent to the first circle and internally tangent to the second.
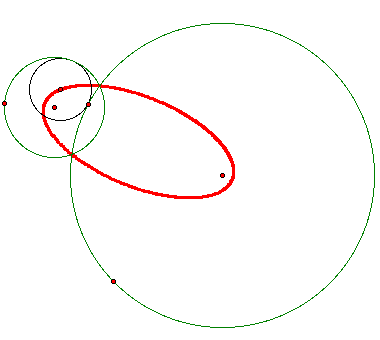
When we make the two given circles disjoint, we get a drastic change, as shown below.
Now the locus of centers is a hyperbola. Interestingly, the foci are the centers of the given circle, just as they were on the elliptical locus, and the constant difference is R + r, just as the radius (constant sum) is on the elliptical locus. Mathematically, we can derive that constant difference in a similar way to how we derived the radius of the ellipse, by considering the tangent circle whose center lies on the line defined by the centers of the given circles. The distance from this locus point to the center of the first given circle (focus 1) is

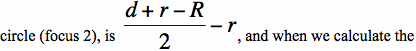

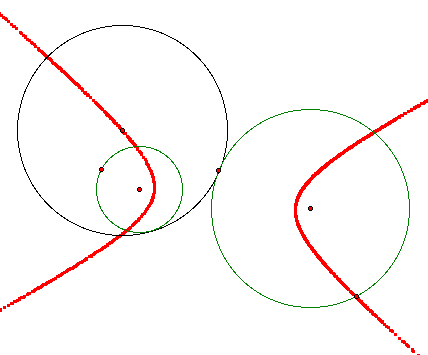
Next we'll examine the locus of centers for the second construction of the tangent circles, with the circles again varying from one inside the other, to intersecting, to disjoint. First, let's look at the case where one circle is inside the other.
We get an ellipse, just like with the other construction. The foci are again the centers of the given circles, but if we think about the difference in construction between these circles and the first set, it is easy to see why the radius of this ellipse is R - r instead of R + r. Next, let's look at the cases where the two given circles intersect and when they are disjoint.
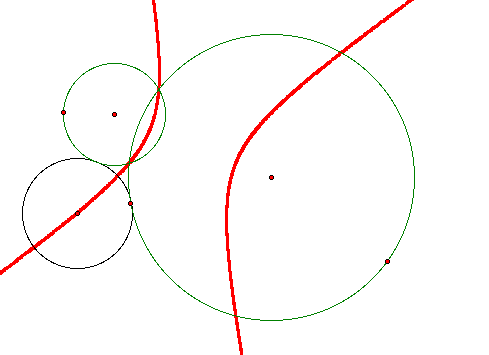

This time, the locus becomes a hyperbola when the circles intersect, and obviously stays that way as they move apart and become disjoint. The foci are still the centers of the two given circles, and as we could expect due to the difference in construction, the constant difference is R - r.