#13. The internal angle bisectors of triangle ABC are extended to meet the circumcircle at points L, M, and N, respectively. Find the angles of triangle LMN in terms of the angles A, B, and C. Does your result hold only for acute triangles?

To begin this problem, we need to construct the set-up given in the problem using GSP.

We start with a triangle ABC and its circumcircle.

Remember the circumcircle is constructed from any vertex and its center at the circumcenter of the triangle, constructed from the point of intersection of each side of the triangle's perpendicular bisector. You can also use the script tool here, from assignment 5 to construct your circumcircle.

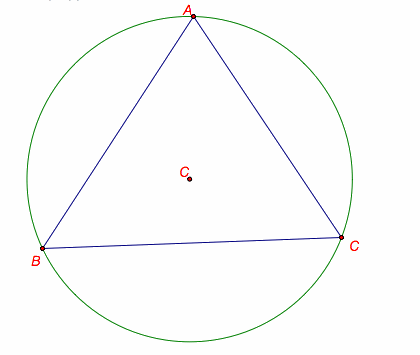
We begin with the above triangle and circumcircle.

Next, we want to construct the angle bisectors for the three angles of triangle ABC.

We want to construct a second triangle from the points of intersection of the circumcircle and the angle bisectors. Below, this triangle is in red and is label triangle LMN.

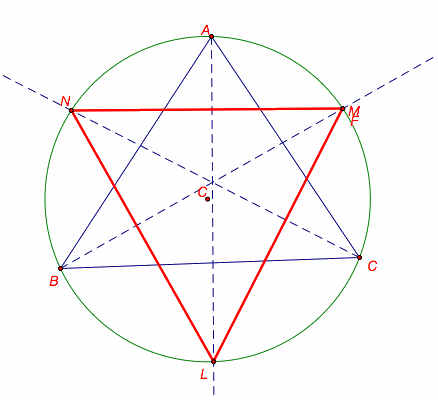
Now we have the appropriate set-up for our problem.

Click here for a GSP file with this construction.

We want to determine the measurements of the angles of the triangle LMN in terms of the angles of the triangle ABC.

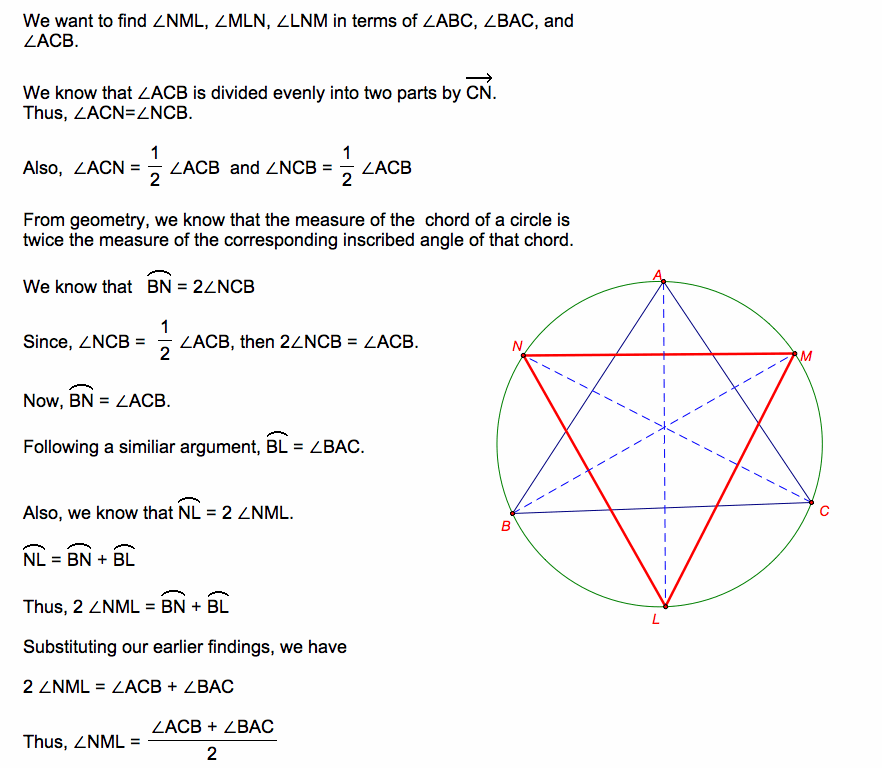
Solution

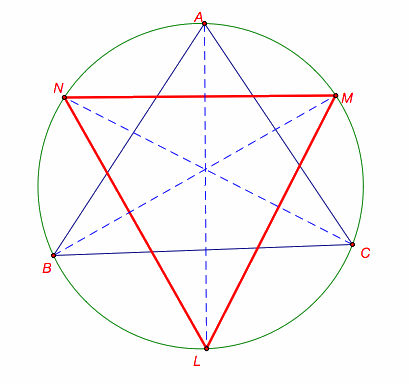
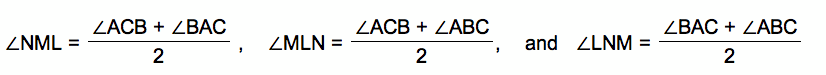
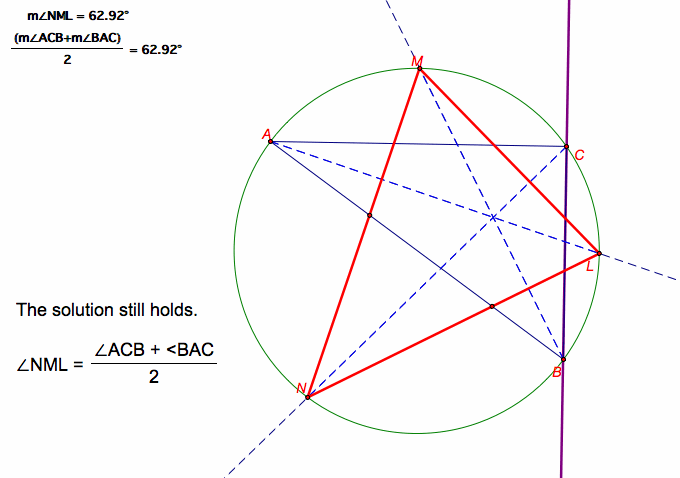
Does your result hold only for acute triangles?

Above, we could check our answer on GSP using the angle measurement command.

We can use the same command to see if our answer holds for ocute triangles and for right triangles.

First, we'll look at an obtuse triangle.

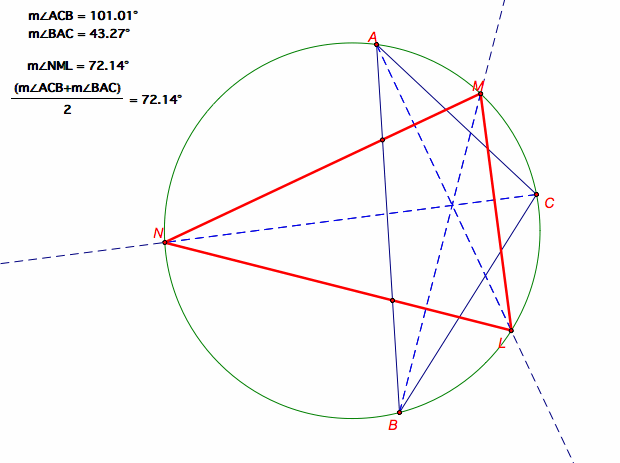
Next, we will see if our solution holds for a right triangle.

Return Home