
Brenda King
Altitudes and Orthocenters
Introduction:
When an orthocenter is
created for a triangle, it produces three smaller sub-triangles. The
orthocenter of these smaller triangles will be explored in this write up to see
how they are related to the original larger triangle.
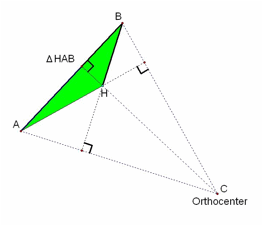
The ORTHOCENTER (H) of a
triangle is the common intersection of the three lines containing the
altitudes. An altitude is a
perpendicular segment from a vertex to the line of the opposite side.
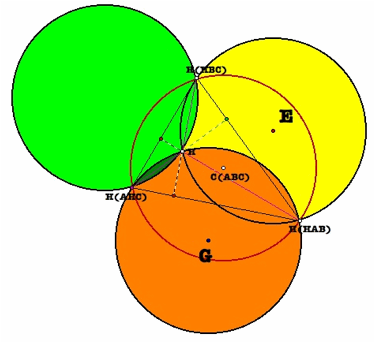
Also, the circumcircles of
triangle ABC, HBC, HAB and HAC will be constructed. The circumcircle for each triangle turns out to have the
same radii. They are all the same
size.
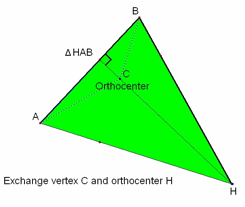
Exchanging vertices with
orthocenter H
Triangle ABC is constructed
with orthocenter H. Using this
construction, each vertex of triangle ABC is switched with orthocenter H. This is like moving the vertex of the
triangle to where the orthocenter H is located. The follow results are found
when exploring this change:
Orthocenter
of triangle HBC is vertex A
Orthocenter
of triangle HAC is vertex B
Orthocenter
of triangle HAB is vertex C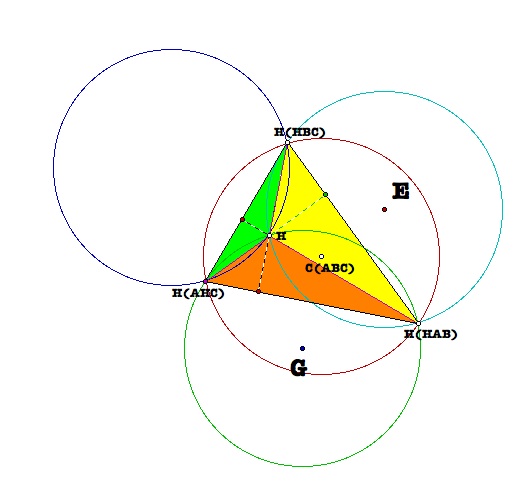
Specific example
Why does this result
happen? Looking at one specific
example, ∆HBC, gives some insight.
Since HA is an altitude of
∆ABC, it is perpendicular to BC and passes through vertex A. This
determines one of the altitudes of ∆HBC. The other two altitudes of
∆HBC are outside the triangle and can be found along sides AB and AC of
∆ABC. HB and HC pass through
vertex B and C and are perpendicular to side AC and AB (from construction of
orthocenter H). The common intersection of these three altitudes is vertex
A. See diagram 1 and 2 for this
case and diagram 3-6 for the other sub-triangles.
In a likewise manner the
orthocenter of triangle HAB and triangle HAC will be vertex C and vertex B,
respectively.
Diagram 1 Diagram
2

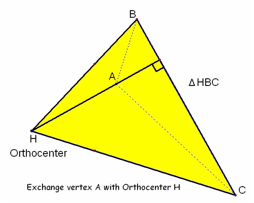
Diagram 3 Diagram
4
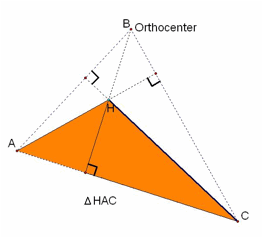
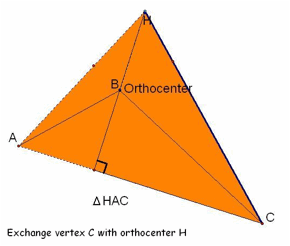
Diagram 5 Diagram
6
Circumcircle of triangle ABC,
HBC, HAB and HAC
Construct the circumcircle of ∆ABC and all sub-triangles.
The circles appear to have the same radius and all pass through the
orthocenter H. To check out this
conjecture, the circumcircle for a specific case will be reviewed. The specific case used is ∆HBC.
Specific example
The circumcenters for ∆ABC and ∆HBC is
constructed. The points E and F
are the center, both lie on the perpendicular bisector of BC, because they both
are equidistant from B and C. It
can be seen that the two circles have the same radii. In fact, all circumcircles produced from the sub-triangles
will be the same size.
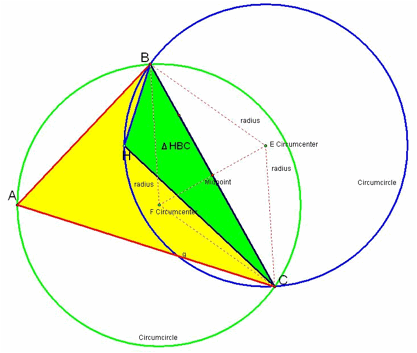
Point E and F are both equidistant from
BC and the angles at E and F both subtend the arc BC, therefore the angles are congruent.
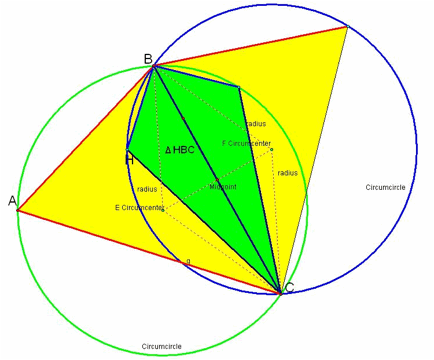
When ∆ABC and ∆HBC are
reflected about BC, they touch the opposite circumcircle.
Here is a sketchpad file with all the results pulled together (see several different tabs along bottom of file).