Assignment 4:
Centers of a Triangle
by
Wenjing Li
1. The CENTROID (G) of a triangle is the common intersection of the three medians. A median of a triangle is the segment from a vertex to the midpoint of the opposite side.
Use Geometer's Sketchpad (GSP) to Construct the centroid and explore its location for various shapes of triangles.
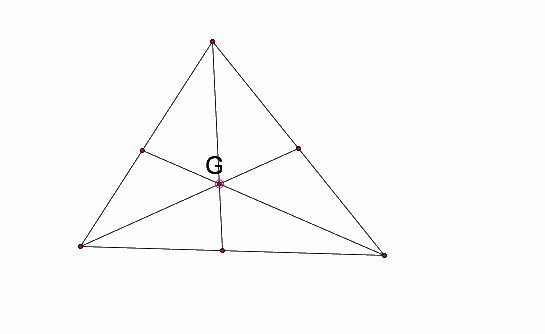
We draw a triangle, connect three vertexes to the midpoint of the corresponding opposite sides. The three medians intersect at a point G which is called the centroid of the triangle. We know that the centroid of the triangle is always inside the triangle no matter what shape of the triangle is.
2. The ORTHOCENTER (H) of a triangle is the common intersection of the three lines containing the altitudes. An altitude is a perpendicular segment from a vertex to the line of the opposite side. (Note: the foot of the perpendicular may be on the extension of the side of the triangle.) It should be clear that H does not have to be on the segments that are the altitudes. Rather, H lies on the lines extended along the altitudes.
Use GSP to construct an orthocenter H and explore its location for various shapes of triangles. (Make sure your construction holds for obtuse triangles.)

Let us draw a triangle and then draw three perpendicular line from the three vertexes to the corresponding lines of the corresponding opposite sides, and the three perpendicular lines from the three vertexes intersect at common point H, which is called the orthocenter of the triangle. Whether the orthocenter of the triangle is insides the triangle, or on one side of the triangle or outside the triangle depends on whether the triangle is acute triangle, or right triangle or obtuse triangle. For the above acute triangle, the othocenter is inside the triangle.
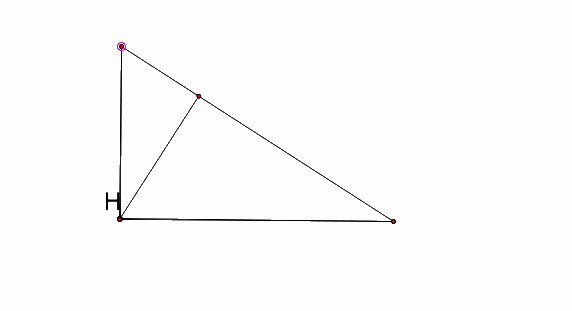
For the above right triangle, the othocenter is vertex for which it forms the vertex of the right angle of the triangle and it is on the side of the triangle.

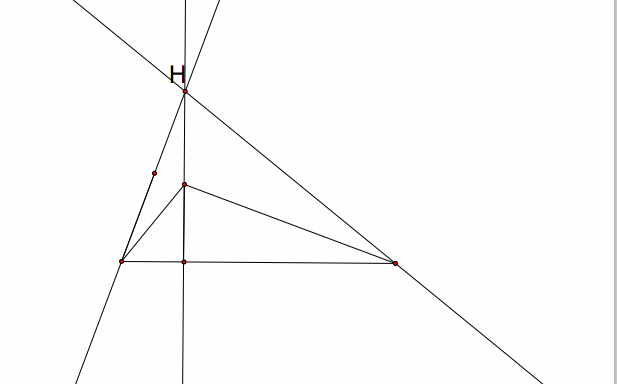
For the above obtuse triangle, the othocenter is outside the triangle.
3. The CIRCUMCENTER (C) of a triangle is the point in the plane equidistant from the three vertices of the triangle. Since a point equidistant from two points lies on the perpendicular bisector of the segment determined by the two points, C is on the perpendicular bisector of each side of the triangle. Note: C may be outside of the triangle.
Construct the circumcenter C and explore its location for various shapes of triangles. It is the center of the CIRCUMCIRCLE (the circumscribed circle) of the triangle.
We draw a triangle, then find the midpoint of one side and draw a perpendicular line through the midpoint of the side. The three perpendicular lines pass the three midpoints of the corresponding three sides and the three perpendicular line intersect at a common point C which is called the circumcenter. Since the point C has the same distances from the three vertex of the triangle, so if we draw a circle with the center C and radius the distance from the point C to one vertex, the triangle will inscribe this circle.
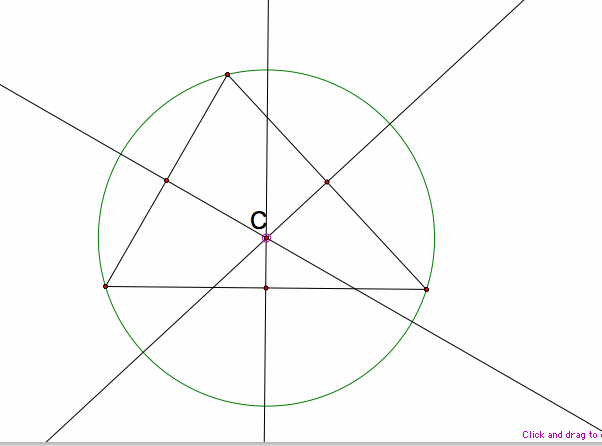
If the triangle is an acute triangle, then the circumcenter C is inside the triangle.
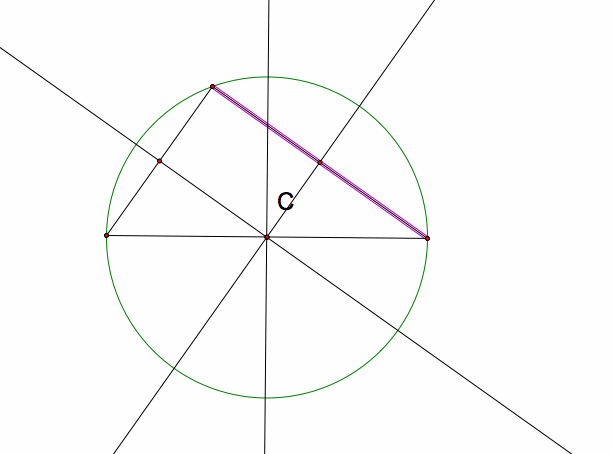
If the triangle is the right triangle, then the circumcenter C is on the side opposite to the right angle. The side opposite to the right angle is the diameter of the circle.
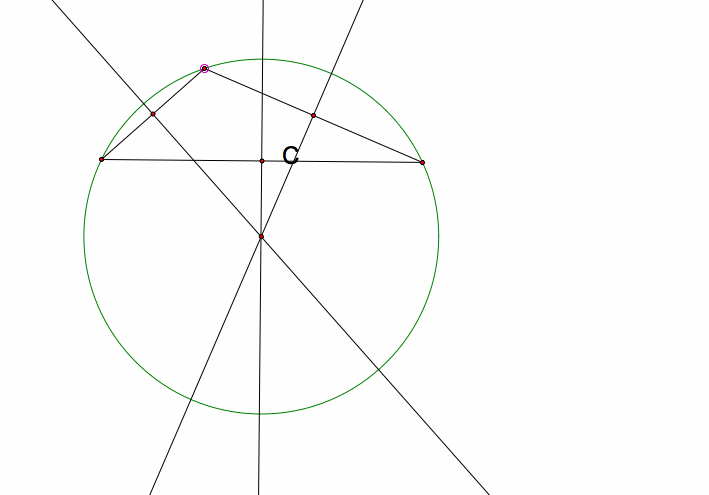
If the triangle is an obtuse triangle, then the circumcenter C is outside the triangle.
4. The INCENTER (I) of a triangle is the point on the interior of the triangle that is equidistant from the three sides. Since a point interior to an angle that is equidistant from the two sides of the angle lies on the angle bisector, then I must be on the angle bisector of each angle of the triangle.
Use GSP to find a construction of the incenter I and explore its locationfor various shapes of triangles. The incenter is the center of the INCIRCLE (the inscribed circle) of the triangle.
If the triangle is an acute triangle, then the incenter I is inside the triangle.
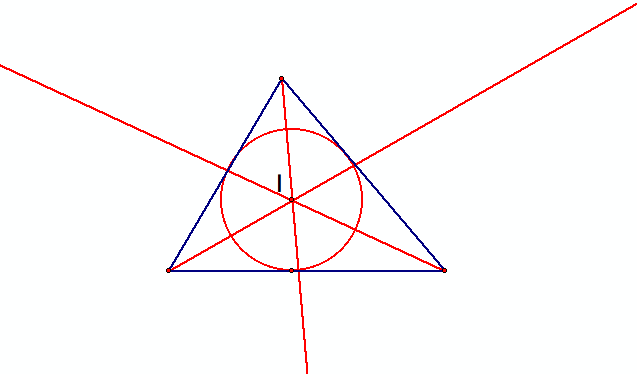
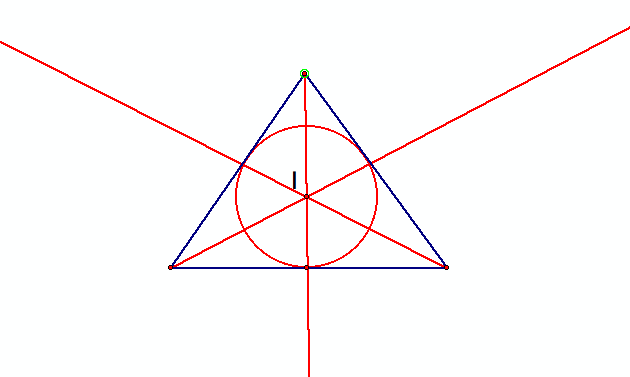
If the triangle is an obtuse triangle, then the incenter I is inside the triangle.
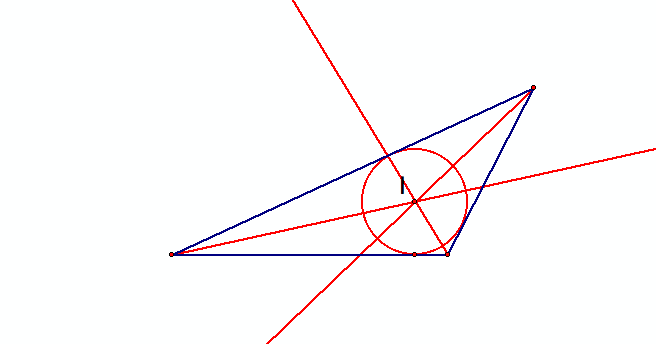
We explore the location for various shapes of triangles and found that the incenter I is always inside the triangle.
5. Use GSP to construct G, H, C, and I for the same triangle. What relationships can you find among G, H, C, and I or subsets of them? Explore for many shapes of triangles.
We observe that H, G, C are always on a same line no matter what the shape of the triangle is. In general I does not lie on this line. If the triangle is an acute triangle, then G, H, C, and I are all inside the triangle.
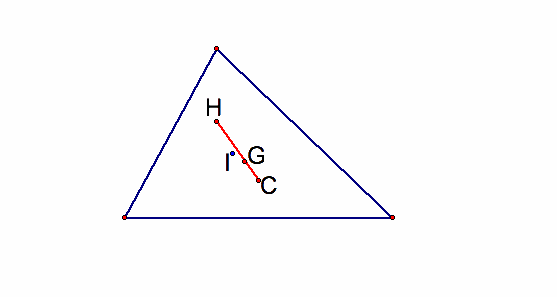
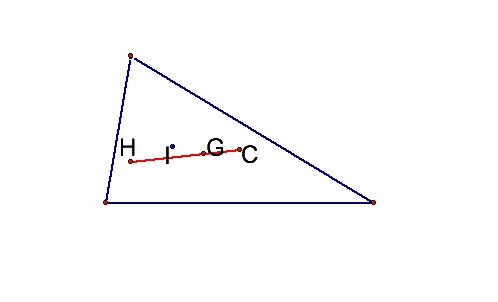
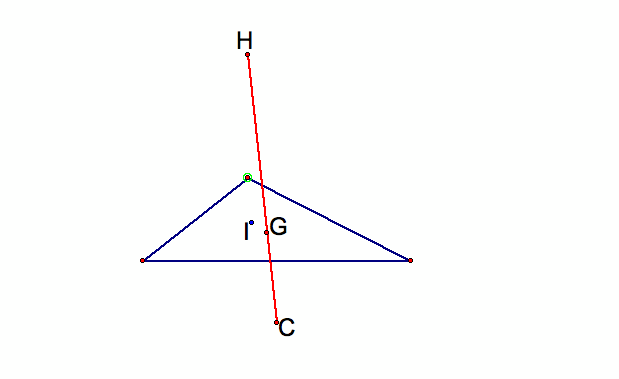
If the triangle is a right triangle, then G and I areinside the triangle, H is the same as one vertex B and Cis the midpoint of AC.
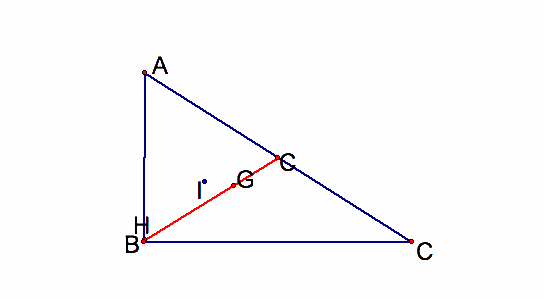
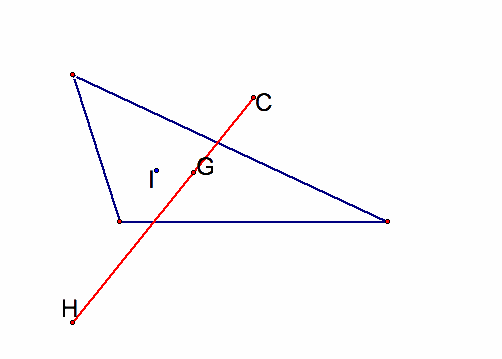
If the triangle is an obtuse triangle, then G and I is inside the triangle and H, C are outside the triangle.
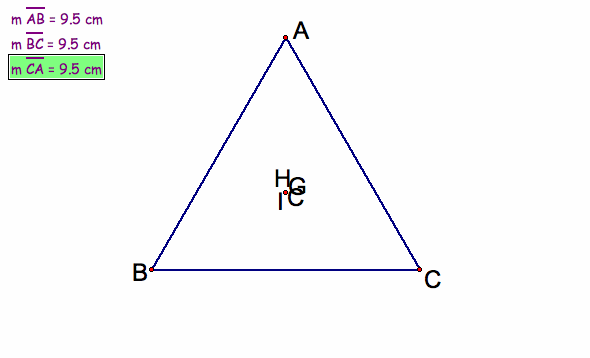
If the triangle is an equilateral triangle, then G, I, H, C are the same point and all inside the triangle.