

Assignment 9:
Pedal Triangles
by
Weaning Li
1a. Let triangle ABC be any triangle. Then if P is any point in the plane, then the triangle formed by constructing perpendiculars to the sides of ABC (extended if necessary) locate three points R, S, and T that are the intersections. Triangle RST is the Pedal Triangle for Pedal Point P.
We construct any triangle ABC and choose any point P in the plane. Then we draw the three lines perpendicular to the three sides AB, BC, AC and get the three intersection points R, S, T. The three intersection points R, S, T form a triangle RST which is the pedal triangle for the pedal point P. If the pedal point P is located outside the triangle ABC, then the situation is the following yellow pedal triangle RST which is outside the triangle ABC.
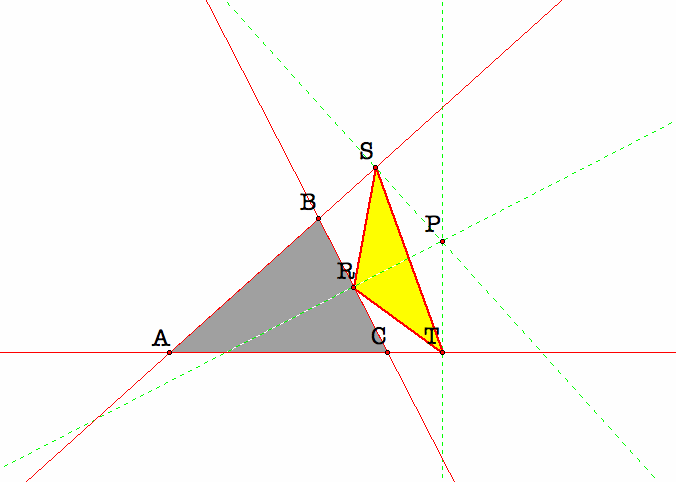
If the pedal point P is located inside the triangle ABC, then the situation is the following yellow pedal triangle RST which is inside the triangle ABC.
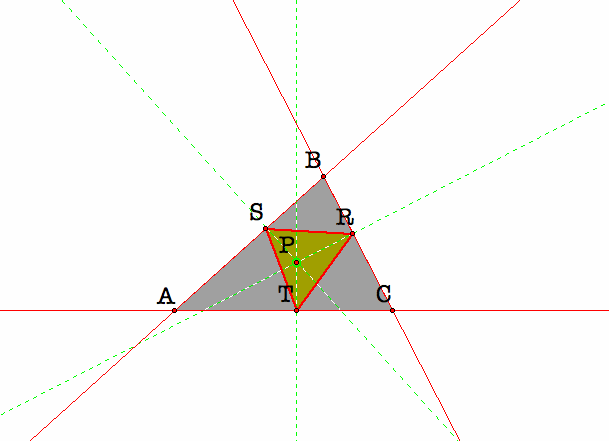
1b. Use GSP to create a script tool for the general construction of a pedal triangle to triangle ABC where P is any point in the plane of ABC.
We use the GSP software to create a script tool for constructing the pedal triangle of the given triangle ABC and any given pedal point P on the plane. Please try our following script tool.
Given triangle ABC and a pedal point P, we construct the pedal triangle XYZ of the triangle ABC, then construct the pedal triangle RST of the triangle XYZ through the pedal point P, and similarly we construct the pedal triangle A'B'C' of the triangle RST through the pedal point P. The following is our construction.
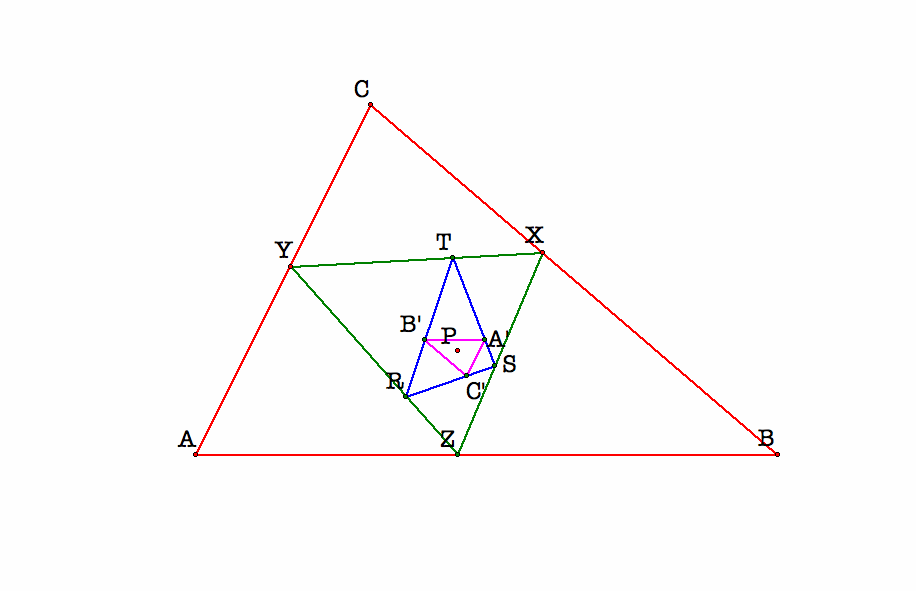
2. What if pedal point P is the centric of triangle ABC?
Let us investigate what will happen if the pedal point P is the centric of triangle ABC. Recall the CENTROID of a triangle is the common intersection of the three medians. A median of a triangle is the segment from a vertex to the midpoint of the opposite side.

If the pedal point P is the centric of the triangle ABC, then the pedal triangle is in the interior of the triangle ABC and it is always inside of the triangle ABC which will not be out the triangle ABC.
3. What if . . . P is the incenter . . . ?
We investigate what will happen if the pedal point P is the incenter of triangle ABC. Recall the INCENTER (I) of a triangle is the point on the interior of the triangle that is equidistant from the three sides. The incenter is always on the angle bisector of each angle of the triangle since a point interior to an angle that is equidistant from the two sides of the angle lies on the angle bisector.
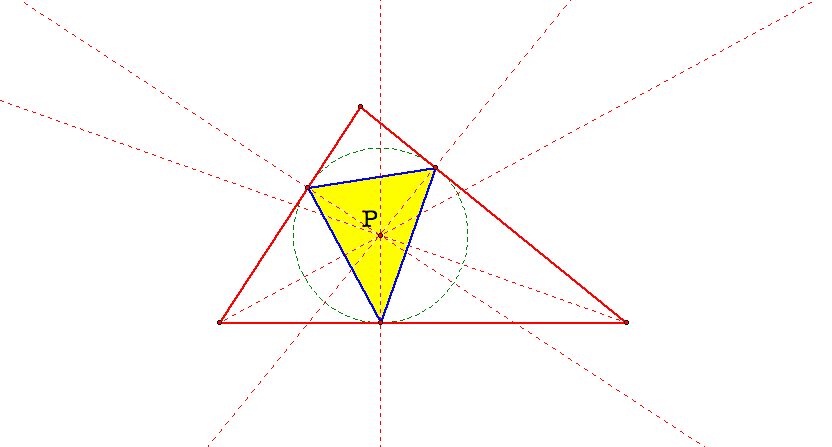
If the pedal point P is the incenter of the triangle ABC, then the pedal triangle is in the interior of the triangle ABC and it will always inside of the triangle ABC which will not be out the triangle ABC.
4. What if . . . P is the orthocenter . . . ? Even if outside ABC?
We investigate what will happen if the pedal point P is the Orthocenter of triangle ABC. Recall the ORTHOCENTER (H) of a triangle is the common intersection of the three lines containing the altitudes and an altitude is a perpendicular segment from a vertex to the line of the opposite side. The foot of the perpendicular may be on the extension of the side of the triangle. The othocenter may not be on the segments that are the altitudes but it lies on the lines extended along the altitudes. If the pedal point P is located inside the triangle ABC, then the pedal triangle is the the same as the Orthic triangle which is inside the triangle ABC. Recall that given a triangle ABC, the triangle RST whose vertices are endpoints of the altitudes from each of the vertices of ABC is called the orthic triangle, or sometimes the altitude triangle.
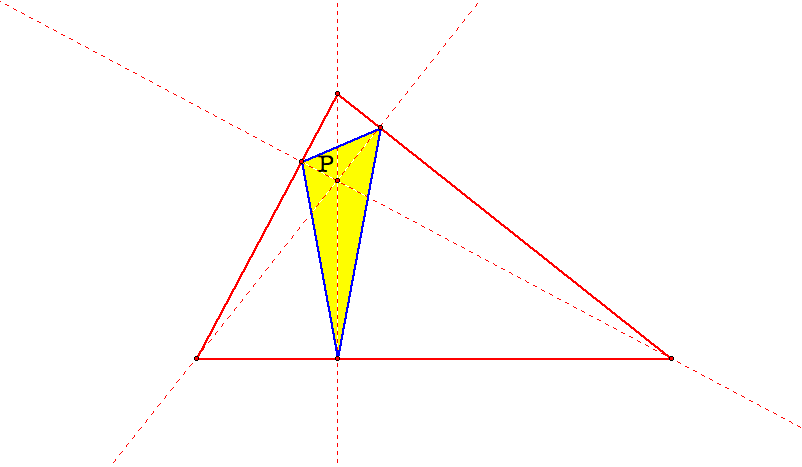
If the pedal point P is located outside the triangle ABC, then the yellow pedal triangle is the the same as the Orthic triangle some part of which is outside the triangle ABC as shown in the following picture.
If the pedal point P is the Othocenter, then the pedal triangle is the same as the Orthic triangle.
If the pedal point P is the Orthocenter of the triangle ABC, then the pedal triangle can be either inside or outside the triangle ABC depend on where the pedal point is located. If the triangle is an acute triangle, then the pedal triangle is inside the triangle. If the triangle is an obtuse triangle, then the pedal triangle is outside the triangle.
5. What if . . . P is the Circumcenter . . . ? Even if outside ABC?
Let us investigate what will happen if the pedal point P is the Circumcenter of triangle ABC. Recall the CIRCUMCENTER (C) of a triangle is the point in the plane equidistant from the three vertices of the triangle. The circumcenter is on the perpendicular bisector of each side of the triangle because a point equidistant from two points lies on the perpendicular bisector of the segment determined by the two points. The circumcenter may be outside of the triangle.

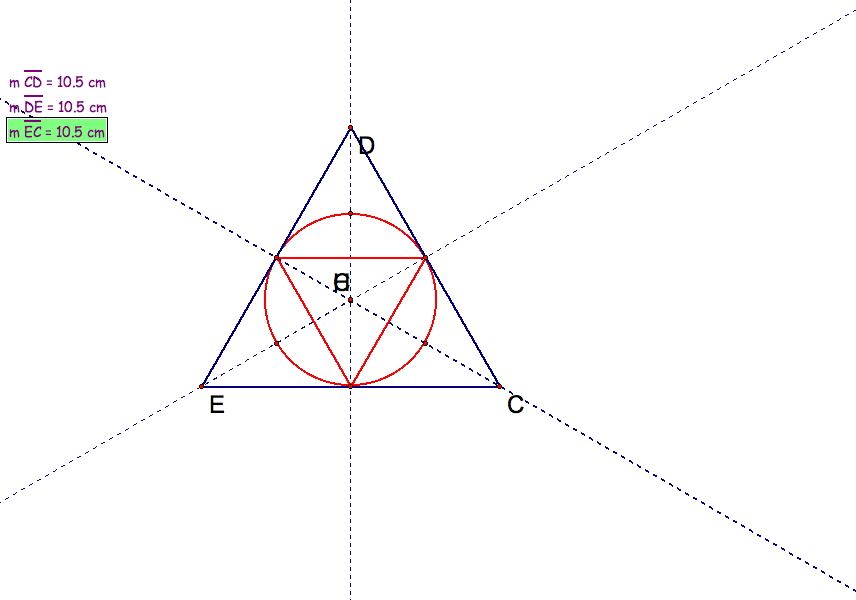
If the pedal point is the circumcenter, then the pedal triangle is the same as the medial triangle. Recall that the medial triangle is formed by joining the midpoints of the sides of a triangle ABC.
6. What if . . . P is the Center of the nine point circle for triangle ABC?
Now we investigate what will happen if the pedal point P is the nine point circle of triangle ABC. Recall the nine-point circle is a circle that can be constructed for any given triangle. It is so named because it passes through nine significant points, six lying on the triangle itself unless the triangle is obtuse. The nine significant points include the three midpoints of each side of the triangle, the three foots of each altitude and the three midpoints of the segment of each altitude from its vertex to the orthocenter where the three altitudes meet.
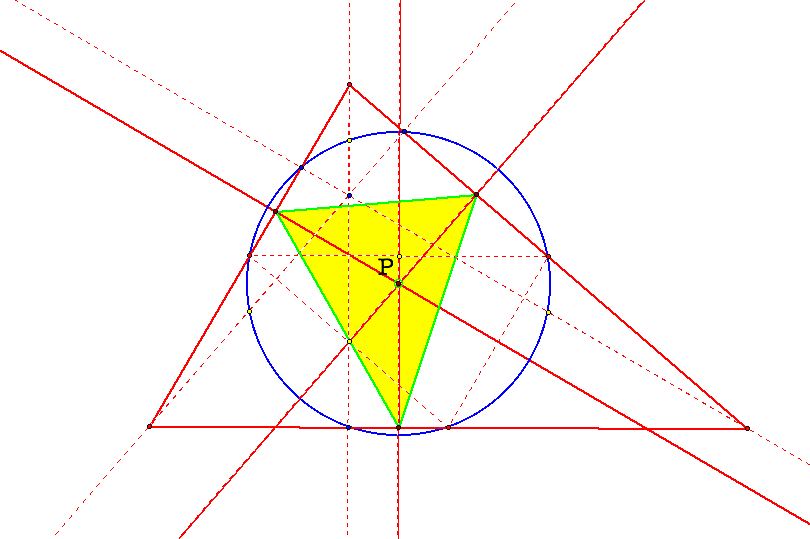

We investigate whether the pedal triangle is always on the nine point circle. When the triangle ABC is an equilateral triangle, then the circumcenter C, the othocenter H and the center of the nine point circle coincide and it is the pedal point as well. The pedal triangle lies on the nine point circle.
7. What if P is on a side of the triangle?
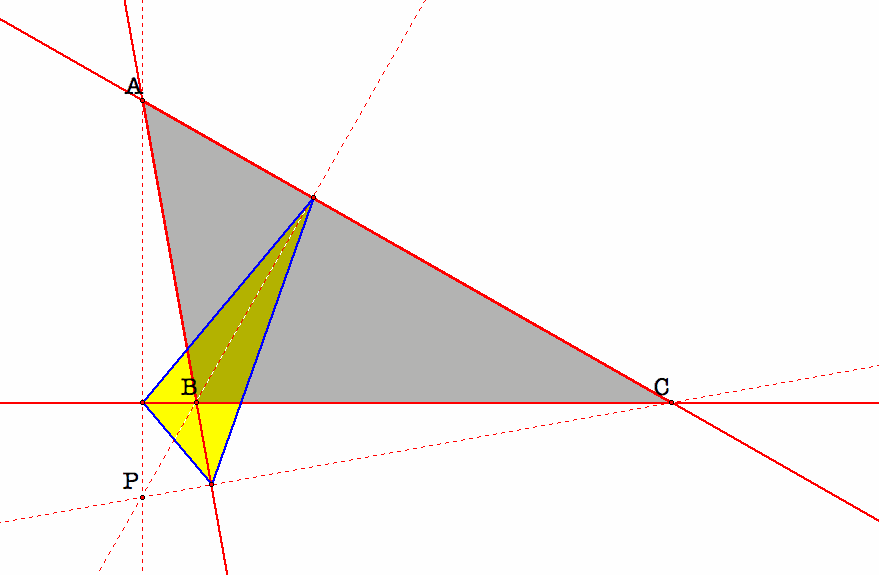
If the pedal point P is on one side AC of the triangle ABC, we have the following yellow pedal triangle.

We observe that if the pedal point P is on a side of the triangle ABC, then P is one of the vertices of the pedal triangle.
8. What if P is one of the vertices of triangle ABC?
If the pedal point P is one of the vertices of the triangle ABC say the vertices B, then the situation is as shown in the following picture.
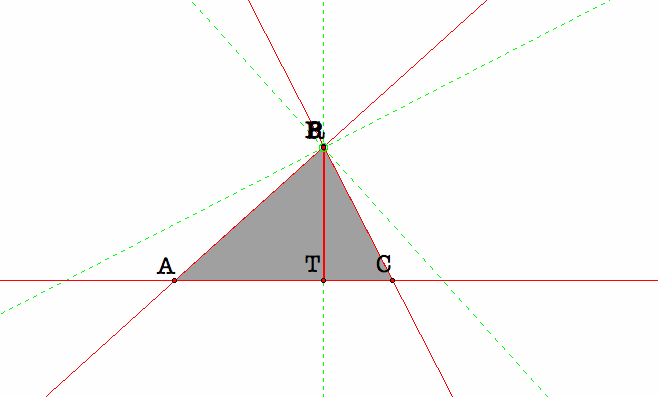
We see that if the pedal point P is one of the vertices of the triangle ABC, then the pedal triangle will become a line.
9. Find all conditions in which the three vertices of the Pedal triangle are collinear (that is, it is a degenerate triangle). This line is called the Simpson Line.
We want to investigate under what condition the three vertices of the Pedal triangle are collinear.

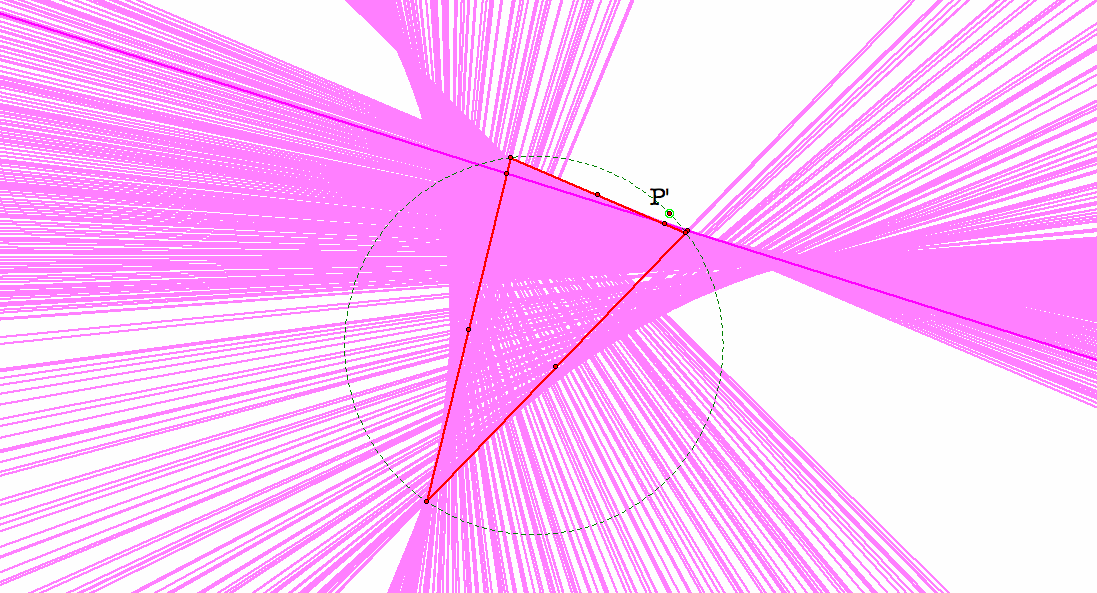
We found that if the pedal point P' is on the circumscribe of the triangle ABC, then the three vertices of the Pedal triangle are collinear and it is a line which is the pink line in our above picture and it is called Simpson line. We trace the envelope of the Simpson line as the Pedal point is moved along the circumscribe and get the above tracing envelope.