
Final Project: Part I --
Bouncing Barney
by
Kristina Little

Final Project: Part I --
Bouncing Barney
by
Kristina Little
Barney is in a triangular room shown here. He walks from a point on BC parallel to AC. When he reaches AB, he turns and walks parallel to BC. When he reaches AC, he turns and walks parallel to AB.
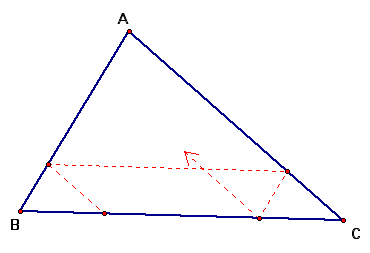
We would like to know if Barney always returns to his starting point. Look at this GSP file to see the written proof of Barney's path.
Now we know that Barney will always return to his starting point, we can see how many times Barney will "bounce" off the walls before reaching his starting position again.
 |
 |
 |
|---|---|---|
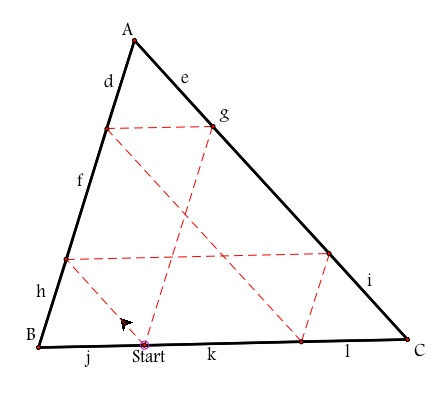
When Barney starts at any arbitrary point along, BC he will bounce five times before returning to his original starting point. |
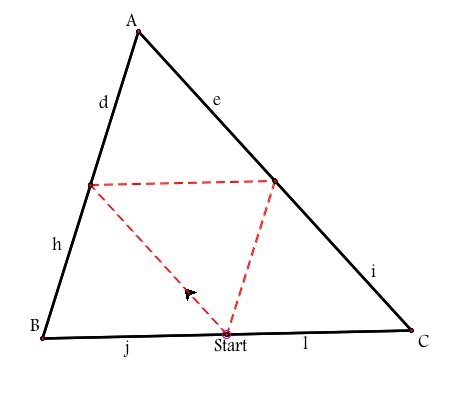
If Barney were to start at the midpoint of BC, then he will only bounce twice before returning to his starting point. |
On the rare chance, that Barney starts on the very edge of the room, he will also only hit the walls twice before returning to the beginning. |
Another question then arises, are these triangles that Barney makes inside the room similar?
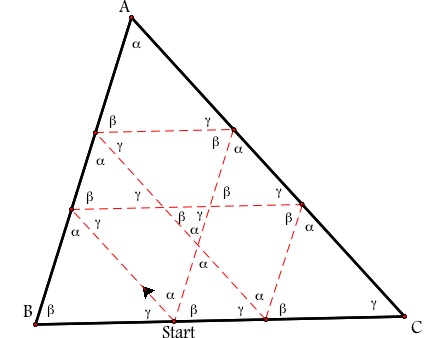
We can see based on the properties of parallel lines and alternate angle theorem that we do have a group of similar triangles. Only where we have trapezoids instead of triangles, do we have a small gap where we do not know as much about those angles.
Then we would like to know about when the triangles are also congruent to one another. What starting points give us these situation where the triangles are not only similar but congruent.
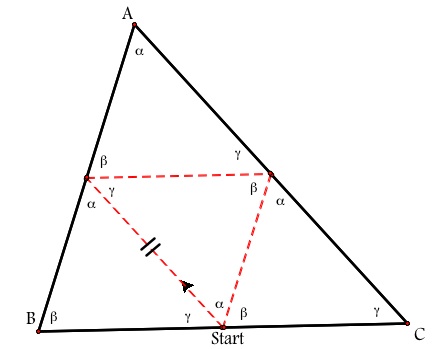
Using the angles that we were able to find above, along with the property that a line segment is congruent to itself, we are able to show that all the triangles inside triangle ABC are congruent to one another.
We only have two ways the smaller triangles are all congruent to each other. When the starting point is any other point along BC that the parallel paths cut not only triangles but also trapezoidal type figures. Only the midpoint and the trisection points give us all triangles inside the triangular room.
Starting at an arbitrary point inside the triangular room, instead of along BC, we see that Barney travel along much of the same similar paths as if he started on the line segment BC. The only significant difference is that is Barney start at an interior point then he will have to bounce off the wall one more time in the first two conditions above to return to his starting point. Also, starting inside of the room will eliminate the ability for Barney to walk around the edges of the room.
The next question would be does Barney always travel the same distance?
If Barney starts outside of the triangle it is easy to see that Barney is traveling much further along each given side the further outside of the room he starts. Therefore, the distance Barney travels will follow directly by what length his starting point is outside of segment BC.
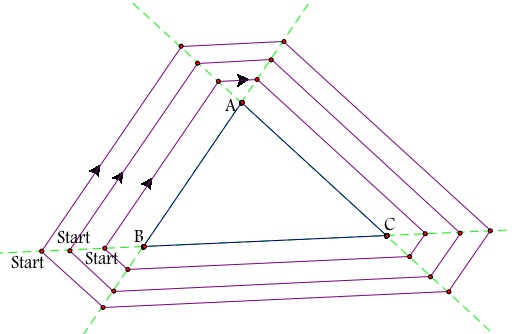
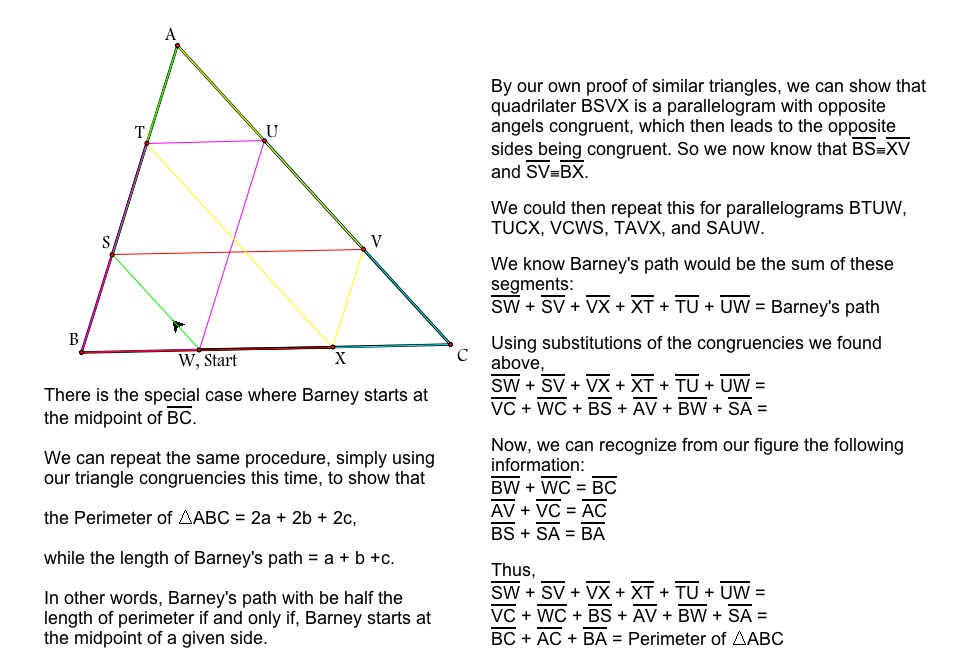
If Barney starts inside of the room, we can say that he will always travel the same distance except if he starts exactly at the midpoint of the segment BC. Follows is the proof.
Final Project: Part II -- Animated Pedal Points