

Assignment 4: The Nine-Point Circle
Ebru Ersari
The Nine-Point circle for any triangle passes through the three mid-points of the sides, the three feet of the altitudes, and the three mid-points of the segments from the respective vertices to orthocenter. Construct the nine points, locate the center (N) and construct the nine point circle.
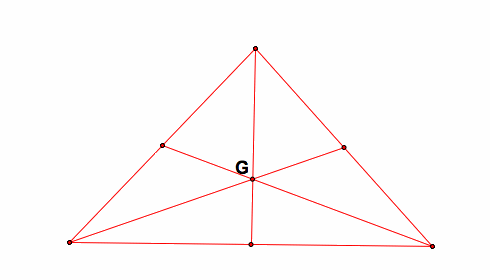
For constructing the nine-point circle, first I found three midpoints of the sides. When we draw the segment from a vertex to the midpoint of the opposite side, it gives us the median. The intersection of three medians in a triangle gives us the Centroid (G). Here is the centroid of a triangle.
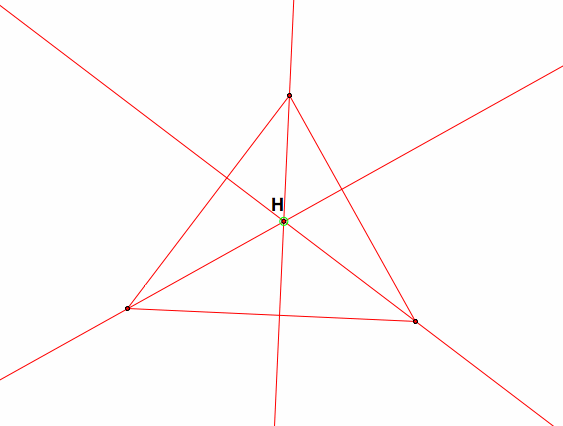
Next, I drew three perpendicular segments from each vertex to the line of the opposite sides. These three perpendicular segments give the attitudes. The intersection of three attitudes in a triangle gives us the ORTHOCENTER (H). Here is the orthocenter of a triangle.
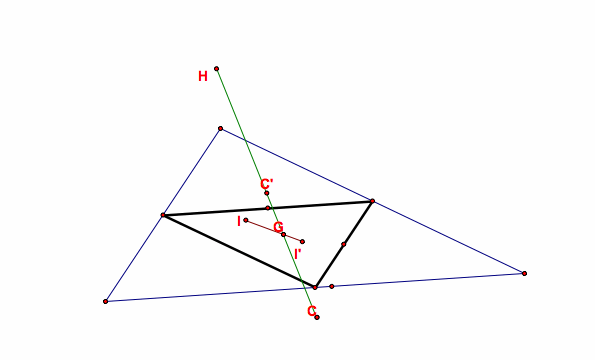
I have already found the midpoints of each side. Then, I drew 3 lines perpendicular to each side passing through these midpoints. The intersection of these three lines give us the CIRCUMCENTER (C). The distance between the circumcenter and three vertices are equal. The circumscribed circle centered by the circumcenter is the CIRCUMCIRCLE. Here is the circumcenter of a triangle and circumcircle.

Later, I drew the bisectors of each angle of the triangle. The intersection of these three bisectors is the INCENTER (I) of the triangle. The inscribed circle centered by the incenter is the INCIRCLE. Here is the incenter of a triangle and incircle below.

When we construct G, H, C and I for the same triangle, G, H, and C are on the same line.

For any triangle, a triangle connecting the three midpoints of the sides is the MEDIAL triangle.
The Relation Between the Triangle and Its Medial Triangle:
The medial triangle’s area is one fourth the original triangle’s area.
The centroid of these two triangles is the same.
The circumcenter of the original triangle and the orthocenter of the medial triangle are the same point.
For any acute triangle, the triangle that connects the feet of the altitudes is the ORTHIC triangle.
The Relation Between The Triangle and Its Orthic Triangle:
The orthocenter of the original triangle and the incenter of the orthic triangle are the same point.
For any acute triangle ABC, first we constructed the orthocenter (H) of the triangle and the segments HA, HB, and HC. Next, we constructed the midpoints of HA, HB, and HC. Then, we constructed the midpoints to get a triangle (KLM triangle).
When we check the angles,
![]() CAB =
CAB = ![]() MKL
MKL
![]() BCA =
BCA = ![]() LMK
LMK
![]() ABC =
ABC = ![]() KLM
KLM
From AAA similarities, triangle ABC and triangle KLM are similar triangles.
AH = 2 AK = 2 HK
BH = 2 BL = 2 KL
CH = 2 CM = 2 HM
The similarity ratio:
AH / HK=BH / KL = 2
Thus,
AB / KL=BC / LM=AC / KM = 2
Then, the similarity ratio between the triangle ABC and KLM is 2.
When we check the angles of the triangles KLM and OPN,
![]() NOP =
NOP = ![]() MKL
MKL
![]() PNO =
PNO = ![]() LMK
LMK
![]() OPN =
OPN = ![]() KLM
KLM
From AAA similarities, triangle KLM and OPN are similar triangles. Since OPN is the medial triangle, the similarity ratio between the triangle ABC and OPN is 2. We also know that the similarity ratio between the triangle ABC and KLM is 2. Thus, the similarity ratio between OPN and KLM is 1 and these two triangles are congruent triangles.
Both the original triangle ABC and KLM have the same orthocenter.
The circumcenter of the triangles KLM and OPN is the same point.
We already knew that the original triangle and the medial triangles’ circumcenter (C) is the same point. Also, the original triangle’s circumcenter (C)and the medial triangle’s orthocenter (H) are the same point.
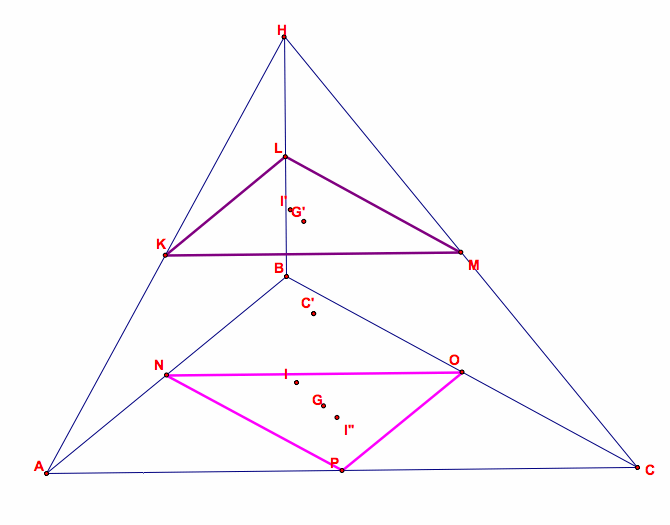
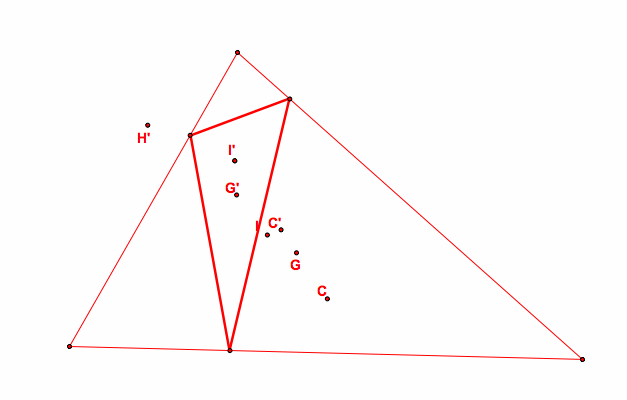
I took the same original triangle and constructed the three secondary triangle (medial triangle, orthic triangle and the triangle from the previous example). When I constructed the circumcenter for each of the secondary triangles, I saw that all three triangles have the same circumcenter.
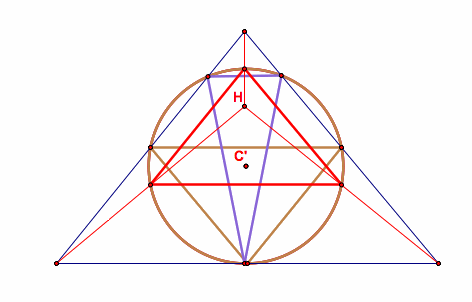
I constructed the nine points: the three mid-points of the sides, the three feet of the attitudes, and the three mid-point of the segments from the respective vertices to ortocenter.
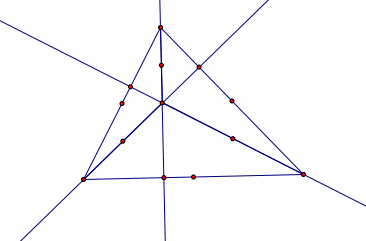
For constructing the nine-point circle, I first needed to find the center. For finding the center, I connected the midpoints of the original triangle and got a new triangle.
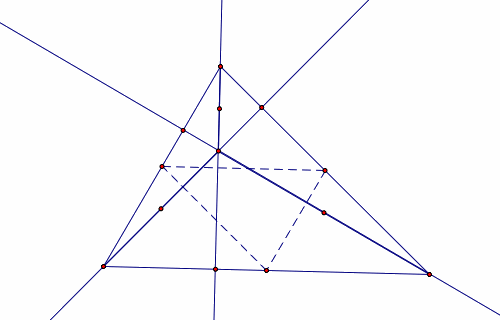
Then, I found the intersection point of the perpendicular bisector of the new triangle. This intersection point is the center of the nine-point circle.

Here is the nine-point circle:
