Assignment 4

The Mid-Segment Triangle

by

Kaitlin Spooner

Take an acute triangle ABC. Construct H and the segments HA, HB, and HC. Construct the midpoints of HA, HB, and HC. Connect the midpoints to form a triangle. Prove that this triangle is similar to triangle ABC and congruent to the medial triangle. Construct G, H, C, and I for this triangle. Compare.

We will first prove that mid segment triangle DEF is similar to triangle ABC. Construct a line through A and H. Then construct a line parallel to line AH through the point B. Construct a line through points D and E, and construct point N at intersection of the line through B and the line DE. This line is a transversal of the parallel lines AH and BN. Extend segment AB to form a line through the two points to make it a transversal through the two parallel lines as well. By alternate interior angles, angle EDH is equal to angle ENB. By corresponding angles, angle ENB is equal to angle ABO. Then by the transitive property, since angle EDH is congruent to ENB and angle ENB is congruent to ABO, angle EDH is congruent to angle ABO. Next, angle ABO is congruent to angle BAD since they are alternate interior angles. Finally, angle EDH is congruent to angle BAD by the transitive property.

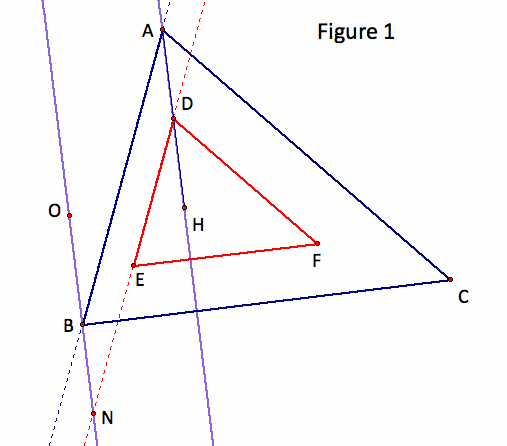
We have now shown that angle EDH is congruent to angle BAD. We must now show that angle FDH is congruent to angle DAC. We will go through the same process of extend lines as we did in the previous section. Construct a line parallel to line AH through the point C. Construct a line through points D and F, and construct point P at intersection of the line through C and the line DF. This line is a transversal of the parallel lines AH and CQ. Extend segment AC to form a line through the two points. It is also a transversal through the two parallel lines. By alternate interior angles, angle FDH is equal to angle FPC. By corresponding angles, angle FPC is equal to angle ACQ. Then by the transitive property, since angle FDH is congruent to FPC and angle FPC is congruent to ACQ, angle FDH is congruent to angle ACQ. Next, angle ACQ is congruent to angle DAC since they are alternate interior angles. Finally, angle FDH is congruent to angle DAC by the transitive property.

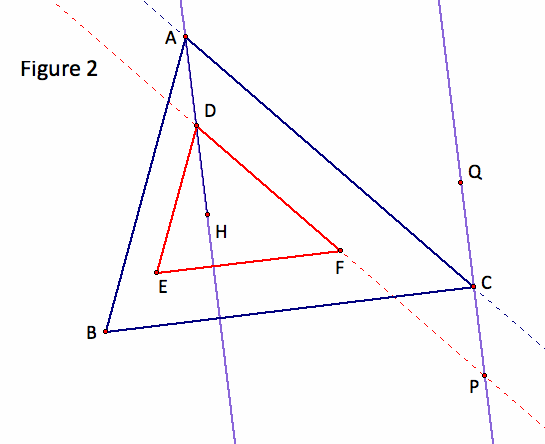
We now know that angle EDH is congruent to angle BAD and angle FDH is congruent to angle DAC. Each of these is only part of an interior angle in each triangle, and we want to show an entire interior angle in the original triangle is equal to an entire angle in the mid segment triangle. In our original triangle, angle BAC is equal to angle BAD plus angle DAC. Similarly, in our mid segment triangle, angle EDF is equal to angle EDH plus angle FDH. By using the conclusions we came to in the two previous sections, we can conclude:

BAC = BAD + DAC = EDH + FDH = EDF

Therefore, angle BAC is congruent to angle EDF. Using a similar method, we can also prove that angle ABC is congruent to angle DEF and angle BCA is congruent to angle EFD. Then by angle-angle similarity, our original triangle ABC is similar to our mid segment triangle DEF.

We now want to prove that the mid-segment triangle is congruent to the medial triangle. The medial triangle, which is shown in green below, is constructed by joining the midpoints of each of the segments of the original triangle.

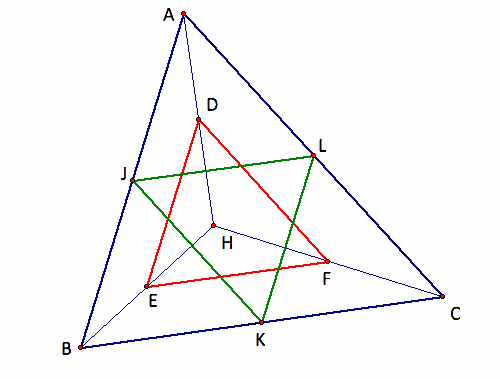
First, we will look at triangle FEH and show that it is similar to triangle CBH using side-angle-side similarity. By construction, segment EH is half the length of segment BH and segment HF is half the length of segment HC. Angle EHF is congruent to angle BHC by the identity property. Then by side-angle-side similarity, triangle EHF is similar to triangle BHF. Since these two triangles are similar, we know that their corresponding sides all have the same ratio. Therefore, segment EF is half the length of segment BC. By the same method, we can show that triangle DHE is similar to triangle AHB and triangle FHD is similar to triangle CHA. Then segment DE is half the length of segment AB and segment FD is half the length of segment CA.

Next, we will discuss the side lengths of the medial triangle. Remember that to construct the medial triangle, we connect the midpoints of each of the sides of the original triangle. Take side JK of the medial triangle. By theorem, segment JK is parallel to segment AC, and JK is half the length of AC. Also by this theorem, segment JL is half the length of segment BC, and segment KL is half the length of segment AB.

To summarize:

Therefore, by side-side-side congruency, the mid-segment triangle DEF is congruent to the medial triangle KLJ.

Lastly, we will look at the centers of the mid-segment triangle. These centers include the centroid, the orthocenter, the circumcenter, and the incenter. How do you think each of these centers will relate to the centers of the original triangle?

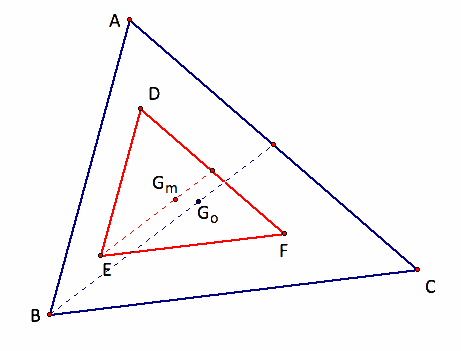
How do you think the centroid of the original triangle will relate to the centroid of the mid-segment triangle? In the construction below, the centroid is labeled with a G for each triangle. The centroid is a point constructed at the intersection of the three medians of a triangle. In the diagram, one set of corresponding medians for each is shown for each triangle. These medians are parallel, so we can hypothesize that for each median in the original triangle, there is a corresponding median that is parallel in the mid-segment triangle. For this triangle, each of the centroids are both inside the mid-segment triangle, but this is not always the case. The centroids are at the same point when we have an equilateral triangle. The medians are the same line when we have an isosceles triangle, so the centroids are on the same line.

What are your predictions for the orthocenter? The two triangles seem to have the same orthocenter at the point H. Let's think about how the orthocenter is constructed. Remember that the orthocenter is the intersection of the three altitudes of a triangle. When we take an altitude of the original triangle, it also forms an altitude of the mid-segment triangle. A vertex is constructed on the line to form the mid-segment triangle, and the line is perpendicular to the opposing side of the mid-segment triangle. Since the two triangles have the same altitudes, they also have the same orthocenter.

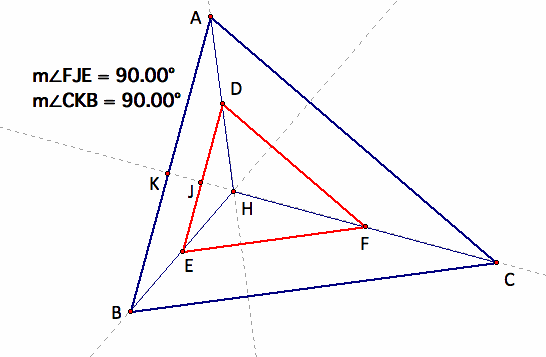
The circumcenter is the intersection of the perpendicular bisector of each side of the triangle and is equidistant from each of the vertices of the triangle. Similar to the medians of each triangle, we can see that a set of corresponding perpendicular bisectors for the two triangles are parallel. Here, the circumcenter for each triangle is inside its corresponding triangle, and they are both inside the mid-segment triangle. If the triangle is changed, we observe that the circumcenter for the mid-segment triangle is in the same position in the mid-segment triangle as the circumcenter in the original triangle is in relation to the original triangle. For example, if the original circumcenter is outside the original triangle, the circumcenter of the mid-segment triangle is outside of the mid-segment triangle.

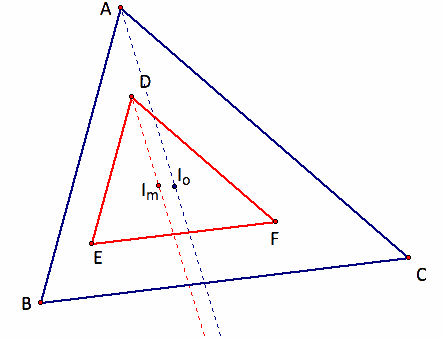
Lastly, how do you predict the incenters of the two triangles will relate? The incenter is constructed by the intersection of the three angle bisectors of each triangle and is equidistant from the three sides of the triangle. Once again, we can see that a set of corresponding angle bisectors for each triangle seem to be parallel like we observed with the medians and perpendicular bisectors of the sides of the triangle. In this triangle, both of the incenters are always inside the mid-segment triangle. Even though this is not always the case, it is true that the incenter will always be inside of its parent triangle.

Return to Kaitlin Spooner's homepage