
EMAT 6680 Write Up # 4a :: Clay Kitchings
:: How To Construct a Specific Triangle Given the Medial Triangle
I recently made an
observation concerning the Medial Triangle and its relationship compared to its
“parent” triangle. So what if the typical question were asked in reverse? Example: “Given the Medial Triangle
DEF, find the three points of the original Triangle ABC.”
I was recently
considering the Triangle Midsegment Theorem (for a proof of said theorem). As I constructed the triangle with GSP,
I decided to hide certain portions of the segments of the triangle. As I began to hide various segments of
Triangle ABC, I came across this picture:

I saw that there
seems to be a parallelogram formed (ADFE). Based on the Triangle Midsegment Theorem, we do indeed have
a parallelogram if D, E, and F are the midpoints of the segments of the
triangle ABC. Then I
thought, “If I have a triangle, I have the ability to construct a
parallelogram. If I can consider the Medial Triangle problem in this light, I
can construct a triangle given the Medial Triangle of that original
triangle.”
Prior Supposition:
The Medial Triangle creates three parallelograms, each whose opposite vertices
are: 1) midpoints of triangle ABC, and 2) a midpoint on a segment and the
vertex opposite that side.
Reference the figure above. Is ADFE a parallelogram? According to the
Triangle Midsegment Theorem, we can conclude that EF = AD and AE = DF. Since the opposite sides of this
quadrilateral are congruent, it is sufficient to argue that ADFE is indeed a
parallelogram.
Let us construct a
triangle ABC given the Medial Triangle DEF of that triangle… shall we?
Let’s start with
triangle DEF, and we’ll assume that these form the Medial Triangle of the
“mystery” triangle ABC. How can we
find the points A, B, and C such that triangle DEF is the Medial Triangle? The Medial Triangle DEF is given below.
(Note the appropriate colors: Red and Black, the color of champions! –
that’s an exclamation point, not meant to be a factorial.)

Let us construct a
parallelogram DFAE. (Note: one could argue similarly for a point A’ which would
create another parallelogram DA’FE “below” segment DF in the figure.) This will
not change the argument here.

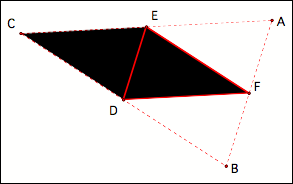
Basically we are
going to construct other parallelograms in order to find points B and C of our
“parent” triangle. The parallelograms (CEFD, EFBD, and DFAE) are illustrated
separately below:


Summary: If you
are given the Medial Triangle, the points of the original triangle may be found
by “completing” parallelograms with that triangle.