Exploring the Pedal Triangle
by
Julie Anne Laycock
What if the pedal point P is the circumcenter (inside and outside of triangle ABC)?
First, lets look at creating the Pedal triangle.
Start by creating triangle ABC. Use lines and not line segments so we won't have to worry about extending the sides of the triangle. Then choose any point in the plane as the pedal point. By constructing perpendicular lines from point P to the sides of the triangle we can then see the pedal triangle by connecting the points that are the intersection of the sides of the triangle and the perpendicular lines.
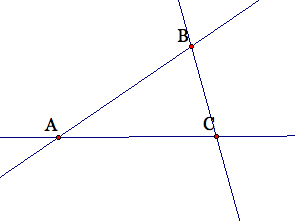Triangle ABC
Pedal Point P and Perpendicular lines
Pedal Triangle
Now lets look at what happens when point P is the circumcenter of triangle ABC. First, lets look at when the circumcenter is inside the triangle.
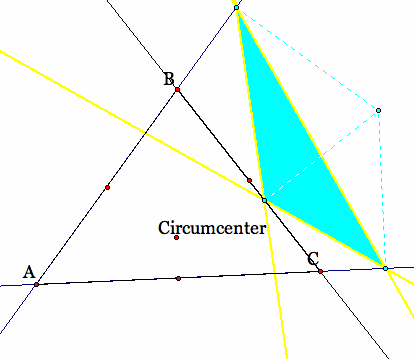
We had to change the triangle a little so the circumcenter will be inside triangle ABC. Remember the circumcenter is the point of con currency of the perpendicular bisectors.
When the pedal point P becomes the circumcenter we can see that the pedal triangle also becomes the medial triangle of triangle ABC. The vertices of the pedal triangle are on the midpoints of the sides of the triangle ABC.
Now lets look at what happens when the circumcenter is outside of triangle ABC and we move the pedal point P to the become the circumcenter.
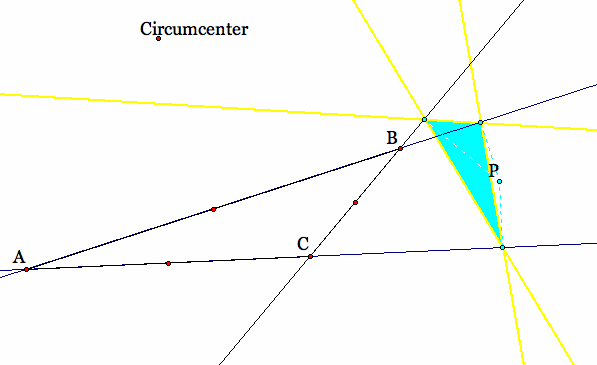
Again, we had to change triangle ABC to move the circumcenter to outside of the triangle.
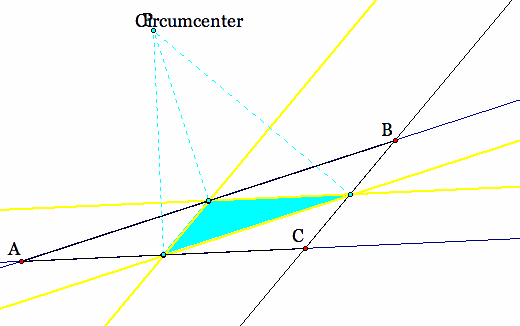
In this case, the circumcenter is outside the triangle ABC. We can see when the pedal point P becomes the circumcenter we can see that the pedal triangle also becomes the medial triangle of triangle ABC. The vertices of the pedal triangle are on the midpoints of the sides of the triangle ABC.