

Assignment 11: Polar Equations
Presented by: Amanda Oudi
Problem: Investigate:
![]()
Discussion:
Let’s use Graphing Calculator to investigate the graph of
the equation.
Begin by exploring the case when a = b and k is an integer.
Example 1:
![]()

The graph produced looks like it has two "petals". Let’s keep a
= b = 1 and focus on changing the k-value.
Example 2:
![]()

Okay, now we see that we have 3 "petals". So, I predict if I change the k-value, then I will have k number of "petals". We can explore that this seems to be the case using Graphing Calculator.
Below are snapshots of when k = 4, 5, 6, and 7:

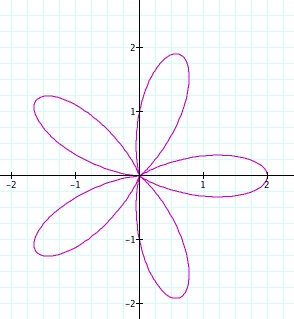

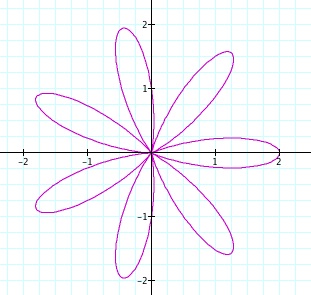
Now, what would happen if the the a and b values were not
equal? I predict that the graphs would be different in shape. Let’s explore
with examples.
Example 3:
![]()
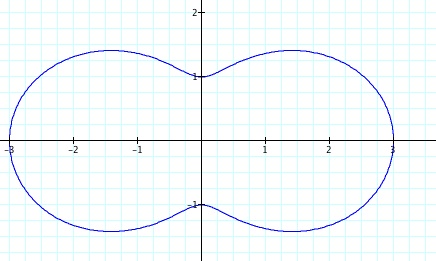
This graph has a somewhat similar shape to Example 1, but
the graph does not pass through the origin. The "petals" are now wider than the ones in previous examples. I think it kind of has a peanut
shape to it!
Example 4:
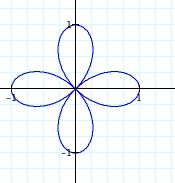
![]()

This graph has 4 "petals" because the k-value in this case =
4. So, we see that altering the a and b values does affect the shape of the "petals"
and whether the graph passes thru the origin. Explore other examples using Graphing Calculator.
Now let's investigate this case:
![]()
Suppose b = 1. Let's see what happens for k = 2, 4, 6.
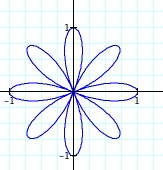

It seems that the number of "petals" are twice the k-value. For instance, for k = 2, there are 4 "petals". For k = 4, there are 8 "petals" and for k = 6, there are 12 "petals". Does the same happen for odd k-values?
Suppose b = 1. Let's see what happens for k = 3, 5,
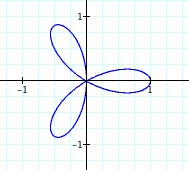

Seems as if for odd k-values, the number of "petals" is the same as the k-value.
What happens if we replace cos() with sin()? Here is a snapshot of r = cos() and r = sin(). It seems that the replacement of cos() with sin() changes orientation.
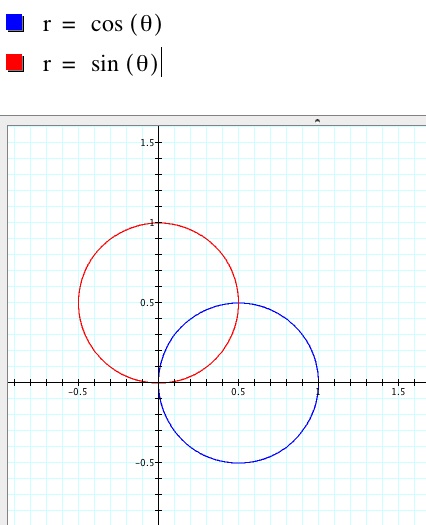
Explore other examples relating to replacing cos() with sin() and make a few conjectures. Why does the orientation change? What do we know about trigonometry that would help us understand why the orientation of these curves is different?
Extensions: What happens if a and b are opposite signs? Can you identify the maximums and minimums in the polar curves explored in this investigation?