

Assignment 4: Triangle Centers
Presented by: Amanda Oudi
There are numerous triangle
centers, but only 4 are focused on in secondary school. The 4 centers that
students study in secondary school are: the centroid, orthocenter, incenter, and circumcenter. Let’s
construct each of these centers and then explore the location of the centers
for various shapes of triangles.
Centroid:
The centroid (G) of a
triangle is the common intersection of the medians. The median of a triangle is
a segment from a vertex to the midpoint of the opposite side. In fact, the
centroid is the balance point/center of mass of a triangle.

Open the GSP animation of the centroidof a triangle. Notice how that as the triangle shape varies, the
centroid remains inside the triangle. This should make sense because how could
the center of gravity of a triangle lie somewhere outside the triangle!
Hands on activity: Students
can easily pinpoint the centroid using by cutting out a triangle using stock
paper and trying to balance a pen or pencil on that triangle.
Orthocenter:
The orthocenter (H) of a
triangle is the point of concurrency of the three lines containing the
altitudes. An altitude is a perpendicular segment from a vertex to the line of
the opposite side.
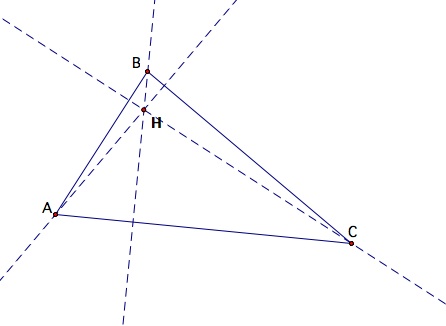
Open the GSP animation of the orthocenter of a triangle. Notice that the orthocenter doesn’t solely lie
inside the triangle like the centroid. Instead, the orthocenter lies inside,
outside, and even on the triangle. When we have an acute triangle, the
orthocenter lies inside the triangle. When we have a right triangle, the
orthocenter lies on a vertex of the right angle. When we have an obtuse
triangle, the orthocenter lies outside the triangle.
Circumcenter:
The circumcenter (C) of a triangle is the point in the plane equidistant from the three vertices of the triangle. Since a point equidistant from two points lies on the perpendicular bisector a segment, C is on the intersection of the perpendicular bisector of each side of the triangle.

Open the GSP animation of the circumcenter of a triangle. We see that the
center does not always lie inside the triangle. In an acute triangle, the circumcenter lies inside the triangle. In a right triangle,
the circumcenter lies exactly at the midpoint of the
hypotenuse. In an obtuse triangle, the circumcenter lies outside the triangle.
Incenter:
The incenter (I) of a triangle is the common intersection of the angle bisectors.
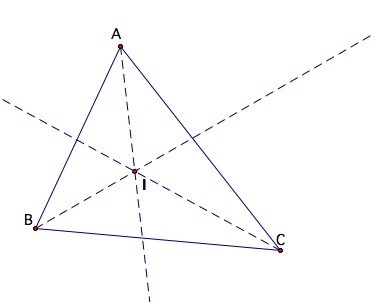
Open the GSP animation of the incenter of a triangle. Like the centroid, the incenter always remains inside the triangle. Notice what happens as the incenter is traced. The trace of the incenter certainly does not form ellipse, but rather a shape that is close to that. Why is this the case?
Another investigation:
Now, let's construct G, H, I, and C for the same triangle. To explore the triangle with all centers, open this GSP file.
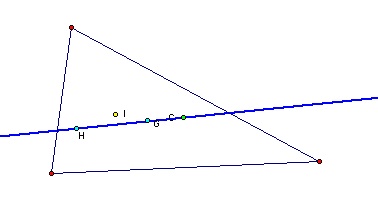
The snapshot above shows a triangle in which the centers G, H, and C all lie on the same line. Using the GSP file, we can explore many shapes of triangles. For an isoceles triangle, centers G, H, and C all lie within the triangle and on the same line, while I lies within the triangle but not on the line. For an obtuse triangle, centers G, H, and C all lie on the same line, but this time, centers H and C lie outside the triangle. This should make sense because we found that the location of centers H and C vary with triangle shape. Again, the incenter does not lie on the line, but does lie within the triangle. For a right triangle, centers G, H, C all lie within the triangle and on the same line. For an equilateral triangle, all four centers, G, H, C, and I, all lie within the triangle and on the same line. The line in which the centers G, H, C form is known as the Euler Line.