Presented by

Dana TeCroney

The As, Bs, and Cs of Quadratics

The purpose of this investigation is to explore what happens as the values of the coefficient ‘b’ varies in a quadratic equation.

Consider the following:

y = ax² +bx + c

If we let a = 1 and c = 1, what would the nature of this equation be? Consider the roots, what would they be (and how many)?

To explore this, consider the equation

0 = x² + bx + 1

In the xy plane the value of b will be one factor that determines the number of roots this equation has. For an example press HERE.

An interesting way to look at this would be in the xb plane.

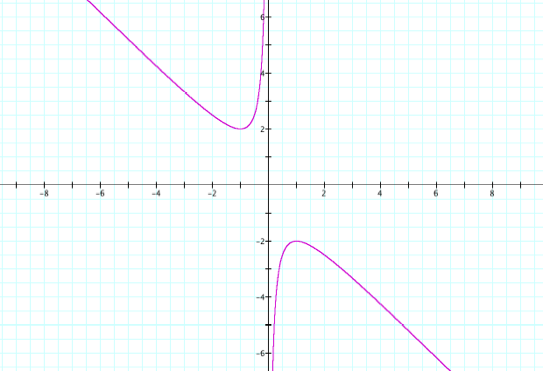
Another way of thinking about the number of roots is to draw in some horizontal lines corresponding to different b values:

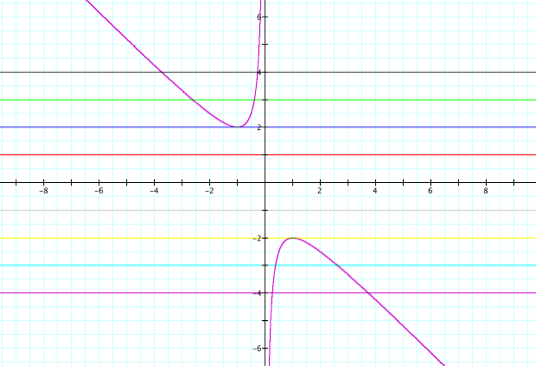
As you can see by the graph, when b > 2 or when b < -2 there are two roots to the equation since the line intersect the curve twice. When –2 < b < 2 there are no real roots since there are no intersection points. This implies that at b = -2, or b = 2 there is one intersection, or one root. Can we see this another way?

Given the equation x² + bx +1 = 0, the roots can be found by

When will this have one root? When the discriminate equals zero of course, ie. when b = -2 or when b = 2.

Another interesting problem would be to change the value of a or c, would this change the nature of the answers? First let’s look at a = -1. So, -x² + bx + 1 = 0, or . In the xb plane, this would look like:

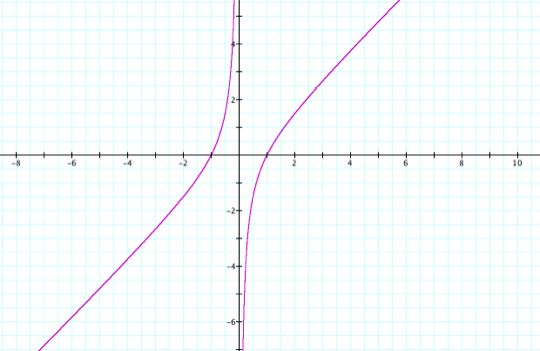
So, if any horizontal line were to be drawn in what would happen, how many intersections would there be? The answer of course is two, and so there will always be two roots. To confirm this you could also look at the discriminate in the quadratic equation:

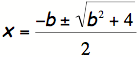
The discriminate of which will never be zero (for real b).

Now let’s consider changing ‘c’. Let a = 1 again and let c = -1.

x² + bx - 1 = 0, or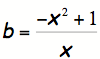

In the xb plane, this would look like:

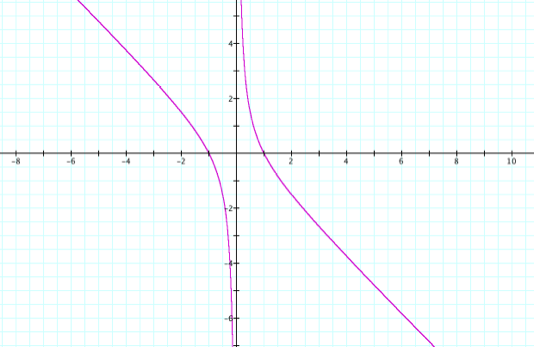
Again by the same arguments as above this equation will have two roots for c = -1, and in fact for c < 0.