

A pedal triangle is constructed as follows:
Let triangle ABC be any triangle. Let P be any point in the plane. Construct perpendiculars to the sides of ABC (extended if necessary). Locate three points R, S, and T that are the intersections. Triangle RST is the Pedal Triangle for Pedal Point P.
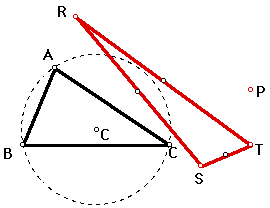
Above, D RST is the pedal triangle of D ABC when P is the pedal point. The dashed circle is the circumcircle of D ABC with its center at C (NOT the vertex C). Click on the picture to manipulate the location of the pedal point P. Make the following observations:
1. If the pedal point is outside D ABC and in the interior of an angle (if you imagine that the sides which form the angle at a vertex are rays extending away from the vertex) of D ABC, then at least one vertex of the pedal triangle D RST lies on a side of D ABC. Specifically, the side is the third side (not used to form the angle).
2. If the pedal point is inside the D ABC, then the vertices of the pedal triangle lie on the sides of D ABC. That is, the pedal triangle is inside D ABC with its vertices on the sides of D ABC.
3. If the pedal point is the circumcenter of D ABC, then the vertices of the pedal triangle are the midpoints of the sides of D ABC.
4. If the pedal point is on a side of D ABC, then the pedal point is in fact one of the the vertices of the pedal triangle.
5. If the pedal point is one of the vertices of D ABC, then the pedal triangle has no area. That is, the vertices R, S, T of of the pedal triangle are collinear. In other words, the pedal triangle is a segment.
6. If the pedal point lies on the circumcircle of D ABC, then the pedal triangle is again a segment since its vertices are collinear. Push the ANIMATE button to observe the pedal triangles whose pedal points lie on the circumcircle.
In 5 and 6, the pedal triangle is a segment. This special segment is called the Simson Line.
If the D ABC is acute or right, then notice that there are times when the Simson Line is actually one of the sides of D ABC. This occurs when the pedal point is the intersection of the circumcircle and the ray which begins at a vertex and passes through the circumcenter.
That is, let ray AC intersect the circumcircle at X, ray BC intersect the circumcircle at Y, and ray CC intersect the circumcircle at Z. Then if X, Y, and Z are pedal points, each of their respective Simson lines (degenerate pedal triangles) would coincide with the side opposite the vertex of that point's respective ray.
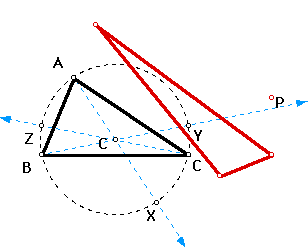
However, if the triangle is obtuse with the above conditions, then the 3 Simson lines still coincide with the sides of D ABC. However, only one of the Simson lines is the same length as its respective side. Click on the picture and make the triangle obtuse.
For which pedal point (X, Y, or Z) is the Simson line a side?
The point which lies in the interior of the obtuse angle. That is:
if < A is obtuse, then the Simson triangle for pedal point X will be side BC.
if < B is obtuse, then the Simson triangle for pedal point X will be side AC.
if < C is obtuse, then the Simson triangle for pedal point X will be side AB.