In the previous unit, we determined that if we could change a standard form quadratic function into vertex form, we could answer any number of questions regarding the key features of a parabola. So it seems worthwhile to try to find a routine to convert a quadratic from standard to vertex form. This process is called completing the square and it is valuable in other areas of mathematics as well. Refer back to the work we did in Unit 6 to change a quadratic function from vertex form to standard form. We will try to simply reverse this process through a step by step approach.
We will start with the same function that we worked with in unit 6
Write a description in your own words of each of the steps below that is left out.
Practice this process of completing the square with the following function (note: if function notation is used, it might be easier to replace f(x) with y.). Each subsequent problem is a little more challenging, so try them all.
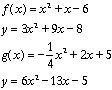
Now we will use this process to convert a general standard form quadratic function into vertex form. This will lead us to the quadratic formula and to a nice shortcut to finding the vertex of a parabola so that we do not have to invest so much time in the completing the square process.
Convert the general form
quadratic equation 
into vertex form
using the completing the square method. Derivation
As a result of this rather lengthy process, we have derived a formula that, given any quadratic equation in standard form, will enable us to find the value(s) of x when the function value is 0. In other word, we can find the x-intercepts (roots/zeros) if we know the coefficients a,b, and c of a quadratic equation in standard form.
Practice using the quadratic
formula on the four functions above. Graph each of the functions
on your calculator and make some notes on your observation. Do
all of these functions have x-intercepts? How can we tell using
the formula when we will have x-intercepts. Is there something
that tips us off as to whether we will have one or two roots (check
out the function 
for example)?
Now we have a relatively straight forward way to find x-intercepts and solve quadratic equations in standard form. In the next units we will learn some shortcuts to help us quickly locate the vertex of a parabola given a standard form quadratic function.