The Problem

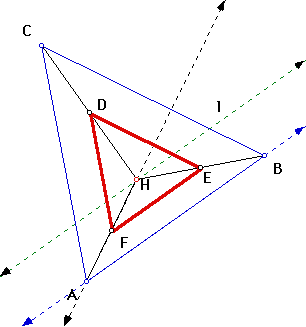
Take an acute triangle ABC. Construct H and the segments HA, HB, and HC. Construct the midpoints of HA, HB, and HC. Connect the midpoints to form a triangle. Prove that this triangle is similar to triangle ABC and congruent to the medial triangle. Construct G, H, C, and I for this triangle. Compare.

(Note: throughout write-up below, click on images to download original file to explore on your own.)

Triangle ABC with segments HA, HB, and HC and the triangle formed by it's midpoints, triangle FED, is as appears below.

To prove triangle ABC is similar to triangle FED, I will show that two pairs of angles are congruent. Two triangles with two pairs of congruent angles are similar.

Begin by constructing line "l" parallel to line BA and through point H. Since line HA is a transversal, angle HAB is congruent to angle HFE. With a similar construction of a line "m", it is evident angle HAC is congruent to angle HFD. Thus, since angle CAB is congruent to angle HAC + angle HAB and angle DFE is congruent to angle HFD + angle HFE, it must be that angle CAB is congruent to angle DFE.

A similar argument will show that angle CBA is congruent to angle DEF. When two triangles have two pairs of congruent angles, they are similar. Therefore triangle ABC is similar to triangle FED.

Next I sketched the medial triangle of ABC. It is labeled triangle LMN below.

To prove triangle FED is congruent to triangle LMN, I will show first that all angles are congruent. Then I will establish the corresponding sides are equal in length. Two triangles with congruent angles and one side length congruent are congruent.

1.	Since LMN is the medial triangle to ABC, the two triangles are similar. Since FED is similar to ABC as well, it must be that FED is similar to LMN. Similar triangles have congruent angles.
2.	Recall from Part 1, angle HFE is congruent to angle HAB. Additionally, angle HEF is congruent to angle HBA. This is enough to conclude triangle HFE is similar to triangle HAB. FH = (1/2)*AH since F is the midpoint of AH. It follows that FE must be (1/2)*AB since triangle HFE is similar to triangle HAB. Next, since triangle FED is similar to triangle ABC and FE = (1/2)*AB, each side of triangle FED is half the length of the corresponding side of triangle ABC. Recall that triangle LMN is the medial triangle for triangle ABC. Thus, the sides of triangle LMN are half the length of corresponding sides of triangle ABC. Since corresponding sides of triangle LMN and corresponding sides of triangle FED are both half the length of the corresponding sides of the same triangle, their corresponding sides must be equal in length.
3.	Two triangles with congruent angles and one side length congruent are congruent. Thus triangle FED incongruent to triangle LMN.

The picture below shows the Euler line color-coded to match each triangle. Note that the Euler Line for triangle ABC is hidden under the other two. Additionally, I've changed the fonts to keep all triangles labeled equivalently. The original triangle ABC and it's Euler Line are labeled with underlines. The medial triangle LMN and it's Euler Line are labeled with outlined letters. And the triangle formed at midpoints to H and it's Euler Line are labeled with italics.

Some observations:

• all three Euler Lines are collinear
• orthocenters of the original triangle and the midpoint triangle are in the same location
• the Euler Lines of the midpoint triangle and of the medial triangle are the same length, and each exactly half the length of the original triangles Euler Line
• the Euler Line of the midpoint triangle is a 180 degree rotation of the Euler Line of the medial triangle about the circumcenter of the medial triangle

PROOF OF THE ABOVE IS LEFT TO YOUR FANCY!

Comments? Questions? e-mail me at blawler@coe.uga.edu

Last revised: December 28, 2000