---

---

Assignment #10

The following write-up will focus on variations of the graph of the parametric equations x=cos(t), y=sin(t) for . Click here to see the graph of this function, which is the unit circle.

Variations of the function provide interesting results. A natural follow-up is to examine the equation x=a cos(t), y=b sin(t) for for variable a and b.

If a=b, the graph is a circle with radius a. For an example when a and b vary from -10 to 10, click here for a Graphing Calculator graph.

By the nature of the cosine and sine curves, we know the graphs will have an x-intercept at a and -a and y-intercept at b and -b. When cos (t) is zero, sin(t) is one , and vice versa.

When a=1 and b=k, constant k, the graph is an ellipse with x-intercept at 1 and -1 and y-intercept at k and -k. Click here to see a graph when k=5.

Similar results follow from a=k and b=1 (except, of course, the x-intercept is 1 and -1 and the y-intercept is k and -k.) Click here to see a graph when k=5 in this case.

Another consideration is to look at c and d when x=cos(ct) and y=sin(dt). When c=d, we get the unit circle. Click here to see when c=d=10. (Note when c and d get large, Graphing Calculator has some error in the graph. It appears the circle "fills in.")

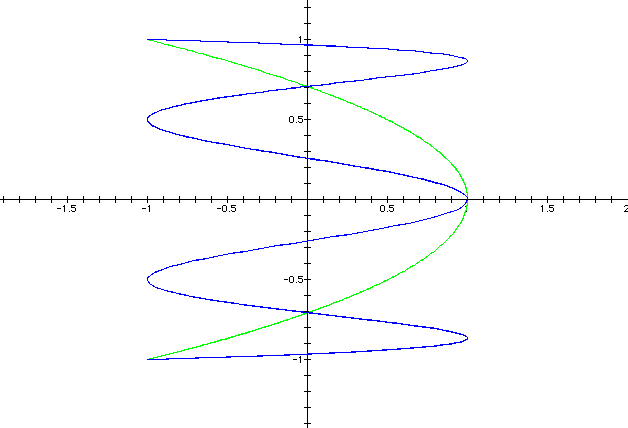
First, let's hold c=1 and vary d among integers. Again, d= - d. See the graphs below of d=2 (green) and d=6 (blue).

Note that each graph intersects itself d-1 times.

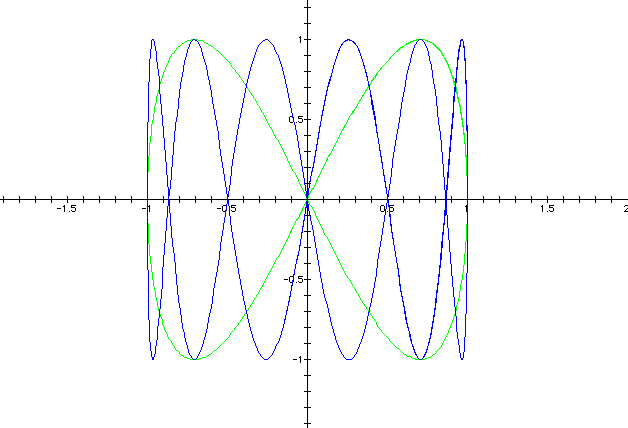
When d=1 and c is different, the graph is as follows(again, c=2 is green and c=6 is blue:)