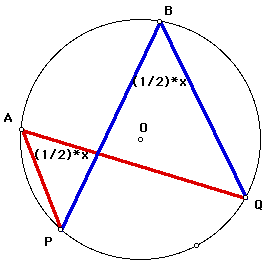
Here, I am given a circle with center O. Angles PAQ and PBQ are inscribed angles of the circle. Both angles intercept the common arc PQ. I want to prove that the measure of angle PAQ = the measure of angle PBQ.

First, I construct segments OP and PQ.
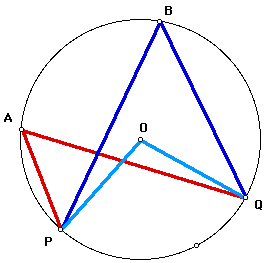
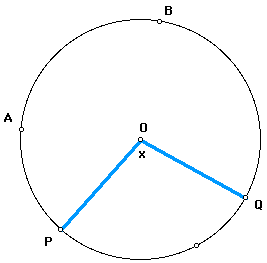
This construction creates the central angle POQ. I'll let the measure of angle POQ = x.
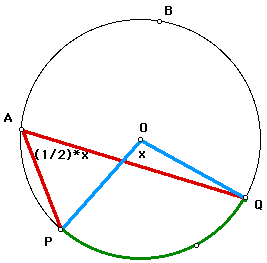
Since central angle POQ and inscribed angle PAQ intercept the common arc PQ, then the measure of angle PAQ = 1/2 of the measure of angle POQ. By substitution, the measure of angle PAQ = (1/2)*x.
Similaryly, since central angle POQ and inscribed angle PBQ intercept the common arc PQ, then the measure of angle PBQ = 1/2 of the measure of angle POQ. By substitution, the measure of angle PBQ = (1/2)*x.
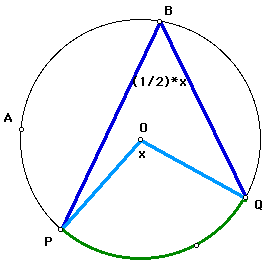
In summary, the measure of angle PAQ = (1/2)*x, and the measure of angle PBQ = 1/2*x. By the transitive property, the measure of angle PAQ = the measure of angle PBQ, which was to be proved.