

This investigation involves constructing a circle that is tangent to two given circles.
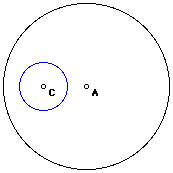
To construct such a circle, we need to choose a point on circle A, point E, then construct a line through the two points A and E. We also need to choose a point on circle C construct the line segment that is the radius of the circle C. We then need to construct a circle with center point B with the same radius as circle C.
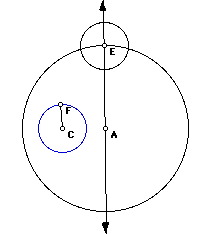
The next step is to choose the outside intersection point of circle E and line AE. Then we need to construct the line segment joining this point and point C. At this point, we need to construct the perpendicular bisector of this segment. Where the perpendicular bisector crosses line AE will be the center of the tangent circle.
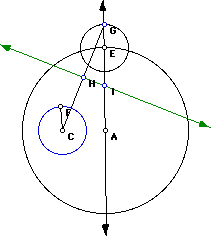
To construct the tangent circle we choose point I and construct a circle with center I and radius IE.
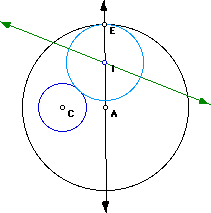
This circle I is the tangent circle to both circle A and circle C.
Now we will trace the path of the center of the tangent circle I, by dragging point E around the big circle A.
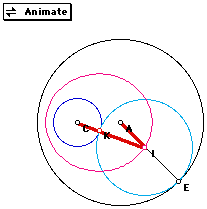
Notice that by animating the point E around the large circle with center A (which is not seen in the picture), and tracing the center of the tangent circle, we can see that the path of the center of the tangent circle appears to be an elipse. Click here to see proof that the path is an elipse. To see this animation, click here, then double click on the animate button. Note, you must have Geometers Sketch Pad in order to see this animation. Notice that the path is an elipse with foci, A and C.
What happens if circle C is outside of circle A?
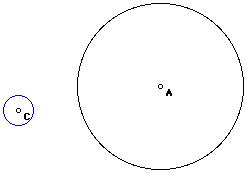
We will construct the circle that is tangent to both circles using the same method as above.

Will the center of the tangent circle follow the path of an elipse as point E is traced around circle A?
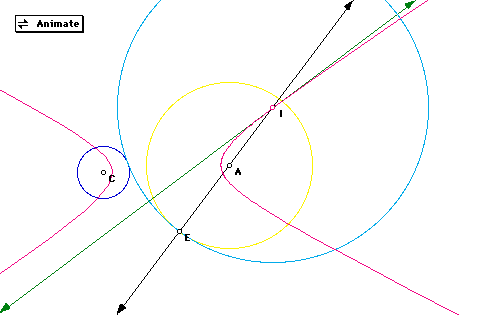
Notice that the path of the center of the tangent circle is no longer an elipse, but the path is a hyperbola with foci A and C. To see proof that the path of point I is an elipse, click here. To see this animation click here then double click on animate. Again, you must have Geometers Sketch Pad in order to access this file.