Write-up #8
Exploring Altitudes in Relation to Circumcircles
by
Holly Anthony
Problem: Construct any acute triangle ABC and its circumcircle. Construct the three altitudes AD, BE, and CF. Extend each altitude to its intersection with the circumcircle at corresponding points P,Q, and R. Then find:
![]()
and prove your result.
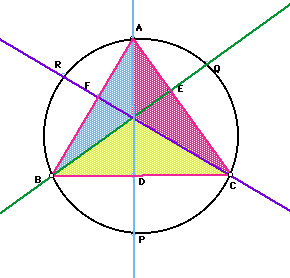
First, let's look at our construction:

To manipulate this graph for yourself with measures and ratios of
![]()
given, open GSP and click here.
You should discover that ![]() = 4
= 4
We find that the sum of these ratios always equals four regardless of your manipulation of the graph. However, the measurements in GSP are not enough to prove this.
For a proof, see below.
Proof
Prove: ![]() = 4
= 4
For the proof, refer to the illustration below:
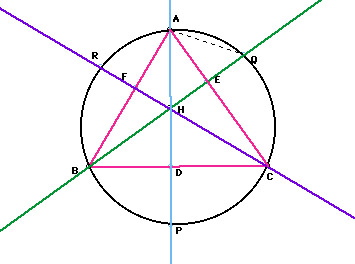
First, consider the two triangles: AHE and AQE.
Angles AHQ and AQH are congruent since they both subtend the same chord on the circumcircle.
AEH and AEQ are both 90 degrees since BE is an altitude and they share the side AE. This shows that they are congruent, thus HE = EQ and similarly HF = FR and HD = DP.
So we have the following:
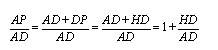
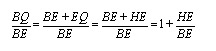
![]()
Now we have:
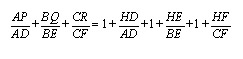
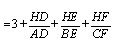
Now let's consider:

First look at the triangle again.
We see that AD*BC is twice the area of ABC since AD is the height for the base BC.
CF*AB and BE*AC are also twice the area of ABC. We will call this number X.
Now notice that the area of ABC could be computed by summing the areas of AHC, BHC, and AHB.
Then twice the area of AHC is HE*AC, the area of BHC is HD*BC, and the area of BHA is HF*AB.
So now consider again:
![]()
Notice the values of the denominators on the right-hand side of the above equation. The are all two times the area of ABC or X. We can factor this out:
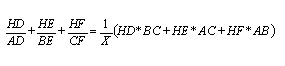
Remember that:
![]()
so we have:
![]()
Therefore,
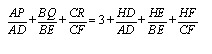
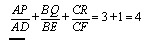
End of Proof.