

Today we will be exploring tangent circles. We first want to look at the picture of the tangent circles we have constructed. We first start with a large circle and then a smaller circle inside of it which look like this:
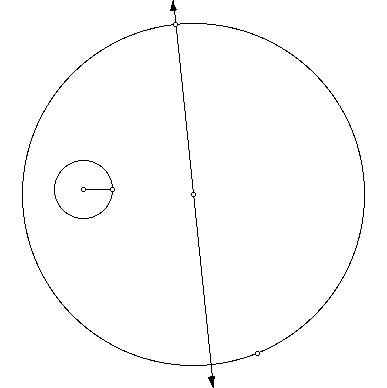
Now we want to use the radius of the smaller circle to find a triangles so with the lines through the center of the large circle and the smaller circle we can start to find it like so:
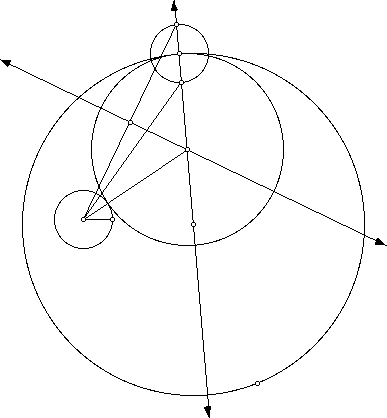
We are able to see that the smaller circle is tangent to the middle circle and also the middle circle is also tangent to the circle we first started with. So we now want to see what it will look like as it moves around. We can now see our triangle in blue and the circle tangent to the large and smaller circles will be in red below:

The middle circle(red) stays tangent to the other two as we keep moving around the circle as shown below:
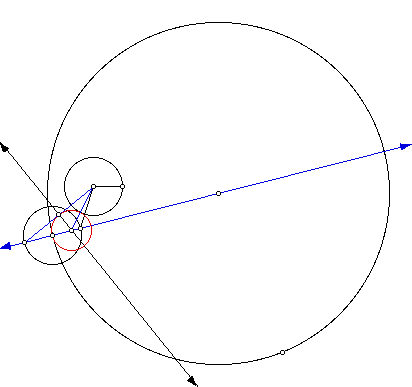
Well we now want to animate the path of the middle circle inside of the larger circle and trace the locus of the center. This will give us the image below when we trace the center:

We can see from the picture above that when we trace the center of the middle circle it looks like we get an ellipse. It also looks like the center of the large black circle and the center of the smaller circle are the two foci because when we constructed the circle we constructed it by connecting two segments to the two given circles (blue and black), so it is the sum of the segments are the same as the sum of the radius from both circles.
We can make a script for tangent circles and if you would like to try one just click here.
If you would like to see an animation of the ellipse as the middle circle moves around the large one and the small one just click here.
We want to explore if the smaller circle is internal to the tangent circle what we will get. As it moves outside it looks like we will get a parabola of some kind, but the picture with the tangent circle internal to the tangent circle is like the one below:

Now we want to see if it also forms two parabolas with the center of each circle seen as the two points in each parabola. So we can observe the following picture:
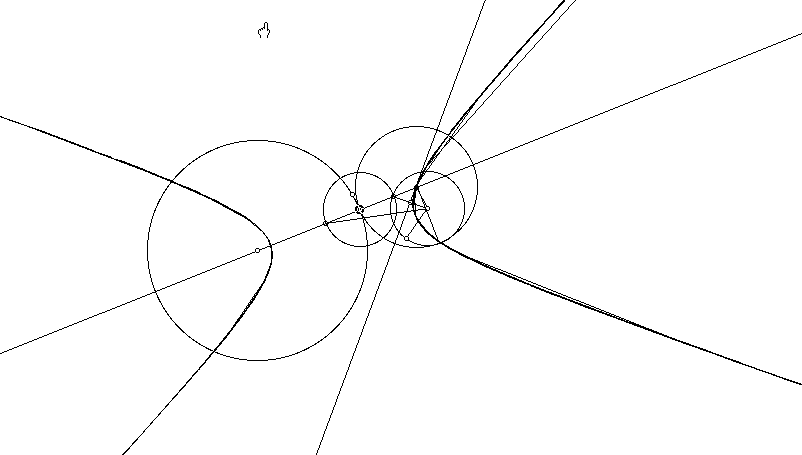
We want to change the point of view and we can still see that we will get a parabola formed above and below each of the radii of the larger circle and the smaller circle:

We see that we get two parabolas going in the oppositedirection from each other and the smaller circle is internal to the tangent circle.
We now want to explore when the smaller circle is external to the tangent circle we get the picture below. What do you think we will get? An ellipse? Two Parabolas? Well lets see.
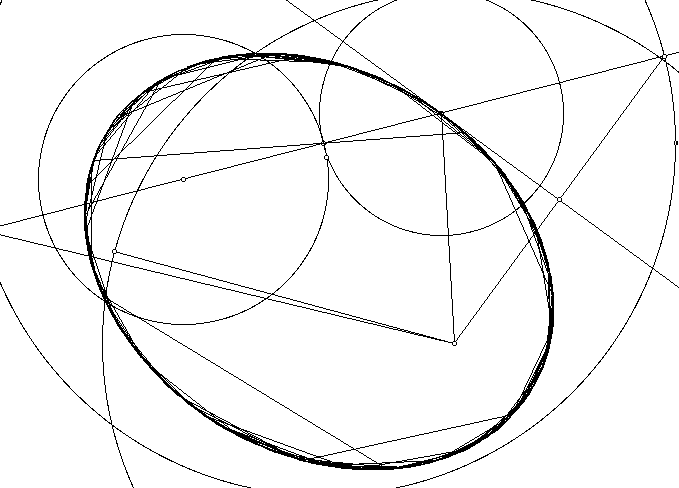
We can see that it will also give us an ellipse, but just a larger ellipse because the smaller cirlce is external to the tangent circle. This concludes our investigation on tangent circles, but we will learn more GSP as we study further in the semester.