Assignment #7
Construction of Two
Circles Both Tangent to a Given Circle and a Line
By: Elizabeth Gore
To begin this investigation we will
start out with a construction sequence yeilding two circles both
tangent to a given circle and a line.
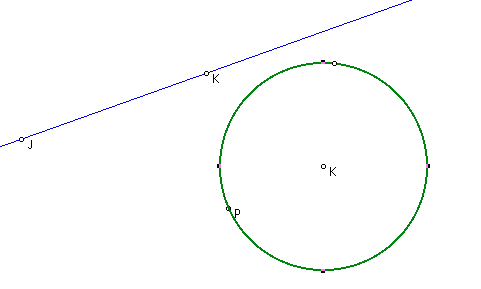
In this first sketch,
I have simply constructed an arbitrary circle with K as the center
and an arbitrary line, which I will make each of the two new circles
tangent to.
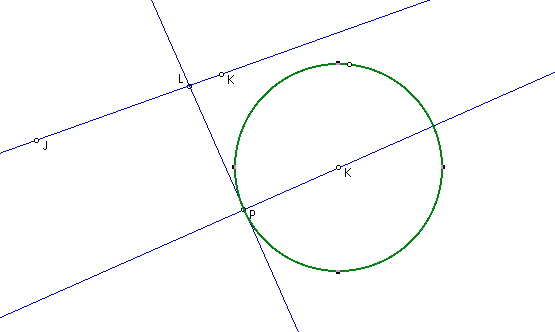
The next step in construction is to
form a line that will connect the circle and the line that the
circles are to be tangent to.
If two new circles are to be tangent
to the given circle at point P,
then the two new circles must be tangent
to the line that is tangent to the given circle at point P.
To so this, I first constructed a line
through the center of the circle K and the arbitrary point P.
I know this line will be perpendicular
to the tangent line at P.
I have also marked the intersection
of the tangent line and the given line at L.
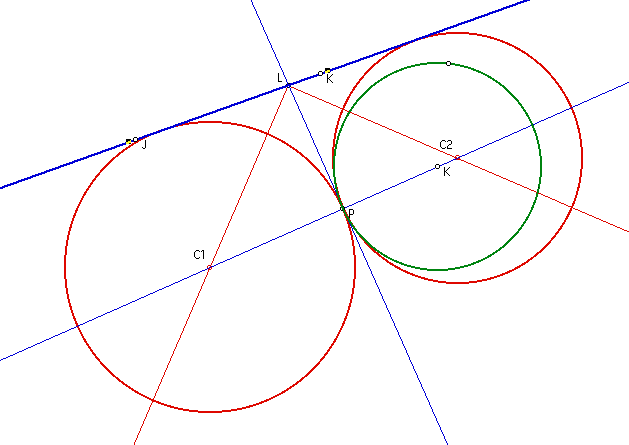
Since each of the two new circles must
be tangent to line PL at point L,
then it follows that their centers
must lie on line KP (since the radius of each circle must be perpendicular
to line PL at point P).
So where on line KP must these centers
lie?
Since the new circles must be tangent
to the given line, there also must be a radius that is perpendicular
to that line.
Therefore, the center of each circle
must be equidistant from the given line and from point P.
Here I have constructed a pair of angle
bisectors of angles JLP and KLP, where J and K are simply points
on either side of L that lie on the same line.
Where these bisectors cross line KP
is where the centers(C1 and C2) of my new circles are.
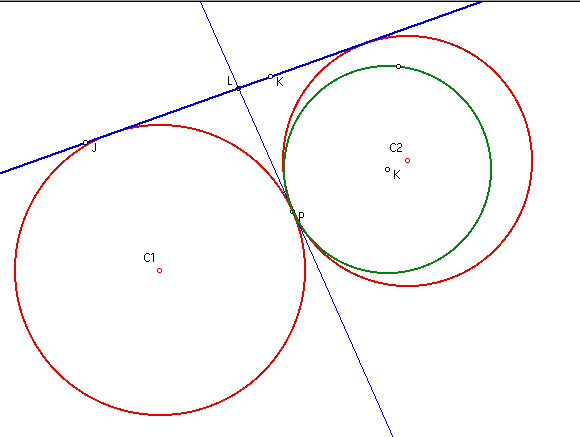
BEHOLD, two circles both tangent to
the given circle K and the given line containing point L.
Would you like a sketch to manipulate
for yourself? If so click HERE.
If you would care to see the proof
that C1 and C2 are really the centers of my two new circles click
HERE.
Return
to my HomePage