2-3: Congruent Segments
In our last example we constructed a six-sided object
and a six-pointed star by using Geometry Sketchpad. In that construction
we used the midpoint construction tool, in this example let's
see how we can use Geometry Sketchpad to find the midpoint of
a segment with using the construction tool.
Step by Step Instructions
a. We first must construct a line segment

b. Now if we had a compass we would construct arcs as described
in the book so without a compass what do we do? Lets construct
circles where the radius is equal to the line segment. We do this
by having an endpoint of the segment be the center of our circle
and the other endpoint being our point on the circle. Then repeat
swapping the role of the endpoints, thus giving us two circles.
(Highlight point on left of segment then point on right of segment,
Select Circle by Center & Endpoint from "Construction"
menu)
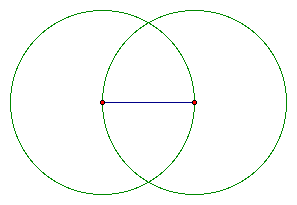
c. Now identify the points where the circles intercept
these would be the same points where the arcs would intercept
if we were using a compass.
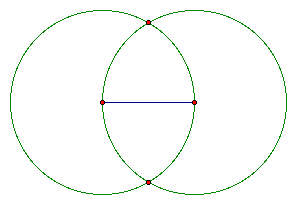
d. Then if we draw a segment between our two points
of interception we can identify where the new line segment intercepts
our original line segmentthis point is considered our midpoint.
 If we were using our compass and straight edge we could
measure the length of the line segment on either side of our midpoint
to verify it is accurate. What can we do with Sketchpad to verify
our construction is accurate?
If we were using our compass and straight edge we could
measure the length of the line segment on either side of our midpoint
to verify it is accurate. What can we do with Sketchpad to verify
our construction is accurate?
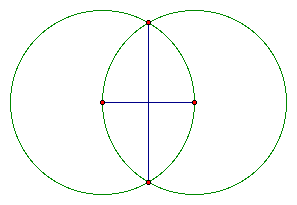
e. This is where we can use our "Measure"
menu. Highlight the left endpoint of our original line segment
then our midpointFrom the "Measure" menu select distance.
 f. Now Highlight the our midpoint and the right endpoint
of our original line segmentFrom the "Measure" menu
select distance.
f. Now Highlight the our midpoint and the right endpoint
of our original line segmentFrom the "Measure" menu
select distance.
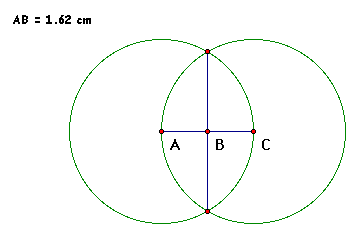
 Here we see that the distance from point A to point
B is equal to the distance from point B to point C thus making
our midpoint accurate.
Here we see that the distance from point A to point
B is equal to the distance from point B to point C thus making
our midpoint accurate.
Using this same technique, given a line segment divide
it into 8 congruent segments.




