The problem:

Given two circles and a point on one of the circles. Construct a circle tangent to the two circles with one point of tangency being the designated point.

Case 1:

The smaller circle is in the interior of the larger circle with the point of tangency on the larger circle.

A. The tangent circle (blue) is in the exterior of the smaller circle.

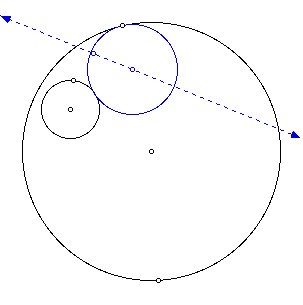
The locus of the center of the tangent circle is an ellipse.

B. The smaller circle is in the interior of the tangent circle (red).

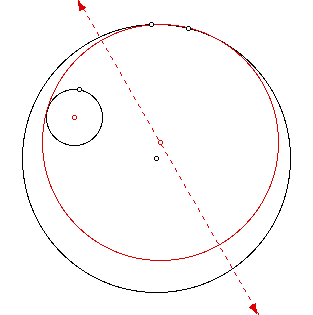
The locus of the center of the tangent circle is an ellipse.

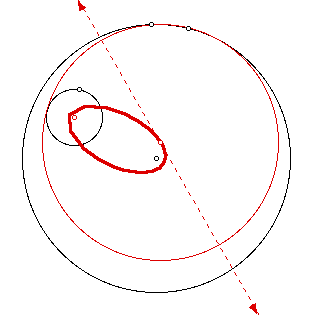
Case 2:

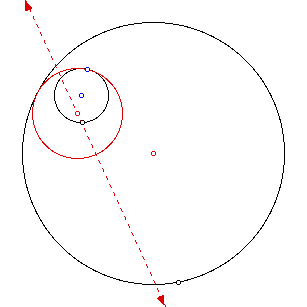
The smaller circle is in the interior of the larger circle with the point of tangency on the smaller circle.

A. The tangent circle (bllue) is in the exterior of the smaller circle.

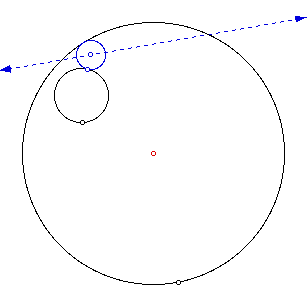
The locus of the center of the tangent circle is an ellipse.

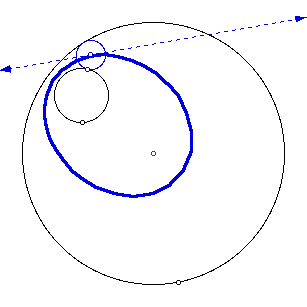
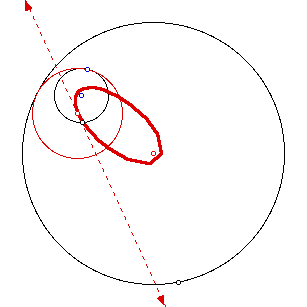
B. The smaller circle is in the interior of the tangent circle (red).

The locus of the center of the tangent circle is an ellipse.

Case 3:

The two given circles intersect.

A. The tangent circle is in the exterior of the smaller circle and the point of tangency is on the larger circle.

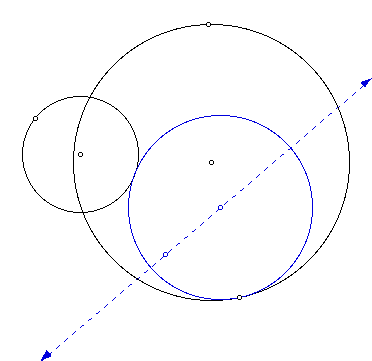
The locus of the tangent circle is an ellipse.

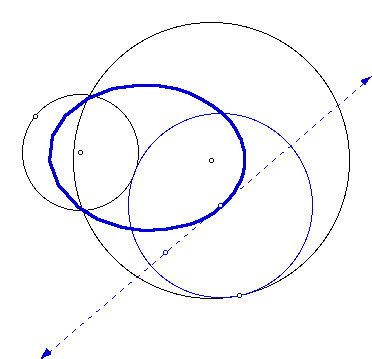
B. The tangent circle is on the interior of both given circles.

The locus of the tangent circle is a hyperbola. Click here to see the image.

C. The tangent circle is in the exterior of both given circles.

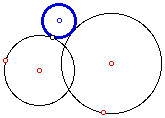
The locus of the tangent circle is a hyperbola. Click here to see the image.

Case 4:

The two given circles are disjoint.

Several tangent circles are drawn in blue.

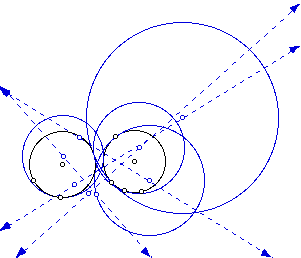
The locus of these tangent circles lie on a hyperbola. Click here to see the locus.

Return to Class Page